Solution: ‘Time Through an LCD Display’
Our September Insights puzzle features a toy model universe inspired by the kinds of serious models discussed by experts in the Time in Cosmology (opens a new tab) conference. This toy universe consisted of a 5-by-6 grid of nodes featuring a seven-segment LCD display of the number 8 at its center. The idea was to explore the concepts of time and entropy mixed in with an idea inspired by Darwinian natural selection, called stratified stability. The rules of the universe are summarized below:
Atoms: The universe is populated by seven red bars, each one unit long, which span two neighboring nodes on the grid horizontally or vertically.
“Central Galaxy” or LCD: The central 3-by-2 portion of this universe can be thought of as the familiar seven-segment liquid crystal display (LCD) from an old-fashioned calculator.
Initial State: The bars are all arranged on the seven segments of the central LCD to form the number “8.”
Dynamic Laws: Every second, all the bars move randomly as follows: One of the two ends of each bar moves to a new position, picked at random from among the neighbors of the node it was on (like a random walk, but constrained by the grid’s border). The other end of the bar follows randomly to one of the neighbors of the new position.
Entropy (Measure of Disorder): The system is considered to be most orderly if all the segments of the central LCD are occupied. The “entropy” of this initial state is zero. Maximum entropy is achieved when no bars touch any of the nodes of the central LCD “8.”
The value of the entropy is calculated as follows. Count the numbers of the following: unoccupied central LCD segments (u); the number of bars fully occupying a segment of the central LCD (there may be more than one bar occupying the same segment) (b); the number of bars touching a node of the central LCD but otherwise outside it (t). Then calculate the entropy using the formula (14 + 4u – 2b – t)/42.
Note that the above definition of “entropy” is not standard. As Russell O’Connor (opens a new tab) pointed out, “Entropy is the logarithm of the number of microstates that satisfies a given macrostate description. Entropy is a measure of one’s knowledge of the state of a system; it is not an intrinsic property of the state itself.” As I explained (opens a new tab), my idea was to come up with a simple measure of disorder that still somewhat faithfully tracked actual entropy while giving due importance to the visual salience of the central LCD area with primacy to the display of the number 8 and its role in stratified stability.
Andy Hall, the founder of TestTubeGames (opens a new tab), created a wonderful visual simulation of this toy universe, as you can see in the screen shot below or play with here (opens a new tab). The simulation allows you to start in a low or a high entropy state. The STICKY OFF button can be toggled to STICKY ON to simulate stratified stability as specified in question 2 below. If you hold down the ADVANCE button, the simulation will run continuously.
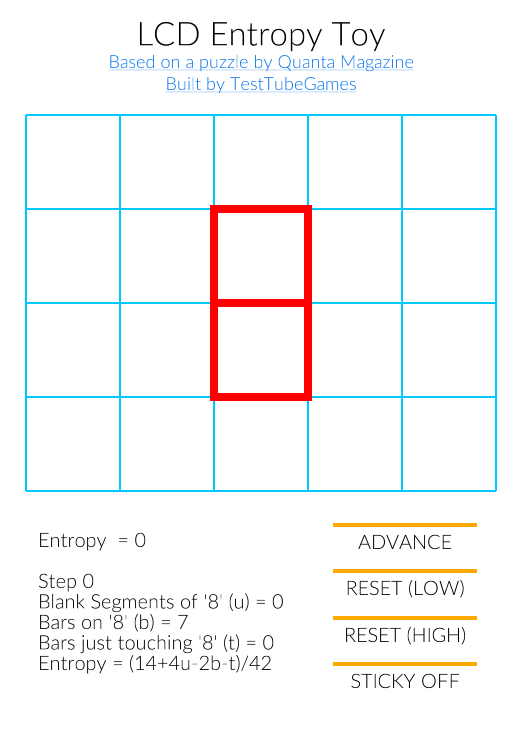
Question 1:
From the initial zero-entropy state, how long do you think it will take to get to the completely “scrambled” or maximum-entropy state? What will be the approximate value of the entropy when the universe reaches a “steady state”? How long will this take? Can it ever get back to the original minimum-entropy or “whole egg” state? If so, how long do you think this scenario will take?
Solution 1:
If you run Andy’s simulation, you can see that the state of maximum disorder where all the red bars are off the central LCD is reached pretty quickly — in around 20 ticks. The value of the entropy in the “steady state,” which is reached extremely quickly, is not flat. Rather, it fluctuates around 0.8, sometimes reaching the maximum value of 1, and sometimes dipping to 0.6 or even lower. The exact average is about 0.83, as determined from a detailed calculation by Jackson Walters (opens a new tab).
There is indeed a finite probability that the universe will be restored to the original “whole egg” state for a fleeting tick, but it is extremely low indeed. How low? As Michael (opens a new tab) noted, there is a possible ambiguity about the distinguishability of the bars in this model: I apologize for not clarifying this right away. The simplest take is to consider the bars to be theoretically distinguishable noninteracting discrete objects, like cards or coins, that we are used to doing probability calculations with. Multiple bars can occupy the same position in the universe, though — presumably each position is wide or deep enough to accommodate up to seven of them. Unlike exotic particles like bosons, however, we can, in our mind’s eye, put distinguishing numbers on the bars, even if these marks are not visible. This makes things simple: You can imagine seven different virtual private universes for each bar labeled one through seven, and then superimpose all of them to come up with the final configuration, with the labels erased. It is a fact that there are 49 equally likely positions for each bar, as we shall see later, so the total number of possible configurations is 497, or 6.8 x 1011. To generate the figure 8, bar number 1 would have to be in one of seven positions in its own private universe, bar number 2 in one of six remaining positions, and so on. So the probability of generating the 8 display will be 7!/497 — it will happen by chance in about 1 in 135 million tries. Since we are starting from the maximum-entropy situation, we need to apply the following correction as specified by Michael G: (opens a new tab) On average, the time is 1/(PERMUT(42,7-b)*PERMUT(7,b)/PERMUT(49,7)), or about 86 million ticks. Andy’s simulation goes through 10 steps in three seconds, so a dedicated Quanta reader who starts from the initial high-entropy state and holds down the “advance” button would have to remain frozen, eagle-eyed, for about three years and nine months on average before catching a glimpse of the 8 in its fleeting glory. Whoops, don’t tell me you missed it!
This tells us two things that are applicable to the real universe. The first is that even in the high-entropy state that we will finally reach — the so-called heat death of the universe — there will be fluctuations where the entropy will decrease just by chance. The second is that it is indeed possible to return from high to low entropy just by chance (the second law of thermodynamics notwithstanding, thanks to both classical and quantum fluctuations), but the time required to do this is many, many orders of magnitude higher than that required for the reverse process. One serious estimate (opens a new tab) of this time, based on quantum fluctuations, is 10^10^10^56 years. This is an unimaginably vast amount of time. But hey, when you have all of eternity to create a universe, you can just say, “Let there be light,” and wait for it to happen!
Our second question can be simulated in Andy’s game by toggling the STICKY OFF button to STICKY ON: It shows the magic that can happen if certain configurations have an inherent stability.
Question 2:
In the “sticky on” condition, how long do you think it will take to reach the minimum-entropy state and form the number 8 on the LCD?
Solution 2:
The answer is, not very long at all! You can see this in the picture below.
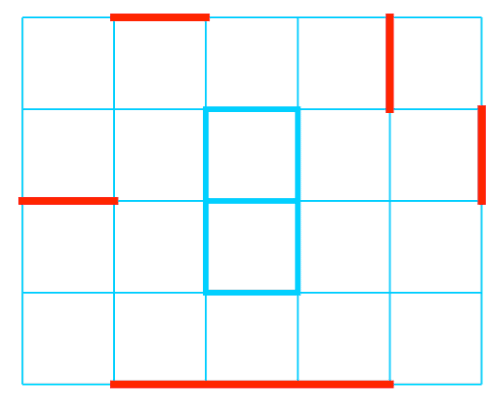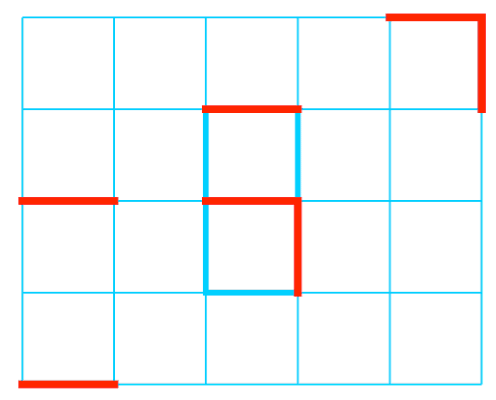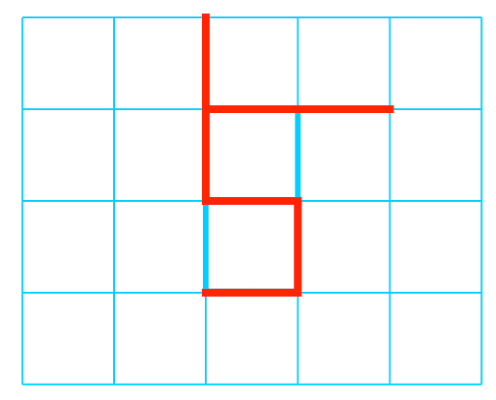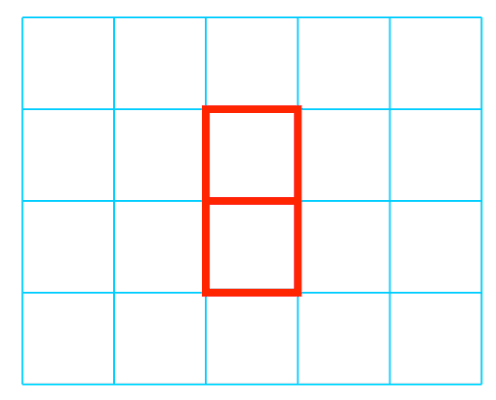
You can go ahead and do several runs to see how long it takes on average. As you will notice, it is easy to get the first bar to enter the central LCD area, but it gets progressively harder as fewer bars remain. The average time, about 125 ticks, can be calculated by adding the expected time for each consecutive bar as Michael (opens a new tab) nicely demonstrated.
As you can see, differential stability can accelerate the formation of an extremely low probability state a millionfold. This kind of speedup, which is achieved by natural selection in the biological realm, can be achieved by the stability conferred on certain structures by physical forces in the physical, chemical and prebiological realms. As I mentioned in the puzzle column, this is Jacob Bronowski’s concept of stratified stability (opens a new tab), which functions in a way analogous to natural selection: Stable structures are “selected” by virtue of their persistence in time and become the basis for increasing complexity and decreasing entropy. Thus, the force of gravity can create stable structures such as stars and galaxies. Our toy model is a simpler version of the more sophisticated model developed by physicist Tim Koslowski (opens a new tab) and his colleagues, which is the basis for a novel view of the arrow of time. As Dan Falk reports in his Quanta article on the Time in Cosmology (opens a new tab) conference:
Koslowski and his colleagues developed simple models of universes made up of 1,000 pointlike particles, subject only to Newton’s law of gravitation, and found that there will always be a moment of maximum density and minimum complexity. As one moves away from that point, in either direction, complexity increases. Naturally, we — complex creatures capable of making observations — can only evolve at some distance from the minimum. Still, wherever we happen to find ourselves in the history of the universe, we can point to an era of less complexity and call it the past, Koslowski said. The models are globally time-symmetric, but every observer will experience a local arrow of time. It’s significant that the low-entropy starting point isn’t an add-on to the model. Rather, it emerges naturally from it. “Gravity essentially eliminates the need for a past hypothesis,” Koslowski said.
To inject some levity into this tale of gravity, I just want to remark in passing (or is it returning?) that in the Hindi and Urdu languages the word for “tomorrow” is the same as the word for “yesterday.”
Question 3:
In the universe of question 2, of the following three shapes that could appear on the LCD — the numbers 6 and 9 and the letter A — which one is most likely to be the last step before the number 8 is reached? Which is least likely?
Solution 3:
Our model universe has a boundary, and when the bars reach it, they eventually have to move back toward the center. This question essentially asks how the boundary affects the likelihood of a bar close to it relative to a bar staying at the center. Does the probability of staying near the border increase, decrease or stay the same? We can determine this by using symmetry and setting up equations for the probabilities among neighboring nodes as we did last time in the puzzle about the drunkard’s walk , or we can use a finite state Markov chain. Neither of these is easy: The first method involves generating and solving a system of 15 equations in 15 variables, while the second involves generating a 49-by-49 matrix. This giant’s labor was performed by Jackson Walters, whose updated model confirms that the border does nothing — the probability of the bar being in any one of the 49 positions in our universe is exactly the same: one in 49. The reason this happens is that the bars are more likely to stick to their positions at the border because they have fewer neighbors. Both the center bars and the corner bars carry out random walks, moving away from their points of origin with time. The greater “self-stickiness” of the corner bars causes them to move more slowly, exactly compensating for the fact that they can only move in constrained directions. There’s probably a simple way to prove this based on the dynamics.
So the answer to the question is that out of 6, 9 and A, all three are equally likely as the last step, as is the number 0.
Question 4:
Can you figure out the rationale behind the entropy calculation?
Solution 4:
Michael G (opens a new tab) nailed this question:
You want the central configuration to be zero “entropy.”
You want all bars totally away from central configuration to be one.
You want all central (but not all central covered) to be >0 but low.
You chose a not-touching bar to have twice the entropy of a touching bar (arbitrary).
14 is just equal to 2b when b=7 and u=0 (so the all-central configuration is zero “entropy”).
42 is just equal to the numerator at maximum “entropy” of b=0,u=7,t=0
yielding 14+4*7=14+28=42 … so the final “entropy” is between 0 and 1.
Bingo!
Thank you to all who contributed. I hope this was a fun and instructive exercise. Special thanks to Andy Hall for creating the simulation. As I mentioned, Andy is the creator of TestTubeGames (opens a new tab), an indie studio devoted to making science games. The games feature a variety of physics topics, letting you run near the speed of light to experience relativity, fling planets to explore the laws of gravity, or electrically charge yourself to learn about electromagnetic forces. Seems like just the kind of thing Quanta readers might enjoy.
The Quanta T-shirt for this month goes to Andy Hall for his splendid simulation. Congratulations!
See you next month for new insights.



