A Child’s Puzzle Has Helped Unlock the Secrets of Magnetism
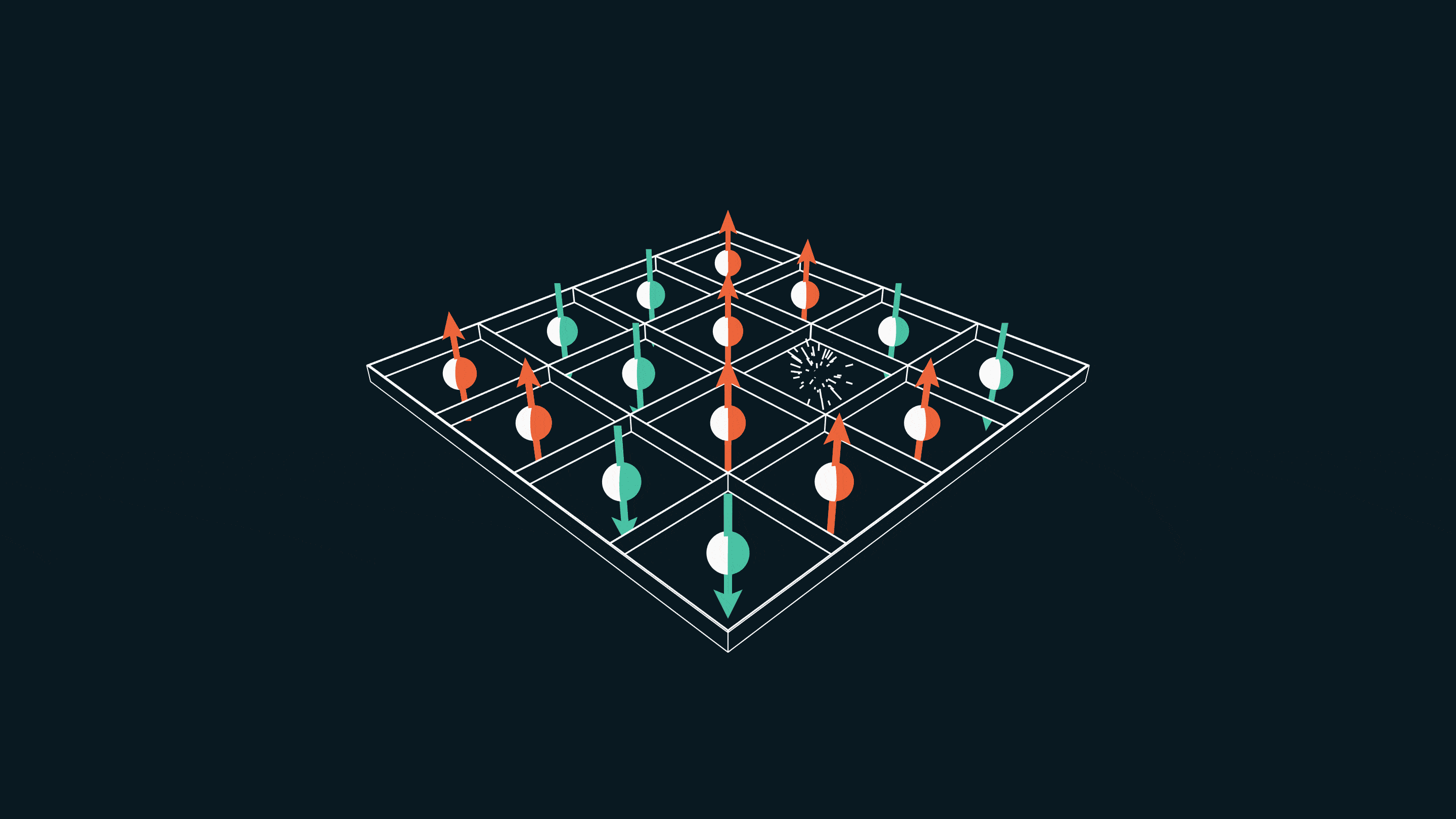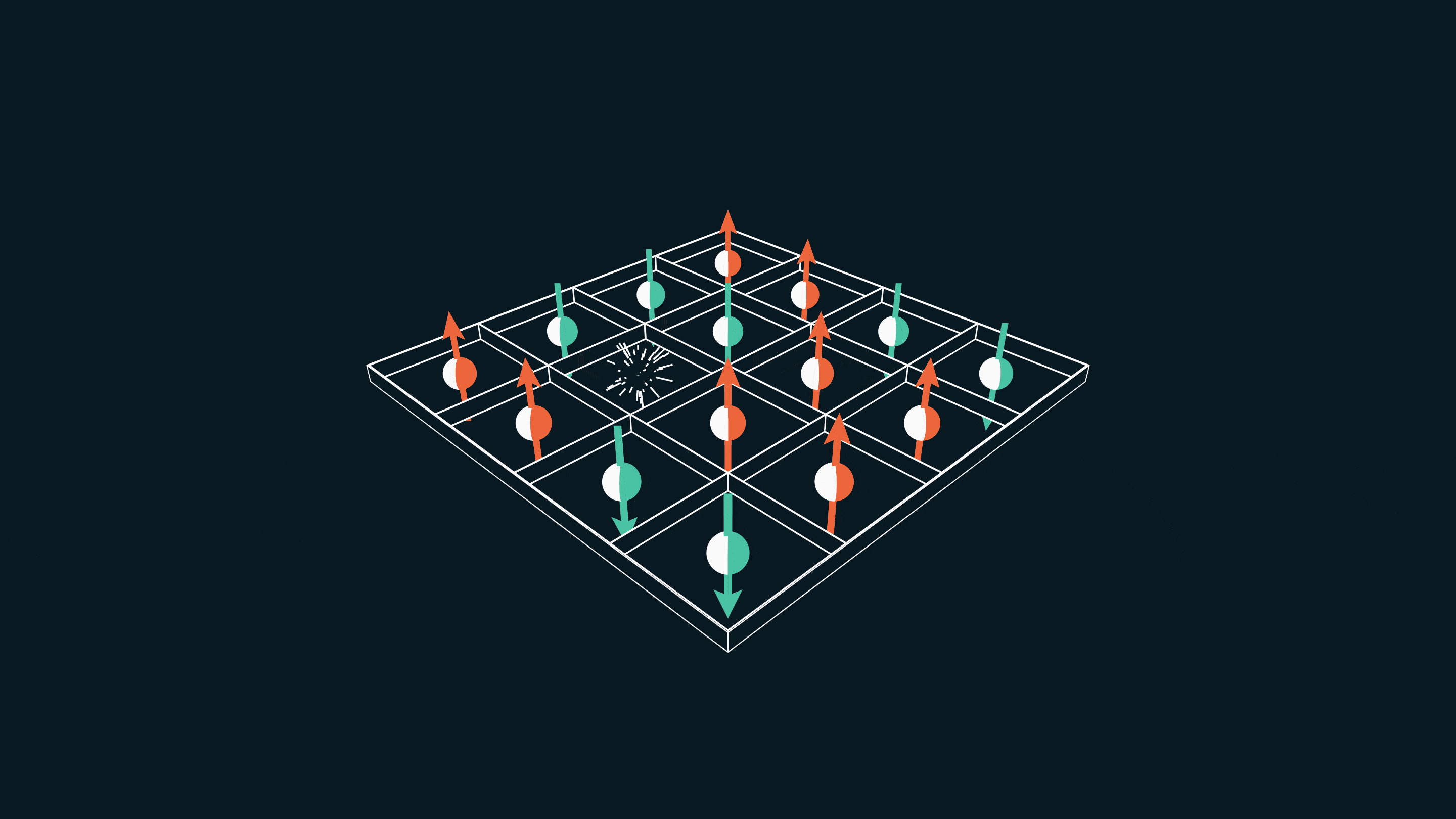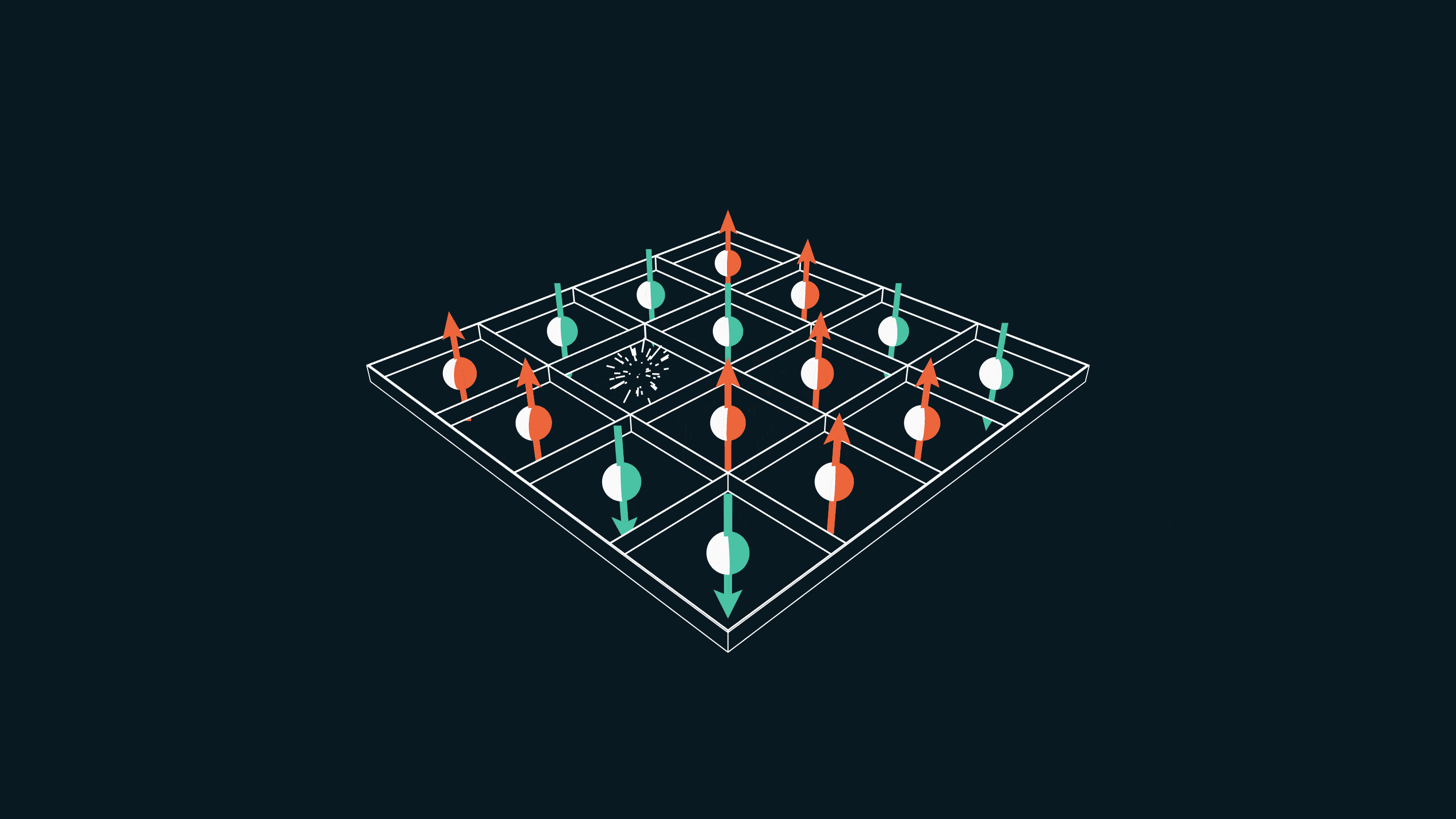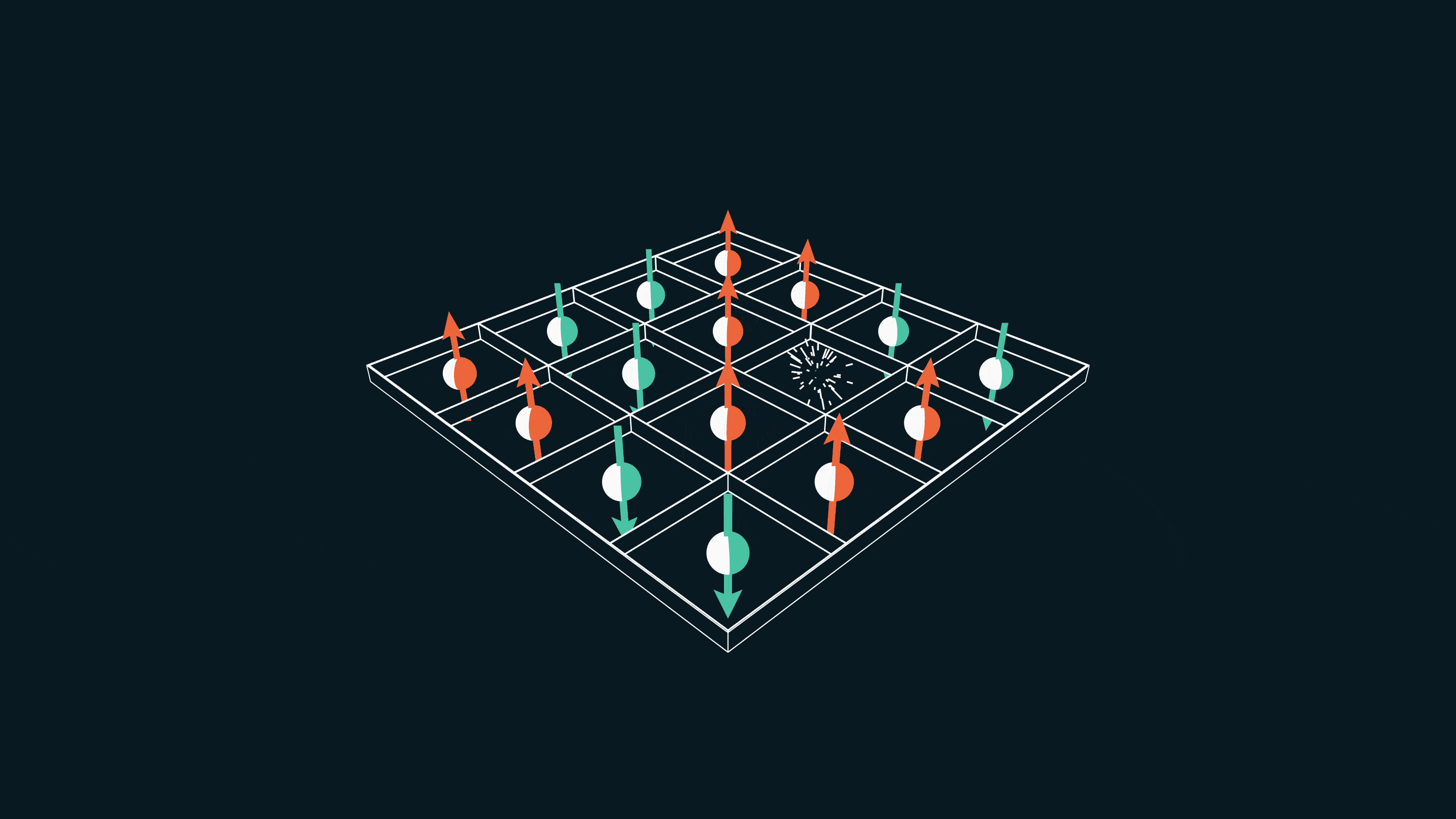
The 15-puzzle asks players to slide numbered tiles around a grid. When the numbers are replaced by the spins of electrons, the puzzle can be used to explain how permanent magnets work.
Dave Whyte for Quanta Magazine
Introduction
For a few months in 1880, entire swaths of the United States succumbed to an addiction the likes of which had never been seen. “It has become literally an epidemic all over the country,” wrote The Weekly News-Democrat in Emporia, Kansas, on March 12, 1880. “Whole cities are distracted, and men are losing sleep and going crazy over it.” The epidemic spread to Europe and as far as Australia and New Zealand.
The disease was a new obsession: a frustratingly simple mechanical game called the 15-puzzle. Still familiar today, it consists of a four-by-four grid in which you slide 15 numbered tiles around, trying to put the numbers in sequence.
The game seems quaint by today’s standards, but in 1880, it was all the rage. “No child is too puerile to be beneath its entertaining powers, and no man is too vigorous or in too high station to escape its fascination,” the News-Democrat wrote. The frustration, perhaps, stemmed from the mathematically proven fact that only half of the puzzle configurations are solvable (likely unbeknownst to the addicted).
Now, nearly 140 years later, the 15-puzzle is of interest again, this time not as a distraction, but as a way to understand a seemingly unrelated and much more complex puzzle: how magnets work.
Permanent magnets such as the ones on your refrigerator are magnetic because of a phenomenon called ferromagnetism. In a ferromagnet, the spins of electrons align, collectively generating a magnetic field. More specifically, metals such as iron, cobalt and nickel demonstrate itinerant ferromagnetism, which refers to the fact that their electrons can move around freely within the material. Each electron also has an intrinsic magnetic moment, but to understand exactly how and why all those magnetic moments align in a magnet demands calculating the quantum interactions among all the electrons, which is prohibitively complex.
“Itinerant ferromagnetism is actually one of the hardest problems in theoretical condensed matter physics,” said Yi Li, a physicist at Johns Hopkins University.
But Li and two graduate students, Eric Bobrow and Keaton Stubis, may be just a bit closer to solving the problem. Using the mathematics of the 15-puzzle, they expanded a well-known theorem that describes an idealized case of itinerant ferromagnetism. In their new analysis, published in the journal Physical Review B, they extend the theorem to explain a broader and more realistic system, potentially leading to a more rigorous model of how magnets work.
“This is a beautiful paper,” said Daniel Arovas, a physicist at the University of California, San Diego. “Especially because rigorous results for the case of itinerant ferromagnets are rather few and far between, I really like this work.”
Hole Hop
At the most basic level, electrons in a metal have to abide by two big constraints. First, they’re all negatively charged, so they all repel one another. In addition, electrons must obey the so-called Pauli exclusion principle, which states that no two particles can occupy the same quantum state. This means that electrons with the same property of “spin” — which is proportional to the electron’s magnetic moment — cannot occupy the same quantum state around an atom in the metal. Two electrons with opposite spins, however, can.
It turns out the easiest way for an ensemble of freely moving electrons to satisfy both their mutual repulsion and the constraints of the Pauli exclusion principle is for them to stay apart and for their spins to align — and thus become ferromagnetic.

Lucy Reading-Ikkanda/Quanta Magazine
But this is just a simplified sketch. What’s eluded physicists is a detailed model of how such an organized pattern of aligned spins emerges from the countless quantum interactions between individual electrons. For example, Li explained, an electron’s wave function — the complex mathematical description of its quantum properties — can be entangled with another electron’s wave function. To fully understand how the behavior of individual particles leads to the collective phenomenon of ferromagnetism, you would need to keep track of the wave function of every electron in a system as it continually reshapes every other electron’s wave function through their mutual interactions. In practice, this widespread entanglement makes the full, rigorous equations needed to describe ferromagnetism impossible to write down.
Instead, physicists like Li are trying to glean insight by studying simpler idealized models that capture the underlying physics of ferromagnetism. In particular, her recent work expands on a milestone discovery made more than 50 years ago.
In the mid-1960s, two physicists heralding from opposite sides of the globe independently derived a proof that explained why electrons should align and create a ferromagnetic state. David Thouless, a physicist then at Cambridge University who would go on to win the Nobel Prize in 2016, and Yosuke Nagaoka, a physicist visiting the University of California, San Diego, from Nagoya University at the time, published their proofs in 1965 and 1966, respectively. Their result, called the Nagaoka-Thouless theorem (also Nagaoka’s theorem), relies on an idealized system of electrons on an atomic lattice. So while it didn’t explain real-world magnets, it was nevertheless important because it showed, for the first time, in principle why electron spins should align. And because their analyses were mathematical proofs, they were exact, unburdened by the approximations typical in physics.
To understand the theorem, imagine a two-dimensional square lattice. Each vertex can accommodate two electrons of opposite spins, but the theorem assumes that it would require an infinite amount of energy for two electrons to occupy a single site. This ensures only one electron resides in each slot. In this configuration, each electron can be spinning either up or down. They don’t have to be aligned, so the system is not necessarily ferromagnetic.
Now take away one electron. What remains is a vacancy called a hole. An adjacent electron can slide over into the hole, leaving behind another vacancy. Another electron can scoot over into the new opening and leave behind another new hole. In this way, the hole effectively hops from one site to another, shuttling around the lattice. Thouless and Nagaoka found that in this scenario, with the addition of just a single hole, the electrons would spontaneously align. This was, they proved, the lowest energy state, one that’s ferromagnetic.
For the system to be in the lowest energy state, Arovas explained, the hole must be free to roam without disturbing the configuration of electron spins — a process that would require extra energy. Yet as the hole moves, the electrons also move around. For the electrons to move without altering the configuration of spins, the electrons must be aligned.
“Nagaoka’s theorem is one of the few examples with which you can mathematically prove instances of ferromagnetism,” said Masaki Oshikawa, a physicist at the University of Tokyo. “But from a physics point of view, it’s very artificial.”
For example, it costs a lot of energy for two electrons to overcome their mutual repulsion and settle in the same site — but not infinite energy, as the theorem demands. The Nagaoka-Thouless picture also only applies to simple lattices: two-dimensional lattices of squares or triangles, or a three-dimensional cubic lattice. In nature, however, ferromagnetism arises in many metals with all kinds of structures.
If the Nagaoka-Thouless theorem really explains ferromagnetism, then it should apply to all lattices. People assumed this was likely the case, Li said. “But no one really gave a clear proof.” That is, until now.
Spin Tiles
In 1989, Hal Tasaki, a physicist at Gakushuin University in Japan, extended the theorem somewhat, finding that it would apply as long as a lattice has a mathematical property called connectivity. Take the simple case of a square lattice with one moving hole. If, after moving the hole around, you can create every configuration of spins while preserving the number of spin-up and spin-down electrons, then the connectivity condition is satisfied.
But other than the square and triangle lattices and the three-dimensional cubic, it wasn’t clear whether the connectivity condition would be satisfied in other cases — and thus whether the theorem applies more generally.
To tackle this question, Li started by focusing on the six-sided honeycomb lattice. As her students, Bobrow and Stubis, worked on the problem, they realized it resembled that 19th-century obsession: the 15-puzzle. Just swap the labels on the tiles from numbers to up or down spins, and the puzzle becomes equivalent to a Nagaoka ferromagnet, with a hole that moves through a lattice of electrons.
The puzzle is solved when you can reorder the tiles to make any sequence you want, which is precisely the meaning of the connectivity condition. So whether the connectivity condition is satisfied for a given lattice becomes a question of whether an equivalent puzzle with that lattice structure is solvable.
It turns out that back in 1974, a mathematician named Richard Wilson, now at the California Institute of Technology, had figured it out, generalizing and solving the 15-puzzle for all lattices. As part of his proof, he showed that for almost all nonseparable lattices (which are those whose vertices remain linked even after removing one vertex), you could slide the tiles around and get any configuration you want, so long as you make an even number of moves. The only exceptions are single polygons larger than a triangle, and something called the θ0 (“theta zero”) graph, in which a vertex in the center of a hexagon is connected to two opposite vertices.
The researchers could then directly apply the results of Wilson’s proof to the Nagaoka-Thouless theorem. For a system of electrons and a single hole, they proved that the connectivity condition is satisfied for nearly all lattices, including common structures like the two-dimensional honeycomb and the three-dimensional diamond lattices. The two exceptions — polygons larger than a triangle and the θ0 graph — are not structures you would find in a realistic ferromagnetic anyway.
Hole Explosion
Using the 15-puzzle is a fresh and potentially fruitful approach, said Sriram Shastry, a physicist at the University of California, Santa Cruz. “I like the fact that they brought in new language, a new set of connections with graph theory,” he said. “The connection I think is rich — it can be a rich source of insights in the future.” But while the study takes a significant step forward, problems remain.
One complication is that the Nagaoka-Thouless theorem doesn’t always work when the moving hole has to take an odd number of steps as it loops around a lattice, Shastry said. Perhaps the most glaring problem, however, is that the theorem requires the presence of exactly one hole — no more, no less. In metals, though, holes are abundant, often filling up half the lattice.
But physicists have tried generalizing the theorem to multiple-hole systems. Using numerical calculations, physicists have shown that Nagaoka ferromagnetism seems to work for a square lattice of finite size that’s up to 30 percent filled with holes. In the current paper, the researchers applied exact analytic techniques to the two-dimensional honeycomb lattice and the three-dimensional diamond lattice. Nagaoka ferromagnetism seems to exist as long as the number of holes is less than the number of lattice sites raised to the 1/2 power for the honeycomb, or the 2/5 power for the diamond.
These exact solutions could lead to a more complete model of itinerant ferromagnetism. “This is just one small step forward to set up some rigorous mathematical starting point for future study,” Li said.
This article was reprinted on Wired.com.




