Latest Articles
Can AI Models Show Us How People Learn? Impossible Languages Point a Way.
Certain grammatical rules never appear in any known language. By constructing artificial languages that have these rules, linguists can use neural networks to explore how people learn.
Why Computer Scientists Consult Oracles
Hypothetical devices that can quickly and accurately answer questions have become a powerful tool in computational complexity theory.
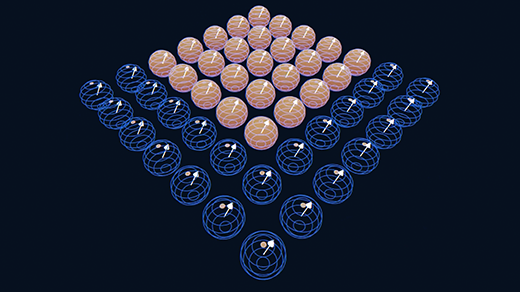
Quantum Computers Cross Critical Error Threshold
In a first, researchers have shown that adding more “qubits” to a quantum computer can make it more resilient. It’s an essential step on the long road to practical applications.
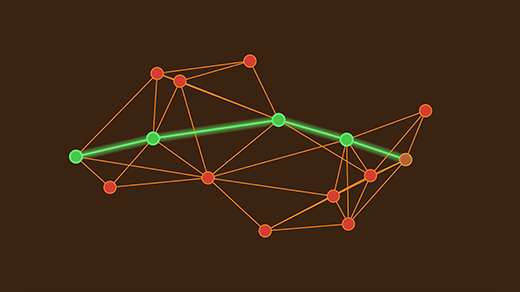
Computer Scientists Establish the Best Way to Traverse a Graph
Dijkstra’s algorithm was long thought to be the most efficient way to find a graph’s best routes. Researchers have now proved that it’s “universally optimal.”
Computer Scientists Combine Two ‘Beautiful’ Proof Methods
Three researchers have figured out how to craft a proof that spreads out information while keeping it perfectly secret.
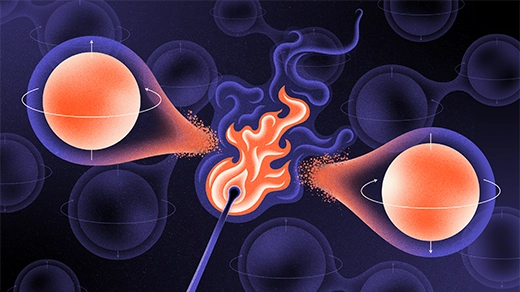
Computer Scientists Prove That Heat Destroys Quantum Entanglement
While devising a new quantum algorithm, four researchers accidentally established a hard limit on entanglement.
With Fifth Busy Beaver, Researchers Approach Computation’s Limits
After decades of uncertainty, a motley team of programmers has proved precisely how complicated simple computer programs can get.
Cryptographers Discover a New Foundation for Quantum Secrecy
Researchers have proved that secure quantum encryption is possible in a world without hard problems.
Cryptography Tricks Make a Hard Problem a Little Easier
Researchers have shown how to find the simplest description of a data set faster than by simply checking every possibility.