Big Question About Primes Proved in Small Number Systems

On September 7, two mathematicians posted a proof of a version of one of the most famous open problems in mathematics. The result opens a new front in the study of the “twin primes conjecture,” which has bedeviled mathematicians for more than a century and has implications for some of the deepest features of arithmetic.

“We’ve been stuck and running out of ideas on the problem for a long time, so it’s automatically exciting when anyone comes up with new insights,” said James Maynard, a mathematician at the University of Oxford.

The twin primes conjecture concerns pairs of prime numbers with a difference of 2. The numbers 5 and 7 are twin primes. So are 17 and 19. The conjecture predicts that there are infinitely many such pairs among the counting numbers, or integers. Mathematicians made a burst of progress on the problem in the last decade, but they remain far from solving it.

The new proof, by Will Sawin of Columbia University and Mark Shusterman of the University of Wisconsin, Madison, solves the twin primes conjecture in a smaller but still salient mathematical world. They prove the conjecture is true in the setting of finite number systems, in which you might only have a handful of numbers to work with.

These number systems are called “finite fields.” Despite their small size, they retain many of the mathematical properties found in the endless integers. Mathematicians try to answer arithmetic questions over finite fields, and then hope to translate the results to the integers.

“The ultimate dream, which is maybe a bit naive, is if you understand the finite field world well enough, this might shed light on the integer world,” Maynard said.

In addition to proving the twin primes conjecture, Sawin and Shusterman have found an even more sweeping result about the behavior of primes in small number systems. They proved exactly how frequently twin primes appear over shorter intervals — a result that establishes tremendously precise control over the phenomenon of twin primes. Mathematicians dream of achieving similar results for the ordinary numbers; they’ll scour the new proof for insights they could apply to primes on the number line.

A New Kind of Prime

The twin primes conjecture’s most famous prediction is that there are infinitely many prime pairs with a difference of 2. But the statement is more general than that. It predicts that there are infinitely many pairs of primes with a difference of 4 (such as 3 and 7) or 14 (293 and 307), or with any even gap that you might want.

Alphonse de Polignac posed the conjecture in its current form in 1849. Mathematicians made little progress on it for the next 160 years. But in 2013 the dam broke, or at least sprung major leaks. That year Yitang Zhang proved that there are infinitely many prime pairs with a gap of no more than 70 million. Over the next year other mathematicians, including Maynard and Terry Tao, closed the prime gap considerably. The current state of the art is a proof that there are infinitely many prime pairs with a difference of at most 246.

But progress on the twin primes conjecture has stalled. Mathematicians understand they’ll need a wholly new idea in order to solve the problem completely. Finite number systems are a good place to look for one.

To construct a finite field, start by extracting a finite subset of numbers from the counting numbers. You could take the first five numbers, for instance (or any prime number’s worth). Rather than visualizing the numbers along a number line the way we usually do, visualize this new number system around the face of a clock.

Arithmetic then proceeds, as you might intuit it, by wrapping around the clock face. What’s 4 + 3 in the finite number system with five elements? Start at 4, count three spaces around the clock face, and you’ll arrive at 2. Subtraction, multiplication and division work similarly.

Only there’s a catch. The typical notion of a prime number doesn’t make sense for finite fields. In a finite field, every number is divisible by every other number. For example, 7 isn’t ordinarily divisible by 3. But in a finite field with five elements, it is. That’s because in this finite field, 7 is the same number as 12 — they both land at 2 on the clock face. So 7 divided by 3 is the same as 12 divided by 3, and 12 divided by 3 is 4.

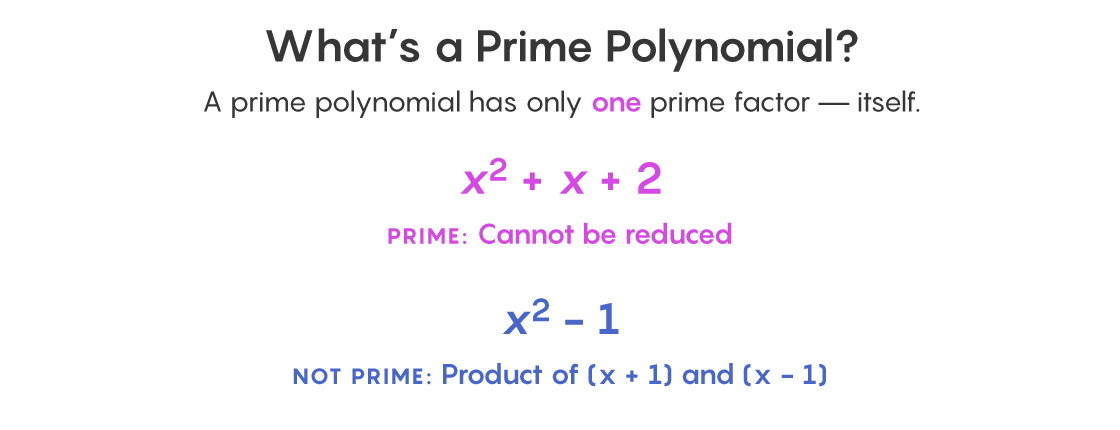
Because of this, the twin primes conjecture for finite fields is about prime polynomials — mathematical expressions such as x² + 1.

For example, let’s say your finite field contains the numbers 1, 2 and 3. An polynomial in this finite field would have those numbers as coefficients, and a “prime” polynomial would be one that can’t be factored into smaller polynomials. So x² + x + 2 is prime because it cannot be factored, but x² − 1 is not prime: It’s the product of (x + 1) and (x − 1).

Once you have the notion of prime polynomials, it’s natural to ask about twin prime polynomials — a pair of polynomials that are both prime and that differ by a fixed gap. For example, the polynomial x² + x + 2 is prime, as is x² + 2x + 2. The two differ by the polynomial x (add x to the first to get the second).

The twin primes conjecture for finite fields predicts that there are infinitely many pairs of twin prime polynomials that differ not just by x, but by any gap you want.

Clean Cuts

Finite fields and prime polynomials might seem contrived, of little use in learning about numbers in general. But they’re analogous to a hurricane simulator — a self-contained universe that provides insights about phenomena in the wider world.

“There is an ancient analogy between integers and polynomials, which allows you to transform problems about integers, which are potentially very difficult, into problems about polynomials, which are also potentially difficult, but possibly more tractable,” Shusterman said.

Finite fields burst into prominence in the 1940s, when André Weil devised a precise way of translating arithmetic in small number systems to arithmetic in the integers. Weil used this connection to spectacular effect. He proved arguably the most important problem in mathematics — the Riemann hypothesis — as interpreted in the setting of curves over finite fields (a problem known as the geometric Riemann hypothesis). That proof, along with a series of additional conjectures that Weil made — the Weil conjectures — established finite fields as a rich landscape for mathematical discovery.

Weil’s key insight was that in the setting of finite fields, techniques from geometry can be used with real force to answer questions about numbers. “This is part of the thing that’s special to finite fields. Many problems you want to solve, you can rephrase them geometrically,” Shusterman said.

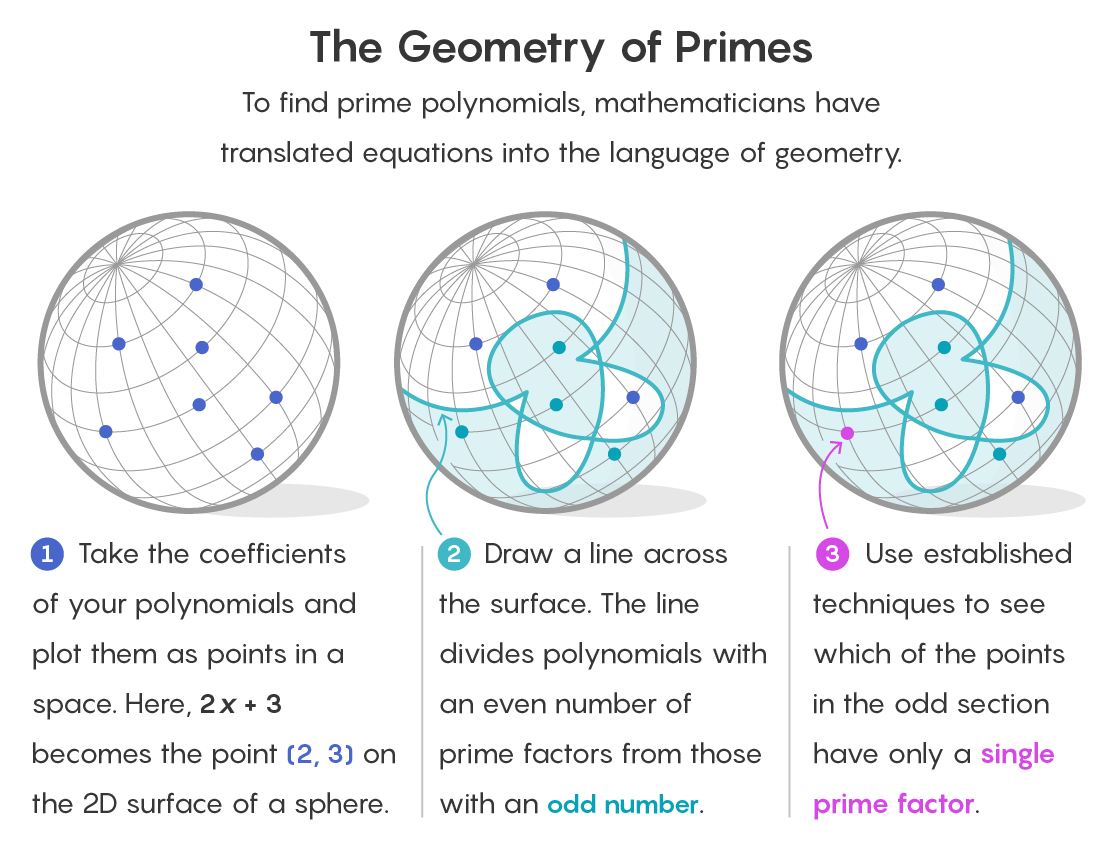
To see how geometry arises in such a setting, imagine each polynomial as a point in space. The polynomial’s coefficients serve as the coordinates that define where the polynomial is located. Going back to our finite field of 1, 2 and 3, the polynomial 2x + 3 would be located at the point (2, 3) in two-dimensional space.

But even the simplest finite field has an infinite number of polynomials. You can construct more elaborate polynomials by increasing the size of the largest exponent, or degree, of the expression. In our case, the polynomial x² − 3x − 1 would be represented by a point in three-dimensional space. The polynomial 3x⁷ + 2x⁶ + 2x⁵ − 2x⁴ − 3x³ + x² − 2x + 3 would be represented by a point in eight-dimensional space.

In the new work, this geometric space represents all polynomials of a given degree for a given finite field. The question then becomes: Is there a way to isolate all the points representing prime polynomials?

Sawin and Shusterman’s strategy is to divide the space into two parts. One of the parts will have all the points corresponding to polynomials with an even number of factors. The other part will have all the points corresponding to polynomials with an odd number of factors.

Already this makes the problem simpler. The twin primes conjecture for finite fields concerns polynomials with just one factor (just as a prime number has a single factor — itself). And since 1 is odd, you can discard the part of the space with the even factors entirely.

The trick is in the dividing. In the case of a two-dimensional object, such as the surface of a sphere, the thing that cuts it in two is a one-dimensional curve, just as the equator cuts the surface of the Earth in half. A higher-dimensional space can always be cut with an object that has one fewer dimension.

Yet the lower-dimensional shapes that divide the space of polynomials are not nearly as elegant as the equator. They are sketched by a mathematical formula called the Möbius function, which takes a polynomial as an input and outputs 1 if the polynomial has an even number of prime factors, −1 if it has an odd number of prime factors, and 0 if it has only a repeated factor (the way 16 can be factored into 2 × 2 × 2 × 2).

The curves drawn by the Möbius function twist and turn wildly, crossing themselves in many places. The places where they cross — called singularities — are especially difficult to analyze (and they correspond to polynomials with a repeated prime factor).

Sawin and Shusterman’s principal innovation was in finding a precise way to slice the lower-dimensional loops into shorter segments. The segments were easier to study than the complete loops.

Once they cataloged polynomials with an odd number of prime factors — the hardest step — Sawin and Shusterman had to determine which of them were prime, and which were twin primes. To do this, they applied several formulas that mathematicians use to study primes among the regular numbers.

Sawin and Shusterman used their technique to prove two major results about prime polynomials in certain finite fields.

First, the twin primes conjecture for finite fields is true: There are infinitely many pairs of twin prime polynomials separated by any gap you choose.

Second, and even more consequentially, the work provides a precise count of the number of twin prime polynomials you can expect to find among polynomials of a given degree. It’s analogous to knowing how many twin primes fall within any sufficiently long interval on the number line — a kind of dream result for mathematicians.

“This is the first work that gives a quantitative analogue of what is expected to be true over the integers, and that is something that really stands out,” said Zeev Rudnick of Tel Aviv University. “There hasn’t been anything like this until now.”

Sawin and Shusterman’s proof shows how nearly 80 years after André Weil proved the Riemann hypothesis in curves over finite fields, mathematicians are still energetically following his lead. Mathematicians pursuing the twin primes conjecture will now turn to Sawin and Shusterman’s work and hope that it, too, will provide a deep well of inspiration.

Correction: On September 27, 2019, this article was edited to remove references to polynomial “equations.” The polynomials referenced in this article are expressions, not equations.

This article was reprinted on Wired.com.