A Scientist Who Delights in the Mundane

L. Mahadevan has made a career out of exploring the science behind everyday phenomena, like the shape of an apple or how the brain folds.
Ken Richardson for Quanta Magazine
Introduction
The complex dynamics behind a dripping faucet, the ways adhesive pads can fail, the cracking of mud — these matters might seem unimportant, or even boring. L. Mahadevan disagrees. A professor of applied mathematics, physics, and organismic and evolutionary biology at Harvard University, Mahadevan uses mathematics and physics to explore commonplace phenomena, showing that many of the objects and behaviors we take for granted, and consequently give little thought to, are quite extraordinary upon closer examination.
For Mahadevan, the everyday world holds great fascination. Paper has proven particularly intriguing: Mahadevan has described the “out-of-plane deformations” that arise when wet paper bends and buckles, the geometry of a crumpled sheet of paper, and the aerodynamic behavior of falling paper. But he’s also provided the definitive explanation of the “Cheerios effect” — the propensity of breakfast cereals suspended in milk to clump together or cling to the bowl. He even took on a process often dismissed as the dullest thing imaginable in his essay “Watching Paint Dry,” written for a Harvard undergraduate journal.
That essay, he noted, “was just an attempt to tell students they don’t always have to follow the crowd. Everyone says they should work on the big questions, which is fine, but there’s also something to be said for working on the little questions and then gradually building a broader understanding.”
Although Mahadevan’s approach is clearly unorthodox, his work has earned him recognition and acclaim. After receiving a doctorate from Stanford University in 1995, he’s held appointments at a string of prestigious institutions, including the Massachusetts Institute of Technology, the University of Cambridge and Harvard, while publishing more than 300 scientific papers. He’s also a fellow of the Royal Society of London. His pursuits have earned him a Guggenheim fellowship in 2006, an Ig Nobel Prize (in physics) in 2007 and a MacArthur fellowship in 2009 — the last for applying “complex mathematical analyses to a variety of seemingly simple, but vexing, questions across the physical and biological sciences.”
Mahadevan is grateful that the schools he’s worked for have always given him the freedom to explore his surroundings as he sees fit. “Finding the sublime in the mundane is an old goal,” he said. “Since the everyday world is messy, with many phenomena continuously vying for attention, there is no dearth of problems. Consequently, I never expect to be bored.”
Quanta Magazine spoke with Mahadevan recently in person (outdoors, at a safe distance) and via video call. The interview has been condensed and edited for clarity.

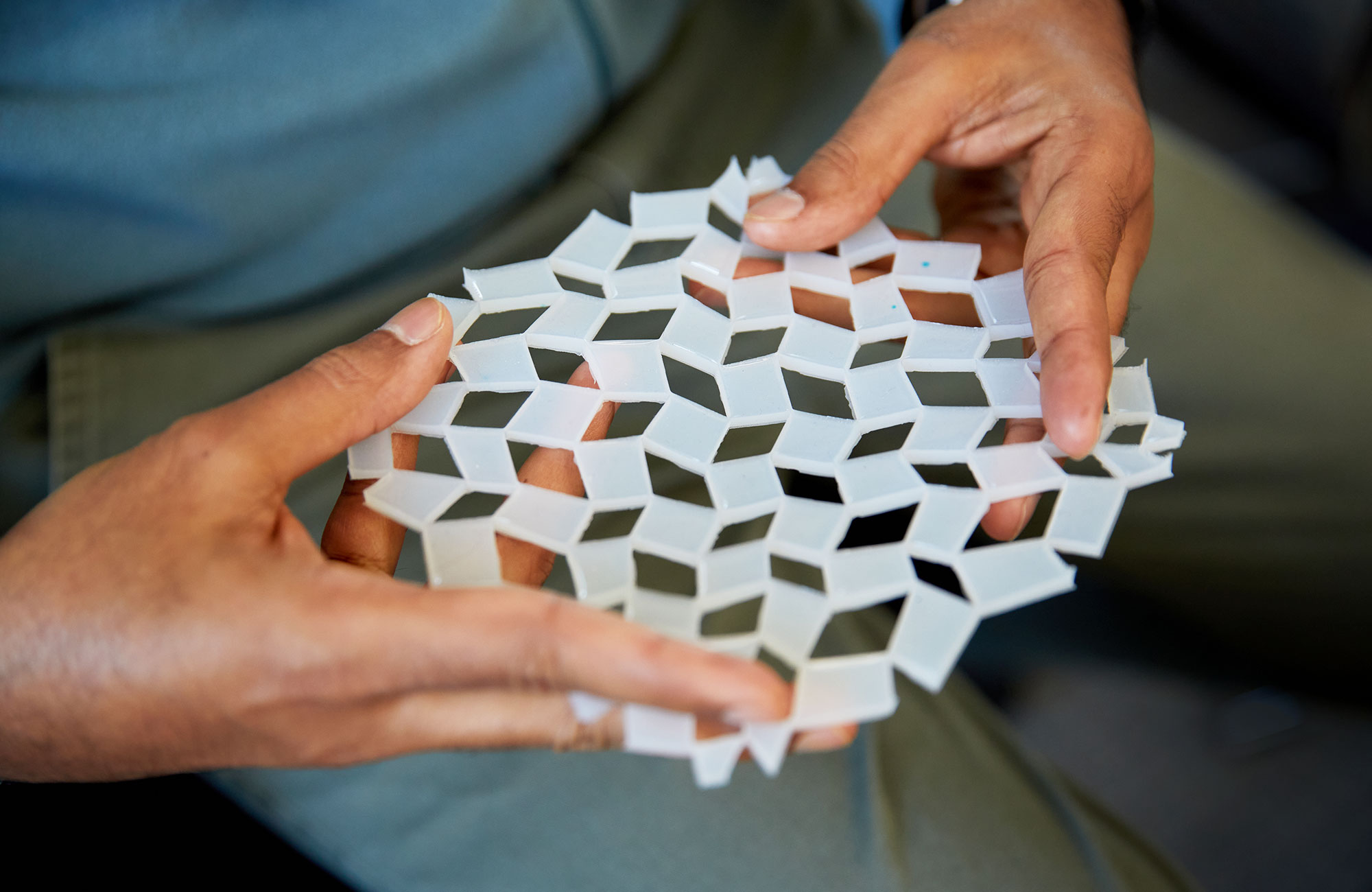
One of Mahadevan’s enduring interests is the mathematics of deformations of paper, including the folds made in origami (red) and the cuts of kirigami (white).
Ken Richardson for Quanta Magazine
When did you realize you wanted to study things that others might consider frivolous?
I’ve always been this way, which may be a cultural thing. I’m not the kind of person who thinks some problems are bigger than others. In my mind, there is no hierarchy. What is frivolous and what is important seems like an irrelevant question. After all, nature does not care!
That perspective may stem from regularly finding myself “in between things.” After coming to the U.S. from India, I was between engineering and mathematics as a student at Stanford and later as a faculty member at MIT, then between mathematics and physics at Cambridge, and now between biology, mathematics and physics at Harvard. One positive aspect of being between disciplines is being left alone to find my own way through the weeds. I have delighted in exploring many little vistas, and I’m always surprised when others take pleasure in these marginalia.
You’ve had a decades-long preoccupation with paper — crumpled, wet, folded, cut or falling. Why the fascination?
You start with one little question and it turns into something much bigger. I began thinking about a crumpled sheet of paper more than 20 years ago, early in my scientific career. There was no epiphany. I was just contemplating its structure — its creases and corners. We still don’t know how to describe the precise physics and mathematics of crumpling, just where and how the paper crumples. The problem involves differential geometry and differential equations. And there may be an analogy in general relativity because inside a crumpled sheet of paper there are many singularities, like black holes, and these singularities can touch and interact with each other. So it’s an interesting, beautiful problem. But it’s still an unsolved problem.
Fortunately, science is very forgiving. You can make mistakes or go astray for a while, but that doesn’t matter in the long run. Science is self-correcting, and what matters is what you ultimately get right.
As for getting something “right,” what recent success have you had with origami?
Whereas crumpling a sheet of paper is an extremely disordered process, folding can be very ordered, and that’s what origami is all about. Earlier this year we showed how to approximate any three-dimensional shape by taking a flat sheet and introducing folds. The way to do that turns out to be the opposite of the usual approach. Normally people start folding on the paper’s boundaries and move inward, but instead we start in the center of the paper and move outward from there. That’s something no one has done before, and it enables us to reproduce any shape. Meanwhile, we’ve converted this new approach to an algorithm that could potentially be used for computational or manufacturing purposes.
And you’ve had similar results with kirigami, which is like origami with scissors?
In some ways, kirigami is even more beautiful, because now you can make cuts. We’ve proved, once again, that we can completely control the shape. And by using the mathematical principles we’ve uncovered [from discrete differential geometry], we can devise an algorithm that tells us the pattern of cuts needed to create any 3D shape from a flat sheet. Our next step is to combine cuts and folds, thereby becoming even better tailors.
Moving into the natural world, you’ve also been studying termite mounds recently. How did you first come across them, and what have you learned?
While visiting an agricultural college in Bangalore [India] in 2009, I saw a termite mound for the first time during a stroll through campus. I knew little about them but decided it would be a cool thing to study.
Termites are considered among the greatest architects on Earth. A couple of years ago, a network of termite mounds in Brazil was discovered that is as large as Great Britain. Inside each mound, which is a few meters tall, millions of millimeter-sized termites live. That’s comparable to humans living in buildings a few kilometers high. The mounds are built to harness the environment — places where temperature, humidity and gas concentrations are well controlled.
We have studied the function of termite mounds in both India and Namibia, and most recently have begun to understand the principles of how they are built. Our experiments showed that the mound operates like a lung, breathing once a day in response to external temperature changes. And we have a mathematical model that shows how the mound geometry, environmental conditions and termite behavior are all interrelated.

As well as studying paper, Mahadevan has explored how the human brain folds.
Ken Richardson for Quanta Magazine
Are termite mounds comparable to other structures in any way?
We build buildings that, like termite mounds, are not completely porous but not completely insulated either. That metaphor transfers to the most basic forms of life. For example, a cell is not a cell without an envelope that enables it to communicate with the outside world, allowing for the transfer of energy, matter and information — not completely insulated nor completely porous.
From our work on termites, we were motivated to ask similar questions about bees and ants, which also live in large colonies. We learned, for instance, that bees maintain the temperature within a bee box [or hive] by fanning their wings near the entrance. Conical clusters of bees hanging from a tree branch have another way of regulating their temperature: They huddle together when it’s cold and spread out when it’s warmer. And if the branch is shaken, the cluster flattens itself to increase stability and keep bees from being thrown from the tree. That’s similar to what you might instinctively do when the ground below is shaken — crouch to keep from falling down.
The bigger question, of course, is how organisms can collectively solve problems without planning, or a designated planner, on scales much larger than an individual. We’re starting to look into these same questions with ants, which can have huge extended colonies, and robots.
You’ve also shown how the geometry, or shape, of a termite mound affects the diffusion of heat and pheromones. How else has geometry figured in your work?
A couple of years ago, we looked at avian eggs from three different angles. First, we quantified the egg shapes of more than 1,400 species by determining an egg’s eccentricity — how far it deviates from a sphere — and asymmetry. Second, we showed how a particular egg shape comes to be: The membrane inside the shell behaves like a pressurized balloon, and shape arises from varying the thickness of the balloon, not from the stiff shell. Finally, we considered the functional [and evolutionary] aspects of egg shape and made a striking finding: Narrow, elongated eggs correlate with better flight ability, although some scientists are not convinced of this.
Shape comes up all the time in my work. We recently analyzed the geometry and physics of how mammalian brains fold and how vertebrate intestines loop and coil. Along a similar vein, we have just analyzed the shape of an apple [in not-yet-published research]. The most interesting thing is not that it’s almost spherical, but rather the beautiful cusplike feature where the stalk meets the fruit. That feature is symmetric in a Fuji apple but not in a Red Delicious. We tried to describe it mathematically and mimic it in the lab, which we were able to do with gel.
Why do we care? There are two reasons. For one thing, creases in the brain and cusps in apples are singularities — just like breaking waves. And as Arthur Conan Doyle once wrote, a “singularity is almost invariably a clue.” The other reason is that it’s right in front of us. We don’t have to use a telescope, or a microscope, or spend a billion dollars to study it — just an inquisitive eye.


Mahadevan’s lab is full of experiments, here testing ideas about liquid suspension and how certain materials precipitate according to mathematical models.
Ken Richardson for Quanta Magazine
Much of your work sounds pretty abstract. How much thought do you give to possible applications?
I actually think my work is far from abstract. I work on things that everyone can see and experience, but few care to think about deeply. As for the second question, does an artist, musician or writer think about applications? Why does science have to do so? It is human to be curious. That is enough, isn’t it?
But I should add that I’m not at all snooty about working on useful or practical things. I have patents on a few devices and algorithms, and just this year we developed potential protocols for mitigating the extreme costs of pandemics.
On the other hand, I also like doing things for the sheer fun of it, like, for instance, designing a fair three-sided coin in order to decide a three-way bet.
Which of course raises the question: How do you make a three-sided coin?
A normal coin lands with heads facing up half the time, tails facing up half the time — almost never on its side. But if you make the coin really thick, so it becomes a long cylinder, it will land on its side almost 100% of the time. How thick does the coin have to be to land on its side exactly one-third of the time? The mathematician John von Neumann is said to have determined that a coin has a 1/3 chance of landing on its edge if the ratio of its thickness to its diameter is 1/(2√2). About a decade ago we showed that if you factor in the conservation of angular momentum, you get a different answer: A fair three-sided coin should have a width-to-diameter ratio of 1/√3. One way of picturing that is to glue eight quarters together. We also carried out experiments confirming that we were right, and we devised a method for creating customized coins with varying probabilities of heads, tails and “sides.”
How do you think this kind of work fits into the greater body of research?
I work on things nobody cares about, and I’m quite happy that way. You can learn about the world by looking at the humblest of things. You don’t have to study cosmology or cure cancer. As William Blake once wrote: “To see … Heaven in a Wild Flower / Hold Infinity in the palm of your hand.” And we have studied the blooming of flowers and the formation of creaselike infinities like those in the palm of one’s hand.
I also believe that the work I’ve done shows that you don’t have to struggle to ask very deep and profound questions about the world. Maybe there is a thread that winds through the various things I’ve taken up. My opinion is that the fabric this thread is woven into will only be visible, if at all, at the end of this journey. But for now, all I really care about is the journey itself.
Where do you get your ideas among the myriad possibilities the world offers?
I walk my dog regularly, and when I do, I often wonder about his behavior. He sniffs the ground and also holds his head up high. He does that to get a sense of the world, olfactorily. Near the ground, he gets an accurate signal, but it’s local. From the air, the signal is inaccurate but long-range. We call the local cue knowledge and its accumulation wisdom, and the canine (and we humans) need both kinds of information to succeed. How did I learn this? From walking my dog.