Mass and Angular Momentum, Left Ambiguous by Einstein, Get Defined
Researchers can now calculate the amount of angular momentum radiating away from an object in a way that doesn’t depend on the observer.
Maggie Chiang for Quanta Magazine
Introduction
More than a century after Albert Einstein unveiled general relativity, his epic theory of gravity has passed every experimental test to which it has been subjected. General relativity has transformed our understanding of gravity, depicting it not as an attractive force between massive objects, as had long been held, but rather as a consequence of the way space and time curve in the presence of mass and energy. The theory has achieved stunning triumphs — from the confirmation in 1919 that light bends in the sun’s gravitational field to 2019 observations that revealed the silhouette of a black hole. It might be surprising to hear, then, that general relativity is still a work in progress.
Even though the equations Einstein introduced in 1915 concern the curvature induced by massive objects, the theory does not offer a simple or standard way of determining what the mass of an object is. Angular momentum — a measure of an object’s rotational motion in space-time — is an even harder to define concept.
Some of the difficulties stem from a feedback loop that is built into general relativity. Matter and energy curve the space-time continuum, but this curvature becomes a source of energy itself, which can cause additional curvature — a phenomenon sometimes referred to as the “gravity of gravity.” And there is no way to separate an object’s intrinsic mass from the extra energy that comes from this nonlinear effect. Moreover, one cannot define momentum or angular momentum without first having a firm grip on mass.
Einstein recognized the challenges involved in quantifying mass and never fully spelled out what mass is or how it can be measured. It was not until the late 1950s and early 1960s that the first rigorous definition was proposed. The physicists Richard Arnowitt, Stanley Deser, and Charles Misner defined the mass of an isolated object, such as a black hole, as viewed from almost infinitely far away, where space-time is almost flat and the object’s gravitational influence approaches zero.
Although this way of calculating mass (known after its authors as “ADM mass”) has proved useful, it doesn’t allow physicists to quantify the mass within a finite region. Say, for instance, that they are studying two black holes that are in the process of merging, and they want to determine the mass of each individual black hole prior to the merger, as opposed to that of the system as a whole. The mass enclosed within any individual region — as measured from the surface of that region, where gravity and space-time curvature might be very strong — is called “quasilocal mass.”

The eminent mathematician Shing-Tung Yau, who retired from Harvard University this spring and moved to Tsinghua University in China, has worked on the mathematical analysis of general relativity since the 1970s.
Pai Li
In 2008, the mathematicians Mu-Tao Wang of Columbia University and Shing-Tung Yau, now a professor at Tsinghua University in China and an emeritus professor at Harvard University, advanced a definition of quasilocal mass that has proved particularly fruitful. In 2015, it enabled them and a collaborator to define quasilocal angular momentum. And this spring, those authors and a fourth collaborator published the first-ever, long-sought definition of angular momentum that is “supertranslation invariant,” meaning it does not depend on where an observer is located or what coordinate system he or she chooses. With such a definition, observers can, in principle, take measurements of ripples in space-time generated by a rotating object and calculate the exact amount of angular momentum carried away from the object by these ripples, which are known as gravitational waves.
“It’s a great result,” Lydia Bieri, a mathematician and general relativity expert at the University of Michigan, said about the March 2022 paper, “and a culmination of intricate mathematical investigations over several years.” Indeed, the development of these facets of general relativity took not just years but many decades.
Staying Quasilocal
In the 1960s, Stephen Hawking came up with a definition of quasilocal mass that is still favored today in some circumstances owing to its simplicity. Seeking to calculate the mass enclosed by a black hole’s event horizon — its invisible spherical boundary — Hawking showed that you can calculate the mass inside any sphere by determining the extent to which incoming and outgoing light rays are bent by the matter and energy contained within. While “Hawking mass” has the virtue of being relatively easy to compute, the definition only works either in a space-time that is spherically symmetric (an idealized condition, as nothing in the real world is perfectly round) or in a “static” (and rather boring) space-time where nothing changes in time.
The search for a more versatile definition continued. In a lecture at Princeton University in 1979, the British mathematical physicist Roger Penrose, another pioneer of black hole physics, identified the task of characterizing quasilocal mass — “where one does not need to go ‘all the way to infinity’ in order for the concept to be meaningfully defined” — as the number-one unsolved problem in general relativity. A definition of quasilocal angular momentum ranked second on Penrose’s list.
Earlier that year, Yau and his former student Richard Schoen, who is now an emeritus professor at Stanford University, proved a major prerequisite for establishing these quasilocal definitions. Namely, they showed that the ADM mass of an isolated physical system — its mass as measured from infinitely far away — can never be negative. The Schoen-Yau “positive mass theorem” constituted an essential first step for defining quasilocal mass and other physical quantities, because space-time and everything in it will be unstable if its energy has no floor but instead can turn negative and keep dropping without limit. (In 1982, Yau won a Fields Medal, the highest honor in mathematics, in part for his work on the positive mass theorem.)

The late British physicist Stephen Hawking, pictured in 1979, devised one of the first definitions of quasilocal mass. While it is appealingly straightforward to calculate, Hawking mass only works in simple scenarios.
Santi Visalli/Getty Images
In 1989, the Australian mathematician Robert Bartnik offered a new definition of quasilocal mass that relied on that theorem. Bartnik’s idea was to take a region of finite size enclosed by a surface and then, by enveloping it with many layers of surfaces of ever-larger area, extend the finite region to one of infinite size so that its ADM mass can be computed. But the region can be extended in many ways, just as a balloon’s surface area could be blown up uniformly or stretched in various directions, each yielding a different ADM mass. The lowest value of ADM mass that can be obtained is, according to Bartnik, the quasilocal mass. “The argument would not have been possible before the positive mass theorem,” explained Wang, “because otherwise the mass could have gone to negative infinity,” and a minimum mass could never be ascertained.
Bartnik mass has been an important concept in mathematics, said the University of Connecticut mathematician Lan-Hsuan Huang, but its main drawback is a practical one: Finding the minimum is extremely difficult. “It is almost impossible to compute an actual number for the quasilocal mass.”
The physicists David Brown and James York came up with an entirely different strategy in the 1990s. They wrapped a physical system in a two-dimensional surface and then tried to determine the mass within that surface based on its curvature. One problem with the Brown-York method, however, is that it can give the wrong answer in a completely flat space-time: The quasilocal mass might turn out positive even when it should be zero.
Still, the approach was utilized in the 2008 paper by Wang and Yau. Building upon Brown and York’s work, as well as on research that Yau had carried out with the Columbia mathematician Melissa Liu, Wang and Yau found a way to bypass the problem of positive mass in totally flat space. They measured the curvature of the surface in two different settings: the “natural” setting, a space-time representative of our universe (where curvature can be rather complex), and a “reference” space-time called Minkowski space that is perfectly flat because it is devoid of matter. Any difference in the curvature between these two settings, they surmised, must be due to the mass confined within the surface — the quasilocal mass, in other words.
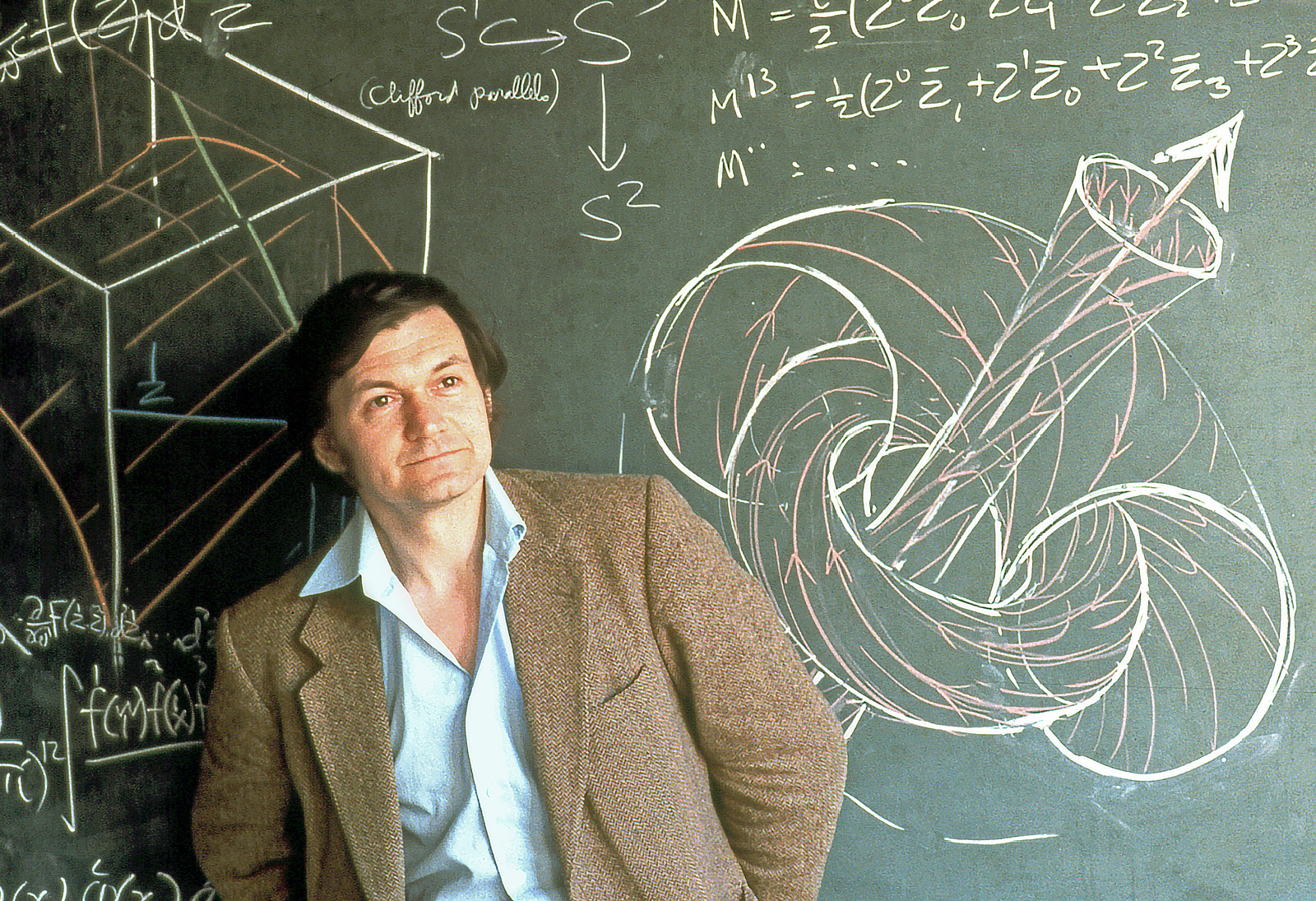
In 1979, a year before this portrait was taken, the mathematical physicist Roger Penrose of the University of Oxford pointed to quasilocal definitions of mass and angular momentum as the first and second most important open problems in general relativity. Penrose would win the 2020 Nobel Prize in physics for showing that black hole formation is a robust prediction of Einstein’s theory.
Anthony Howarth / Science Source
Their definition fulfilled “all the requirements necessary for a valid definition of quasilocal mass,” as they stated in the paper. That said, their approach suffers from one feature that restricts its applicability: “Even though our definition is very precise,” Wang said, “it always involves solving several very difficult nonlinear equations.” The approach is good in theory but often grueling in practice.
Ambiguous Angles
In 2015, Wang and Yau, in concert with Po-Ning Chen of the University of California, Riverside, set out to define quasilocal angular momentum. In classical mechanics, the angular momentum of an object moving in a circle is simply given by its mass times its velocity times the circle’s radius. It’s a useful quantity to measure because it is conserved, meaning it passes between things but is never created or destroyed. Physicists can track how angular momentum is exchanged between objects and the environment to gain insight into a system’s dynamics.
To define the quasilocal angular momentum enclosed within a surface, Wang, Yau and Chen needed two things: a definition of quasilocal mass, which they had, along with detailed knowledge of how rotation works in space-time. As before, they first embedded their surface in the simplest possible milieu, Minkowski space-time — chosen because it is unerringly flat and therefore has the property of rotational symmetry, where every direction looks the same. Rotational symmetry enabled the researchers to define quasilocal angular momentum in a way that does not depend on where you place the origin of the coordinate system you use to measure velocities and distances (the origin is the point where the x, y, z, and t axes intersect). Next, they established a one-to-one correspondence between points on the surface in Minkowski space-time and points on that same surface when placed in its original (natural) space-time, thereby ensuring coordinate independence in the latter setting as well.
The trio then joined forces with Ye-Kai Wang of National Cheng Kung University to tackle a problem that had remained unsolved for about 60 years: how to characterize the angular momentum swept away by gravitational waves, such as those emitted as two black holes spiral together and violently coalesce. Their definition of quasilocal angular momentum wouldn’t work for this task, because the measurement must be done far from the maelstrom rather than in close proximity to the black hole merger. The proper vantage point is called “null infinity,” a notion invented by Penrose that refers to the ultimate destination of outwardly traveling radiation, both gravitational and electromagnetic.
As often happens in general relativity, a new complication arises: The angular momentum transported by gravitational waves, even if measured at null infinity (or far enough away to be a reasonable facsimile), can seem to vary depending on the choice of origin and orientation of an observer’s coordinate system. The difficulty stems from the “gravitational wave memory effect” — the fact that when gravitational waves travel through space-time, they leave a permanent imprint. Waves will expand space-time in one direction and contract it in the orthogonal direction (this is the signal detected by gravitational-wave observatories like LIGO and Virgo), but space-time never reverts exactly to its initial state. “Passing gravitational waves change the distance between objects,” explained Eanna Flanagan, a general relativist at Cornell University. “The waves can also move observers a little bit … but they won’t know that they’ve been moved.”
This means that even if different observers initially agree on where the origin of their coordinate system lies, they won’t agree after gravitational waves have jiggled things around. That uncertainty in turn leads to ambiguities, referred to as “supertranslations,” in their respective assessments of angular momentum. Another way to understand supertranslations is that while neither the mass of an object nor its velocity will be distorted by a passing gravitational wave, the radius of its rotational motion will be. Depending on the orientation of the radius relative to one’s coordinate system, it might seem to be stretched out by gravitational radiation, or shrunk, leading to different possible determinations of angular momentum.
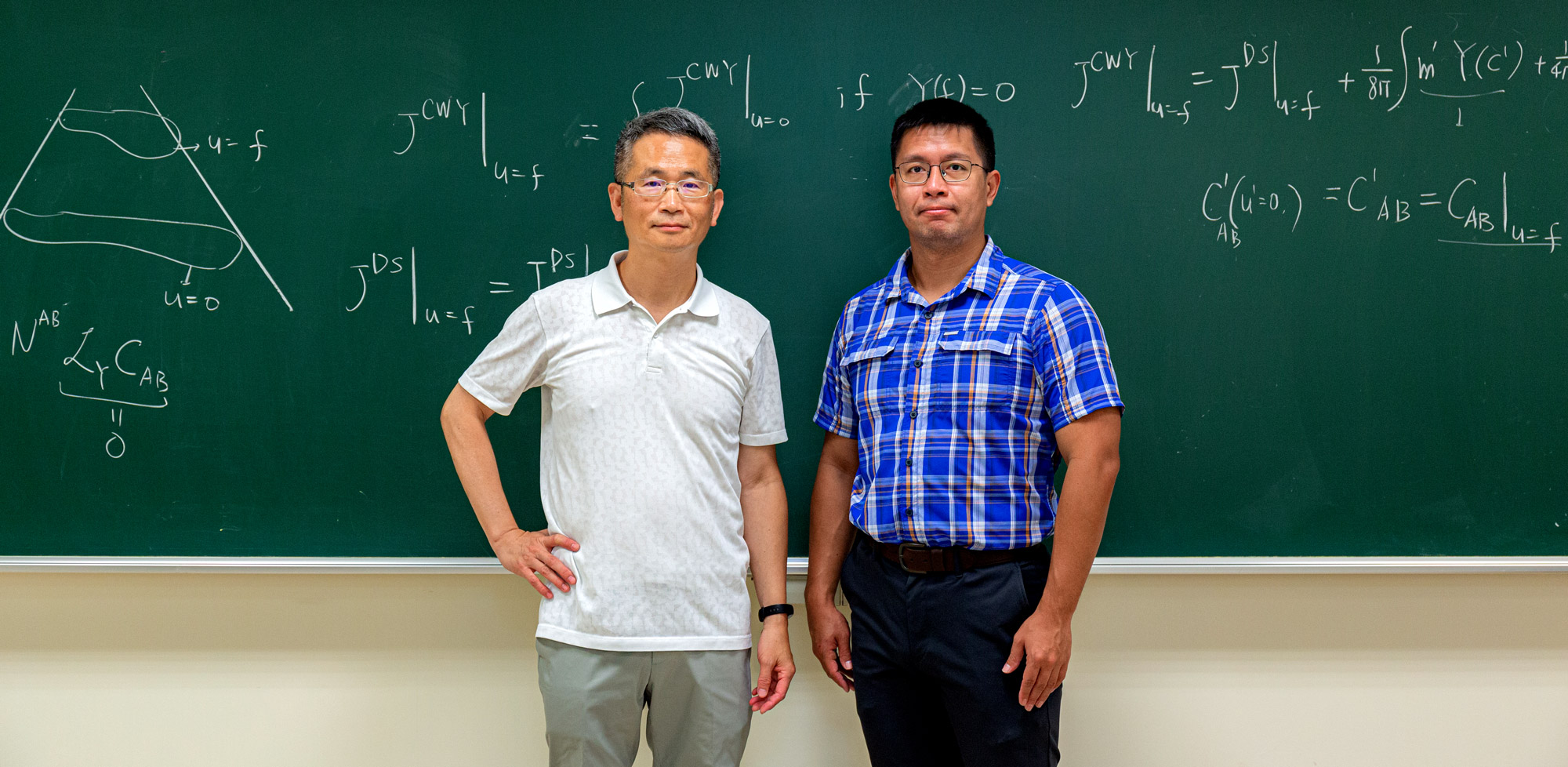
Mu-Tao Wang (left) and Po-Ning Chen in the seminar room at National Taiwan University where they did part of their work on supertranslation-invariant angular momentum.
Po-Chieh Chen
Conserved physical quantities should not vary, or appear to do so, based on how we choose to label things. That was the situation that Chen, Wang, Wang, and Yau hoped to rectify. Starting with their 2015 definition of quasilocal angular momentum, they computed the angular momentum contained within a region of finite radius. Then they took the limit of that quantity as the radius goes to infinity, which turned the coordinate-independent quasilocal definition into a supertranslation invariant quantity at null infinity. With this first-ever supertranslation invariant definition of angular momentum, published in March in Advances in Theoretical and Mathematical Physics, one could, in principle, determine the angular momentum carried away by the gravitational waves emitted during a black hole collision.
“This is a wonderful paper and a wonderful result,” said Marcus Khuri, a mathematician at Stony Brook University in New York, “but the question is, how useful is it?” He explained that the new definition is abstract and hard to compute, “and, generally speaking, physicists don’t like things that are hard to compute.”
A Unique Choice
Hard to compute, however, is an almost unavoidable feature of general relativity. It’s usually not even possible to exactly solve the nonlinear equations that Einstein formulated in 1915 except in highly symmetrical situations. Instead, researchers rely on supercomputers to obtain approximate solutions. They make the problem manageable by breaking space-time into small grids and estimating the curvature of each grid separately and at separate moments in time. Their approximations can get better as they add more grids — akin to adding more pixels to a high-definition television.
These approximations allow researchers to calculate the masses and angular momenta of merging black holes or neutron stars based on the gravitational-wave signals detected by the LIGO and Virgo observatories. According to Vijay Varma, a physicist at the Max Planck Institute for Gravitational Physics in Potsdam, Germany, and a member of the LIGO collaboration, current observations of gravitational waves are not accurate enough for the subtle differences caused by supertranslations to be noticeable. “But when the accuracy of our observations gets 10 times better, those considerations will become more important,” Varma said. He pointed out that improvements of that order could be realized as soon as the 2030s.
Flanagan has a different perspective, maintaining that supertranslations are “not a problem that needs to be fixed” but rather are inevitable properties of angular momentum in general relativity that we need to live with.
The physicist Robert Wald, a general relativity specialist at the University of Chicago, shares Flanagan’s viewpoint to some extent, saying that supertranslations are more of an “inconvenience” than an actual problem. Nevertheless, he has reviewed the Chen, Wang, Wang and Yau paper carefully and concludes that the proof holds up well. “It really is resolving the supertranslation ambiguity,” Wald said, adding, “In general relativity, when you have all these alternative definitions to choose from,” it is nice to have a “unique choice” to pick out.
Yau, who has been working on defining these quantities since the 1970s, takes the long view. “It can take a long time for ideas from mathematics to permeate physics,” he said. He noted that even if the new definition of angular momentum goes unused for now, scientists at LIGO and Virgo “are always calculating something approximately. But ultimately, it’s good to know what the thing is that you’re trying to approximate.”
Editor’s note: Po-Ning Chen receives funding from the Simons Foundation, which also supports this editorially independent magazine.