New Black Hole Math Closes Cosmic Blind Spot
Introduction
Last year, just for the heck of it, Scott Field and Gaurav Khanna tried something that wasn’t supposed to work. The fact that it actually worked quite well is already starting to make some ripples.
Field and Khanna are researchers who try to figure out what black hole collisions should look like. These violent events don’t produce flashes of light, but rather the faint vibrations of gravitational waves, the quivers of space-time itself. But observing them is not as simple as sitting back and waiting for space to ring like a bell. To pick out such signals, researchers must constantly compare the data from gravitational wave detectors to the output of various mathematical models — calculations that reveal the potential signatures of a black hole collision. Without reliable models, astronomers wouldn’t have a clue what to look for.
The trouble is, the most trustworthy models come from Einstein’s general theory of relativity, which is described by 10 interlinked equations that are notoriously difficult to solve. To chronicle the complex interactions between colliding black holes, you can’t just use a pen and paper. The first so-called numerical relativity solutions to the Einstein equations for the case of a black hole merger were calculated only in 2005 — after decades of attempts. They required a supercomputer running on and off for two months.
A gravitational wave observatory like LIGO needs to have a large number of solutions to draw upon. In a perfect world, physicists could just run their model for every possible merger permutation — a black hole with a certain mass and spin encountering another with a different mass and spin — and compare those results with what the detector sees. But the calculations take a long time. “If you give me a big enough computer and enough time, you can model almost anything,” said Scott Hughes, a physicist at the Massachusetts Institute of Technology. “But there’s a practical issue. The amount of computer time is really exorbitant” — weeks or months on a supercomputer. And if those black holes are unevenly sized? The calculations would take so long that researchers consider the task practically impossible. Because of that, physicists are effectively unable to spot collisions between black holes with mass ratios greater than 10-to-1.
Which is one reason why Field and Khanna’s new work is so exciting. Field, a mathematician at the University of Massachusetts, Dartmouth, and Khanna, a physicist at the University of Rhode Island, have made an assumption that simplifies matters greatly: They treat the smaller black hole as a “point particle” — a speck of dust, an object with mass but zero radius and no event horizon.


Scott Field (top) and Gaurav Khanna did not expect their approximation to work for black holes of relatively equal masses.
Scott Field (left) and Gaurav Khanna did not expect their approximation to work for black holes of relatively equal masses.
Courtesy of Scott Field; Courtesy of Gaurav Khanna
“It’s like two ships passing in the ocean — one a rowboat, the other a cruise liner,” Field explained. “You wouldn’t expect the rowboat to affect the cruise liner’s trajectory in any way. We’re saying the small ship, the rowboat, can be completely ignored in this transaction.”
They expected it to work when the smaller black hole’s mass really was like a rowboat’s compared to a cruise ship’s. “If the mass ratio is on the order of 10,000-to-1, we feel very confident in making that approximation,” Khanna said.
But in research published last year, he and Field, along with graduate student Nur Rifat and Cornell physicist Vijay Varma, decided to test their model at mass ratios all the way down to 3-to-1 — a ratio so low it had never been tried, mainly because no one considered it worth trying. They found that even at this low extreme, their model agreed, to within about 1%, with results obtained by solving the full set of Einstein’s equations — an astounding level of accuracy.
“That’s when I really started to pay attention,” said Hughes. Their results at mass ratio 3, he added, were “pretty incredible.”
“It’s an important result,” said Niels Warburton, a physicist at University College Dublin who was not involved with the research.
The success of Field and Khanna’s model down to ratios of 3-to-1 gives researchers that much more confidence in using it at ratios of 10-to-1 and above. The hope is that this model, or one like it, could operate in regimes where numerical relativity cannot, allowing researchers to scrutinize a part of the universe that has been largely impenetrable.
How to Find a Black Hole
After black holes spiral toward each other and collide, the massive bodies create space-time-contorting disturbances — gravitational waves — that propagate through the universe. Eventually, some of these gravitational waves might reach Earth, where the LIGO and Virgo observatories wait. These enormous L-shaped detectors can sense the truly tiny stretching or squishing of space-time that these waves create — a shift 10,000 times smaller than the width of a proton.
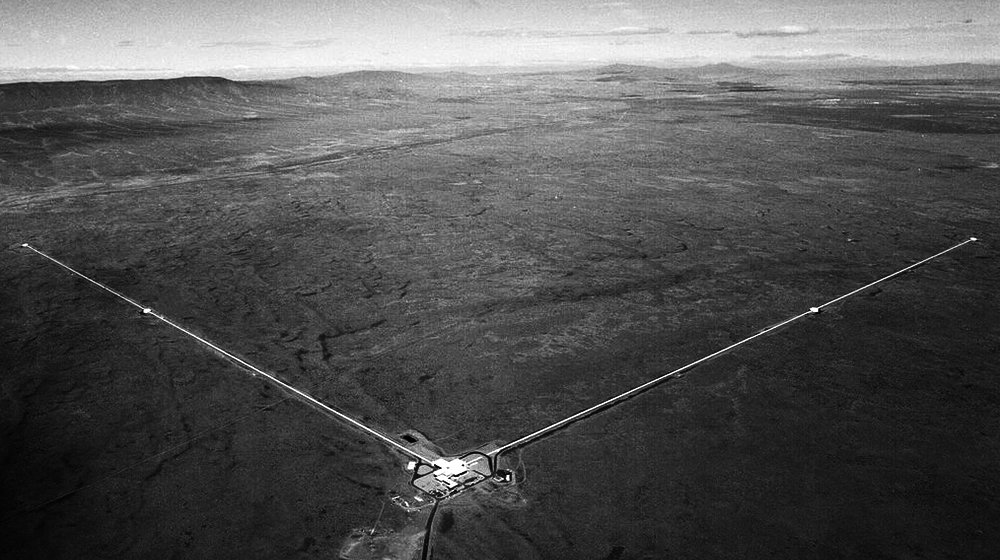
The LIGO detector in Hanford, Washington, has two long arms arranged at right angles. Lasers inside each arm measure the relative difference in length of each arm as a gravitational wave goes by.
LIGO
The designers of these observatories have made herculean efforts to muffle stray noise, but when your signal is so weak, noise is a constant companion.
The first task in any gravitational wave detection is to try to extract a weak signal from that noise. Field compares the process to “driving in a car with a loud muffler and a lot of static on the radio, while thinking there might be a song, a faint melody, somewhere in that noisy background.”
Astronomers take the incoming stream of data and first ask if any of it is consistent with a previously modeled gravitational wave form. They might run this preliminary comparison against tens of thousands of signals stored in their “template bank.” Researchers can’t determine the exact black hole characteristics from this procedure. They’re just trying to figure out if there’s a song on the radio.
The next step is analogous to identifying the song and determining who sang it and what instruments are playing. Researchers run tens of millions of simulations to compare the observed signal, or wave form, with those produced by black holes of differing masses and spins. This is where researchers can really nail down the details. The frequency of the gravitational wave tells you the total mass of the system. How that frequency changes over time reveals the mass ratio, and thus the masses of the individual black holes. The rate of change in the frequency also provides information about a black hole’s spin. Finally, the amplitude (or height) of the detected wave can reveal how far the system is from our telescopes on Earth.
If you have to do tens of millions of simulations, they’d better be quick. “To complete that in a day, you need to do each in about a millisecond,” said Rory Smith, an astronomer at Monash University and a member of the LIGO collaboration. Yet the time needed to run a single numerical relativity simulation — one that faithfully grinds its way through the Einstein equations — is measured in days, weeks or even months.
To speed up this process, researchers typically start with the results of full supercomputer simulations — of which several thousand have been carried out so far. They then use machine learning strategies to interpolate their data, Smith said, “filling in the gaps and mapping out the full space of possible simulations.”
This “surrogate modeling” approach works well so long as the interpolated data doesn’t stray too far from the baseline simulations. But simulations for collisions with a high mass ratio are incredibly difficult. “The bigger the mass ratio, the more slowly the system of two inspiraling black holes takes to evolve,” Warburton explained. For a typical low-mass-ratio computation, you need to look at 20 to 40 orbits before the black holes plunge together, he said. “For a mass ratio of 1,000, you need to look at 1,000 orbits, and that would just take too long” — on the order of years. This makes the task virtually “impossible, even if you have a supercomputer at your disposal,” Field said. “And without a revolutionary breakthrough, this won’t be possible in the near future either.”
Because of this, many of the full simulations used in surrogate modeling are between the mass ratios of 1 and 4; almost all are less than 10. When LIGO and Virgo detected a merger with a mass ratio of 9 in 2019, it was right at the limit of their sensitivity. More events like this haven’t been found, Khanna explained, because “we don’t have reliable models from supercomputers for mass ratios above 10. We haven’t been looking because we don’t have the templates.”
That’s where the model that he and Khanna have developed comes in. They started with their own point particle approximation model, which is specially designed to operate in the mass ratio range above 10. They then trained a surrogate model on it. The work opens up opportunities to detect the mergers of unevenly sized black holes.
What kinds of situations might create such mergers? Researchers aren’t sure, since this is a newly opening frontier of the universe. But there are a few possibilities.
First, astronomers can imagine an intermediate-mass black hole of perhaps 80 or 100 solar masses colliding with a smaller, stellar-size black hole of about 5 solar masses.
Another possibility would involve a collision between a garden-variety stellar black hole and a relatively puny black hole left over from the Big Bang — a “primordial” black hole. These could have as little as 1% of a solar mass, whereas the vast majority of black holes detected by LIGO so far weigh more than 10 solar masses.
Earlier this year, researchers at the Max Planck Institute for Gravitational Physics used Field and Khanna’s surrogate model to look through LIGO data for signs of gravitational waves emanating from mergers involving primordial black holes. And while they didn’t find any, they were able to place more precise limits on the possible abundance of this hypothetical class of black holes.
Furthermore, LISA, a planned space-based gravitational wave observatory, might one day be able to witness mergers between ordinary black holes and the supermassive varieties at the centers of galaxies — some with the mass of a billion or more suns. LISA’s future is uncertain; its earliest launch date is 2035, and its funding situation is still unclear. But if and when it does launch, we may see mergers at mass ratios above 1 million.
The Breaking Point
Some in the field, including Hughes, have described the new model’s success as “the unreasonable effectiveness of point particle approximations,” underscoring the fact that the model’s effectiveness at low mass ratios poses a genuine mystery. Why should researchers be able to ignore the critical details of the smaller black hole and still arrive at the right answer?
“It’s telling us something about the underlying physics,” Khanna said, though exactly what that is remains a source of curiosity. “We don’t have to concern ourselves with two objects surrounded by event horizons that can get distorted and interact with each other in strange ways.” But no one knows why.
In the absence of answers, Field and Khanna are trying to extend their model to more realistic situations. In a paper scheduled to be posted early this summer on the preprint server arxiv.org, the researchers give the larger black hole some spin, which is expected in an astrophysically realistic situation. Again, their model closely matches the findings of numerical relativity simulations at mass ratios down to 3.
They next plan to consider black holes that approach each other on elliptical rather than perfectly circular orbits. They’re also planning, in concert with Hughes, to introduce the notion of “misaligned orbits” — cases in which the black holes are askew relative to each other, orbiting in different geometric planes.
Finally, they’re hoping to learn from their model by trying to make it break. Could it work at a mass ratio of 2 or lower? Field and Khanna want to find out. “One gains confidence in an approximation method when one sees it fail,” said Richard Price, a physicist at MIT. “When you do an approximation that gets surprisingly good results, you wonder if you are somehow cheating, unconsciously using a result that you shouldn’t have access to.” If Field and Khanna push their model to the breaking point, he added, “then you’d really know that what you are doing is not cheating — that you just have an approximation that works better than you’d expect.”
Correction May 19, 2021:
An earlier caption in this article mislabeled the gravitational-wave signals from the LIGO detectors in Hanford, Washington and Livingston, Louisiana.