New Proof Finds the ‘Ultimate Instability’ in a Solar System Model

Harol Bustos for Quanta Magazine
Introduction
In 2009, a pair of astronomers at the Paris Observatory announced a startling discovery. After building a detailed computational model of our solar system, they ran thousands of numerical simulations, projecting the motions of the planets billions of years into the future. In most of those simulations — which varied Mercury’s starting point over a range of just under 1 meter — everything proceeded as expected. The planets continued to revolve around the sun, tracing out ellipse-shaped orbits that looked more or less the way they have throughout human history.
But around 1% of the time, things went sideways — quite literally. The shape of Mercury’s orbit changed significantly. Its elliptical trajectory gradually flattened, until the planet either plummeted into the sun or collided with Venus. Sometimes, as it cut its new path through space, its behavior destabilized other planets as well: Mars, for instance, might be ejected from the solar system, or it might crash into Earth. Venus and Earth could, in a slow, cosmic dance, exchange orbits several times before eventually colliding.
Perhaps the solar system was not as stable as people once thought.
For centuries, ever since Isaac Newton formulated his laws of motion and gravity, mathematicians and astronomers have grappled with this issue. In the simplest model of the solar system, which considers only the gravitational forces exerted by the sun, the planets follow their elliptical orbits like clockwork for eternity. “It’s kind of a comforting picture,” said Richard Moeckel, a mathematician at the University of Minnesota. “It’s going to go on forever, and we’ll be long gone, but Jupiter will still be going around.”
But once you account for gravitational attraction between the planets themselves, everything gets more complicated. You can no longer explicitly calculate the planets’ positions and velocities over long periods of time, and must instead ask qualitative questions about how they might behave. Might the effects of the planets’ mutual attraction accumulate and break the clockwork?
Detailed numerical simulations, like those published by the Paris Observatory’s Jacques Laskar and Mickaël Gastineau in 2009, suggest that there’s a small but real chance of things going haywire. But those simulations, though important, aren’t the same as a mathematical proof. They can’t be completely precise, and as the simulations themselves show, a small imprecision might — over the course of billions of simulated years — lead to very different outcomes. Furthermore, they don’t provide an underlying explanation for why certain events might unfold. “You want to understand what mathematical mechanisms drive instabilities, and to prove that they actually exist,” said Marcel Guàrdia, a mathematician at the University of Barcelona.

The mathematicians Marcel Guàrdia (left) and Jacques Fejoz have been collaborating for years in pursuit of a proof that instability can arise in a model solar system.
Jessica Massetti
Now, in three papers that together exceed 150 pages, Guàrdia and two collaborators have proved for the first time that instability inevitably arises in a model of planets orbiting a sun.
“The result is really very spectacular,” said Gabriella Pinzari, a mathematical physicist at the University of Padua in Italy. “The authors proved a theorem that is one of the most beautiful theorems that one could prove.” It could also help explain why our solar system looks the way it does.
Four Pages and a New Story
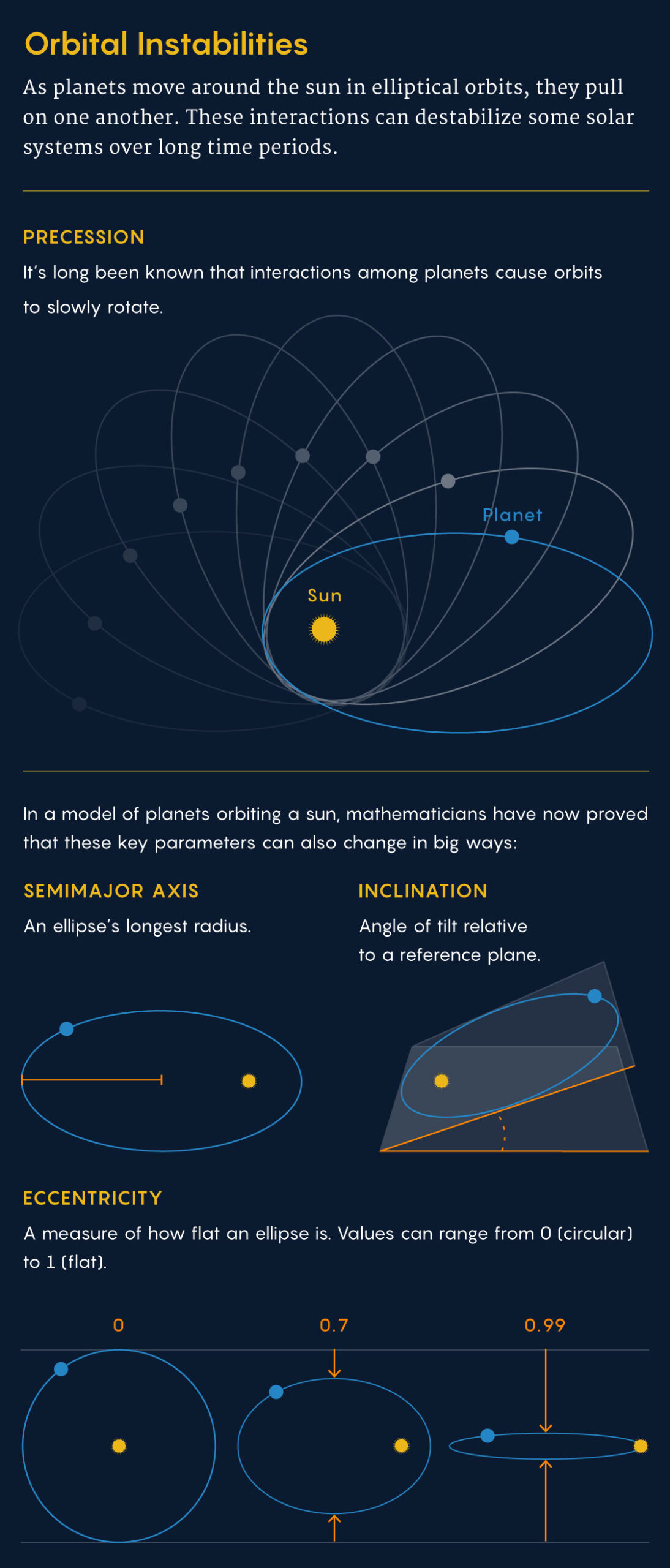
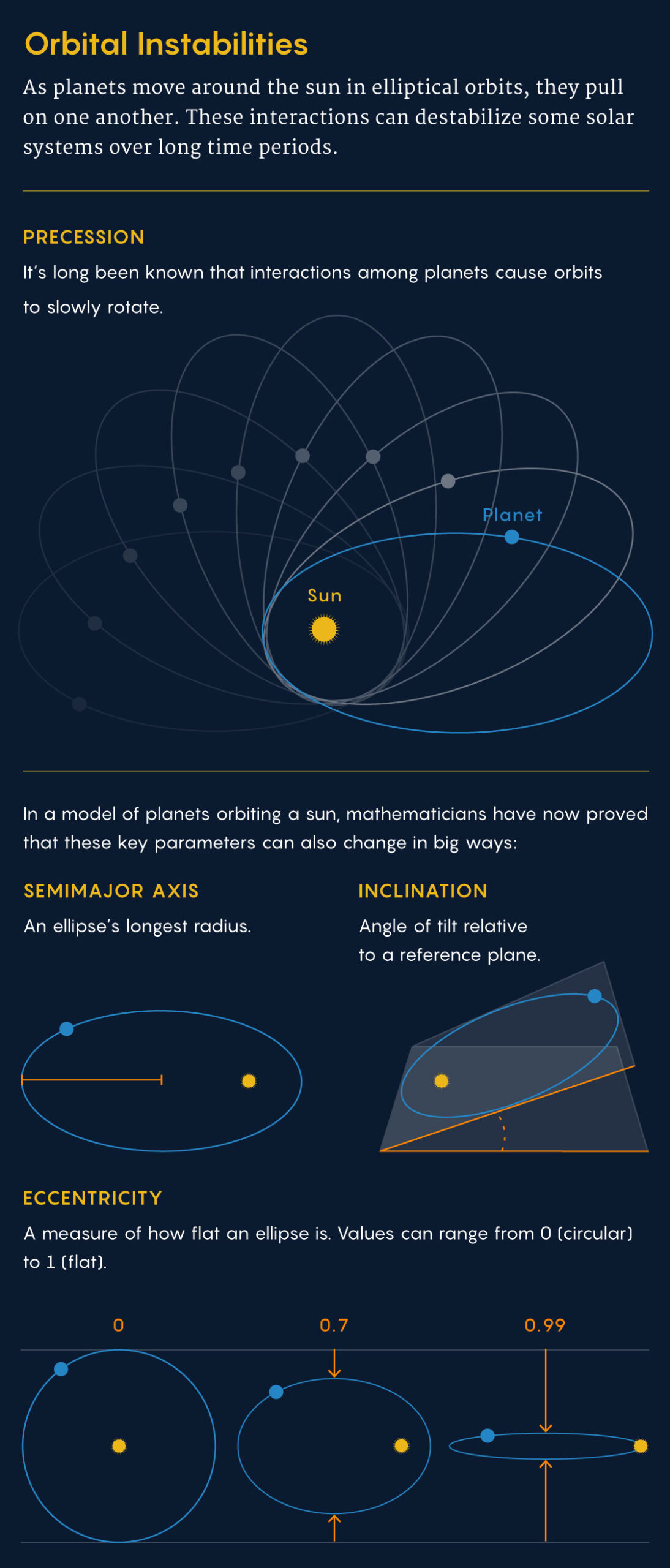
Centuries ago, it was already clear that interactions among the planets could have long-term effects. Consider Mercury. It takes approximately three months to travel around the sun on an elliptical path. But that path also slowly rotates — one degree every 600 years, a full rotation every 200,000. This kind of rotation, known as precession, is largely a result of Venus, Earth and Jupiter pulling on Mercury.
But research in the 18th century by mathematical giants like Pierre-Simon Laplace and Joseph-Louis Lagrange indicated that, precession aside, the size and shape of the ellipse are stable. It wasn’t until the late 19th century that this intuition started to shift, when Henri Poincaré found that even in a model with just three bodies (say, a star orbited by two planets), it’s impossible to compute exact solutions to Newton’s equations. “Celestial mechanics is a delicate thing,” said Rafael de la Llave, a mathematician at the Georgia Institute of Technology. Alter the initial conditions by a hair — for example, by shifting the assumed position of one planet by a mere meter, as Laskar and Gastineau did in their simulations — and over long timescales the system can look very different.
In the three-body problem, Poincaré found a tangle of possible behaviors so complicated that at first he thought he’d made a mistake. Once he accepted the truth of his results, it was no longer possible to take the solar system’s stability for granted. But because working with Newton’s equations is so difficult, it wasn’t clear if the behavior of the solar system might be complicated and chaotic only on a small scale — planets might end up in different positions within a predictable band, for instance — or if, as Guàrdia and his collaborators would eventually prove in their own model, the size and shape of orbits might change so much that planets could conceivably crash into each other or travel off to infinity.
Merrill Sherman/Quanta Magazine
Then, in 1964, the mathematician Vladimir Arnold wrote a four-page paper that established the right language for framing the problem. He found a specific reason why key variables in a dynamical system might change in a big way. First, he cooked up an artificial example, a strange blend of a pendulum and a rotor that didn’t remotely resemble anything you’d encounter in nature. In this toy model, he proved that, given enough time, certain quantities that usually stay constant can change by large amounts.
Arnold then conjectured that most dynamical systems should exhibit this kind of instability. In the case of the solar system, this might mean that the orbital shapes, or eccentricities, of certain planets could potentially shift over billions of years.
But while mathematicians and physicists eventually made a lot of progress on proving that instability arises in general, they struggled to show it for celestial models. That’s because the gravitational effect of the sun is so overwhelmingly strong that many features of the clockwork planetary model persist even when you consider the additional forces exerted by the planets. (In this context, Newtonian mechanics gives such a good approximation of reality that these models don’t need to consider the effects of general relativity.) Such inherent stability makes instability difficult to detect.
Could parameters that stayed so stable in computations done by Laplace, Lagrange and others really change significantly? “You have to handle an instability which is extremely weak,” said Laurent Niederman of Paris-Saclay University. The usual methods won’t catch it.
Numerical simulations offered hope that the hunt for such a proof was not in vain. And there were preliminary proofs. In 2016, for instance, de la Llave and two colleagues proved instability in a simplified celestial mechanics model consisting of a sun, a planet and a comet, where the comet was assumed to have no mass and therefore no gravitational effect on the planet. This setup is known as a “restricted” n-body problem.
The new papers tackle a true n-body problem — showing that instability arises in a planetary system where three small bodies revolve around a much larger sun. Even though the size and shape of the orbits might spend a long time oscillating around fixed values, they will eventually change dramatically.
This had been expected — it was widely believed that stability and instability coexist in this kind of model — but the mathematicians were the first to prove it.
The Ultimate Instability
Together with Jacques Fejoz of the University of Paris Dauphine, Guàrdia first attempted to prove instability in the three-body problem (one sun, two planets) in 2016. Though they were able to show that chaotic dynamics arose in the flavor of Poincaré, they couldn’t prove that this chaotic behavior corresponded to large and long-term changes.
Andrew Clarke, a postdoc studying under Guàrdia, joined them in September 2020, and they decided to give the problem another go, this time adding an extra planet to the mix. In their model, three planets revolve around a sun at increasingly large distances from each other. Crucially, the innermost planet starts out orbiting at a significant tilt relative to the second and third planets, so that its path practically forms a right angle to theirs.

The mathematician Andrew Clarke wakes up in the middle of the night, wondering if our solar system shows the same instabilities as the models that he studies.
Frith Carlisle
This inclination allowed the mathematicians to find initial conditions that result in instability.
They showed the existence of trajectories that led to pretty much any possible eccentricity for the second planet: Over time, it was possible for its ellipse to flatten until it almost looked like a straight line. Meanwhile, the orbits of the second and third planets, which had started out in the same plane, could also end up perpendicular to each other. The second planet could even flip a full 180 degrees, so that while all the planets might at first have moved clockwise around the sun, the second one ended up moving counterclockwise. “Imagine that you look forward a million years, and Mars is going the opposite way,” said Richard Montgomery of the University of California, Santa Cruz. “That would be weird.”
“You cannot avoid very wild orbits, even in this simple setting,” Niederman said.
Even so, the sizes of the orbits stayed stable. That’s because in this model, the planets move around the sun very quickly compared to how long it takes for their orbits to precess — allowing the mathematicians to gloss over the “fast” variables related to the planets’ motions. “It’s tedious to think about what’s happening every year if what you’re really interested in is what’s happening over a thousand years,” Moeckel said. Oscillations in the size of each ellipse (measured in terms of its long radius, or semimajor axis) average out.
This wasn’t surprising. “Common knowledge says that the inclination and the eccentricity should be more unstable than the semimajor axis,” Guàrdia said. But then he and his colleagues realized that if they placed the third planet even farther away from the sun, they might be able to add more instability into their model.
This new system and the equations that governed it were more complicated, and the mathematicians weren’t certain they’d be able to get any results. But “it was too much to ignore,” Clarke said. “If there was a chance of showing semimajor axes could drift, then I mean, you have to pursue that.”
Laskar, who has led much of the numerical work on instability in the solar system, said that if you superimposed this kind of solar system on our own, you might see the first planet nestled right up against the sun, the second planet where Earth would be, and the third planet all the way out at the Oort Cloud, at our solar system’s outer limits. (As a result, he added, this represents a “very extreme situation” — one he doesn’t necessarily expect to find in our own galaxy.)
The greater a planet’s distance from the sun, the longer it takes to complete an orbit. In this case, the third planet is so far away that the precession of the two inner planets occurs at a faster rate. It is no longer possible to average out the motion of the last planet — a scenario Lagrange and Laplace didn’t consider in their accounts of the solar system’s stability. “This will change completely the structure of the equation,” said Alain Chenciner, a mathematician also at the Paris Observatory. There were now more variables to worry about.
Clarke, Fejoz and Guàrdia proved that the orbits can grow arbitrarily large. “They finally get the size of the orbit to increase, as opposed to just the shape or something like that,” Moeckel said. “That’s the ultimate instability.”
Even though these changes accumulated very slowly, they still occurred more quickly than one might have expected — suggesting that in a realistic planetary system, changes might accumulate over hundreds of millions of years, rather than billions.
In 2009, the mathematical physicist Gabriella Pinzari independently rediscovered a complicated coordinate system that had been forgotten for decades, making new work on planetary instability possible.
Department of Mathematics of University of Padua
The results provide a potential explanation for why the planets in our solar system have orbits that all lie nearly in the same plane. It shows that something as simple as a large angle of inclination can be a source of a great deal of instability, on multiple counts. “If you start with a situation where the mutual inclinations are quite big, then you will destroy the system quite ‘quickly,’” Chenciner said. “It would have been destroyed hundreds, thousands of centuries ago.”
High-Dimensional Highways
These proofs required a clever combination of techniques from geometry, analysis and dynamics — and a return to basic definitions.
The mathematicians represented each configuration of their planetary system (the positions and velocities of the planets) as a point in a high-dimensional space. Their goal was to show the existence of “highways” through the space that correspond to, say, large changes in the second planet’s eccentricity, or in the third planet’s semimajor axis.
To do that, they first had to express each point in terms of coordinates that were so esoteric and complex that hardly anyone had even heard of them, let alone tried to use them. (The coordinates were discovered in the early 1980s by the Belgian astronomer André Deprit, then forgotten and later independently discovered by Pinzari in 2009 while she was working on her doctoral thesis. They’ve barely been used since.)
By using Deprit’s coordinates to describe their high-dimensional space of planetary configurations, the mathematicians gained a deeper understanding of its structure. “That’s part of the beauty of the proof: to manage to deal with this 18-dimensional geometry,” Fejoz said.
Fejoz, Clarke and Guàrdia found highways that traversed several special regions in that space. They then used their newfound geometric understanding to prove that the highways corresponded to unstable dynamics in the size and shape of the planets’ orbits.
“When I finished my Ph.D. 30 years ago,” Niederman said, “we were extremely, extremely far from these kinds of results.”
“It’s such a complicated system that you have this feeling that anything that is not obviously forbidden should happen,” Chenciner said. “But it’s usually very hard to prove it.”
Mathematicians now hope to use Clarke, Fejoz and Guàrdia’s techniques to prove instability in models that look more like our own solar system. These kinds of results are becoming particularly meaningful as astronomers uncover more and more exoplanets orbiting other stars, showcasing a broad range of configurations. “It’s like an open lab,” said Marian Gidea, a mathematician at Yeshiva University. “To understand on paper what types of evolutions of planetary systems can happen, and to compare that with what you are able to observe — it is very exciting. It gives a lot of information about the physics of our universe, and about how much of this our mathematics is able to capture through relatively simple models.”
In hopes of making such a comparison, Fejoz has been speaking with a couple of astronomers about identifying extrasolar systems that resemble, even loosely, the model he and his colleagues developed. Other researchers, including Gidea, say that the work could be useful for designing efficient trajectories for artificial satellites, or for figuring out how to move particles at high speeds through a particle accelerator. As Pinzari said, “Research in celestial mechanics is still very much alive.”
The ultimate goal would be to prove instability in our own solar system. “I wake up in the middle of the night thinking about it,” Clarke said. “I would say that would be the real dream, but it would be a nightmare, wouldn’t it? Because we’d be screwed.”
Correction: May 16, 2023
This article was revised to reflect that Marcel Guàrdia is a professor at the University of Barcelona. He moved from the Polytechnic University of Catalonia in the summer of 2022.