Physicists Puzzle Over Emergence of Strange Electron Aggregates

Nash Weerasekera for Quanta Magazine
Introduction
In the 127 years since the electron was discovered, it has undergone more scrutiny than perhaps any other particle. As a result, its properties are not just well known, but rote, textbook material: Electrons have a smidgen of mass and negative electric charge. In a conductor, they swim relatively unimpeded as a current; in an insulator, they barely move.
Over time, caveats have cropped up. Under an intense magnetic field, for example, electrons can lose their individual identities and form “quasiparticles”: collective entities, like the shape formed by a school of fish. But even these collective states have been well cataloged.
So it came as a shock last year when a new effect was seen in electrons. Researchers at the University of Washington reported in August 2023 that in a stack of two atomically thin crystalline sheets offset from each other at a slight angle, electrons behaved like quasiparticles with fractional amounts of charge, such as $latex -\frac{2}{3}$ and $latex -\frac{3}{5}$. A few months later, a team at the Massachusetts Institute of Technology reported the same effect in another material. It was the first time that electrons had formed fractional quasiparticles without the enabling influence of a magnetic field.
While predictions about the possibility of this particular effect date back to 2011, theorists are still puzzling over the new discovery. It’s not clear how the underlying mechanism works in the MIT group’s material; calculations from several groups neither fully explain the fractional states nor agree. Other, even odder quantum phases of matter may also be present.
The new discovery isn’t incidental, or specific to a material. Rather, it’s universal and fundamental — the result of the quantum nature of the electron, albeit a behavior that has until now stayed hidden. While condensed matter physicists want to understand the breadth of electron behaviors for their own sake, there’s always the chance of uncovering the basis of a world-changing technology. In this case, the newfound effect may carry the seeds of long-sought quasiparticles with stable memories that could underpin a new and powerful approach to quantum computing.
Outlandish Fractions
The story that led to last year’s discoveries began back in 1879, when Edwin Hall, a graduate student at Johns Hopkins University, applied a magnetic field vertically through a flat metal ribbon with a current flowing along it. The field pushed the current of electrons to one edge of the ribbon. The resulting sideways current and the associated sideways resistance were later named after Hall.
A century later, the German physicist Klaus von Klitzing investigated the Hall effect in a sheet of ultracold, pure gallium arsenide. As he cranked up the magnetic field, von Klitzing expected to see the Hall resistance increase steadily. But instead of growing continuously, the Hall resistance would lie flat and then jump up, forming a staircase of discrete steps. Even odder, the plateaus were found at integer multiples of the square of a fundamental constant: e, the charge of the electron.

After Klaus von Klitzing (center) discovered the quantum Hall effect, Horst Störmer (left) co-discovered a fractional version of the effect and the theorist Robert Laughlin (right) helped explain why the latter arises.
AIP Emilio Segrè Visual Archives, Lockwood Collection, Gift of David J. Lockwood, Photo by Harry Turner of the National Research Council Canada
Why the strange plateaus? Researchers realized that the Hall resistance could not change smoothly because the strong magnetic field was pushing the electrons’ energy levels — essentially the speeds they’re allowed to have — far apart. That separation meant that slightly increasing the magnetic field did nothing; electrons still had to maintain the same speed, and therefore experience the same resistance, as before. Only large increases of the magnetic field could get the electrons to jump to the next energy level.
The plot thickened in 1982, when three physicists at Bell Labs spotted a dazzling new series of resistance plateaus, this time at fractional values of the square of the electron’s charge. Horst Störmer, one of the discoverers, described the oddness of the fractions in his Nobel lecture after sharing the prize for the work: “Many electrons, acting in concert, can create new particles having a charge smaller than the charge of any individual electron. This is not the way things are supposed to be.” Störmer and his colleagues had used the same material, in a similar experimental setup, using, of course, the same indivisible electrons — so where had the outlandish fractions come from?
Theorists attribute them to the delicate web of repulsive interactions between each electron in the material and every other. These interactions are “the missing ingredient,” said Jennifer Cano, a condensed matter theorist at Stony Brook University. The interacting electrons, poised just so, are able to gather strands of the magnetic field to form new quasiparticles called composite fermions. While individual electrons occupy energy levels of the integer n, composite fermions occupy energy levels that follow the rule n/(2n + 1), yielding a series of fractions. Another interpretation is that the fractional quantum Hall effect is like the integer quantum Hall effect, but for quasiparticles that carry fractional charges.
Dance of Electrons
Both the integer and fractional quantum Hall effects appeared in the presence of a strong magnetic field. But, physicists wondered, was the field essential? In the late ’80s, the theorist Duncan Haldane, then at the University of California, San Diego, predicted that it might even be possible to see integer plateaus without the aid of an external magnetic field — a so-called quantum anomalous Hall effect. (Here, “anomalous” just means “happening without an external magnetic field.”) Haldane imagined a 2D hexagonal lattice of atoms in which electrons hopped back and forth, changing places with their next-to-nearest neighbors. The resulting electron dance acts like an internal magnetic field — a substitute for an external field that pushes electron energy levels apart.

Jennifer Cano, a condensed matter physicist at Stony Brook University, is one of the theorists trying to get a handle on the fractional anomalous quantum Hall effect.
Emily Tan/Simons Foundation
In 2012, a group of researchers at Tsinghua University in China created a metallic film from a mix of elements — bismuth, antimony and tellurium, with a dash of chromium to provide an effective internal magnetic field. When the Tsinghua group ran a current through the film, without any external magnetic field, the characteristic integer plateaus in the Hall resistance showed up.
Around the same time, three independent groups imagined ways to create a fractional quantum anomalous Hall effect, with a series of plateaus at fractions of the square of the electron’s charge. To exhibit it, a hypothetical material would need a powerful effective internal magnetic field. Additionally, it would need to support the delicate many-body interactions that lead electrons to dress themselves in the magnetic field and create composite fermions — stringent requirements. “I thought they’d never be realized,” said B. Andrei Bernevig, a condensed matter theorist at Princeton University who worked on one of the papers.
What they didn’t see coming was a whole new class of 2D materials.
Moiré Magic
In 2004, after decades of trying, physicists managed to isolate a sheet of graphene — a hexagonal lattice of carbon atoms — using a novel exfoliation procedure: They used Scotch tape to peel the sheet off a slab of graphite. 2D materials like graphene soon showed promise as platforms for interesting electron behaviors. Just a few years later, physicists put room-temperature graphene under a powerful magnetic field and saw the integer quantum Hall effect.
But it was “moiré materials” that cemented 2D materials as the platform of choice. Pronounced either mwah-ray or mor-ay, the word traditionally refers to the rippling pattern created by overlaying two sheets of fabric, such as silk. Physicists have borrowed the term to describe the patterns created when overlaid sheets of atoms are offset by an angle, or slightly mismatched in size.
Compared to conventional 3D materials, 2D moirés are extremely customizable. Tiny variations in the systems can produce dramatic changes. For example, in 2017, researchers at MIT twisted two sheets of graphene at exactly 1.1 degrees. When cooled to a couple of degrees above absolute zero, the moiré material exhibited one of the most enticing quantum states: superconductivity, where electrons flow with zero resistance. Adjust the twist angle by half a degree and the superconductivity disappears. Almost overnight, many groups of researchers switched their efforts and began trying to make moiré materials.
“You’re pushing beyond what nature intended these crystals to look like by artificially assembling them,” said Matthew Yankowitz, a physicist at the University of Washington.
Some researchers, including Jie Shan and Kin Fai Mak, a pair of physicists at Cornell University, worked with 2D crystals called transition metal dichalcogenides (TMDs). These are semiconductors like silicon — ideal for investigating electronic behavior. When Shan and Mak created moirés with TMDs, interesting phenomena popped out. One TMD moiré exhibited the quantum anomalous Hall effect. But the effect’s fractional counterpart remained tantalizingly out of reach.
Fractions and Anomalies
A breakthrough came a year ago when a University of Washington team, led by Xiaodong Xu, managed to observe the fractional quantum anomalous Hall effect in a moiré material made of stacked and twisted layers of molybdenum ditelluride, a type of TMD. It was the first time that fractional charges had conclusively been observed without an external magnetic field. A few weeks after Xu’s team published in Nature, a group at Shanghai Jiao Tong University reported the same results in Physical Review X.
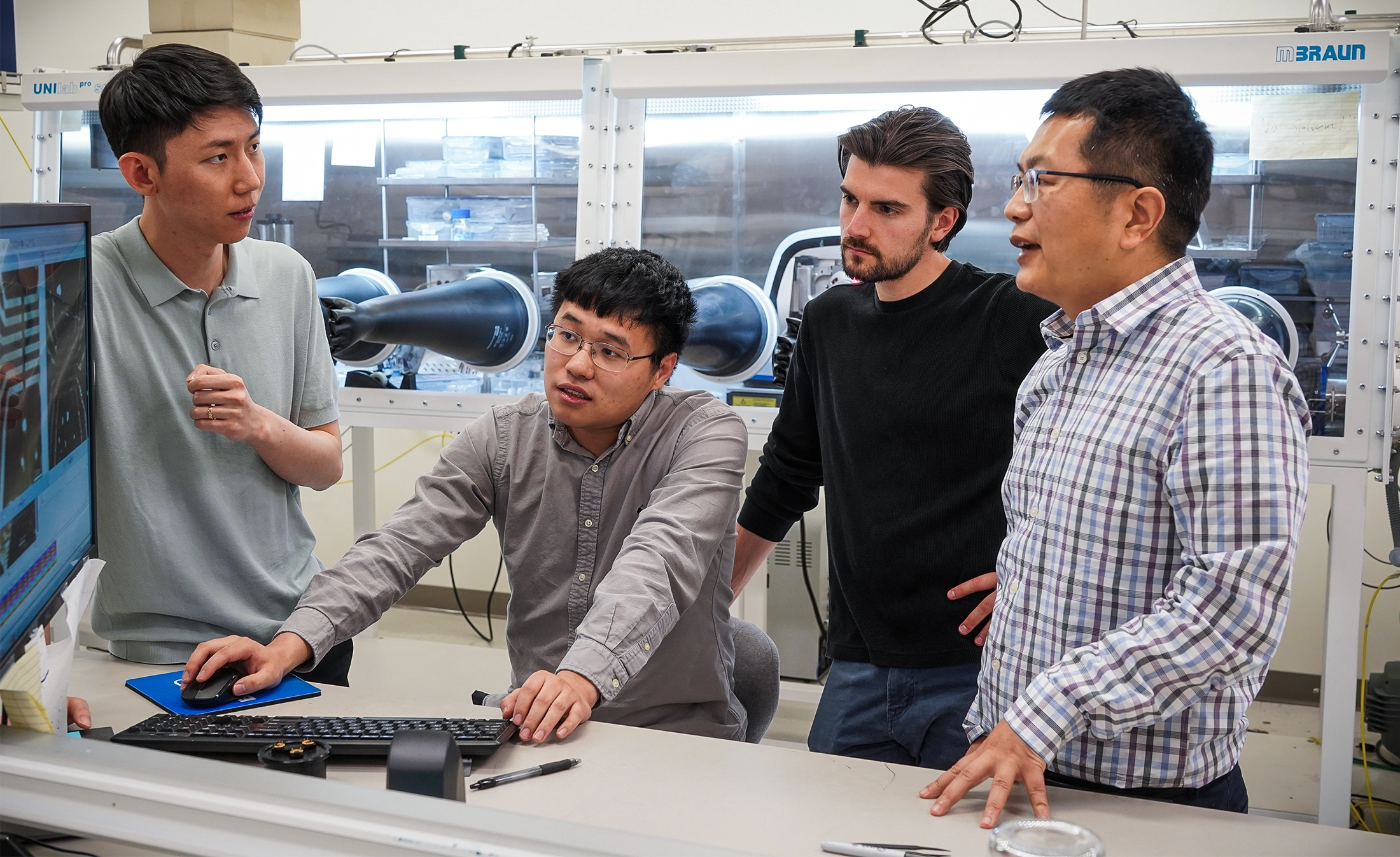
Xiaodong Xu (right) led a team at the University of Washington that discovered the fractional anomalous quantum Hall effect.
Courtesy of Xiaodong Xu
Long Ju, a physicist at MIT who specializes in 2D materials, was also in pursuit of the effect. Ju stuck five layers of carefully aligned graphene between boron nitride, cooled it down, and measured the resistance.
Again, the characteristic fractional plateaus appeared without any magnetic field. “It was a total surprise,” Ju said.
While some studies indicated that TMDs would exhibit the effect (at the right twist angle, the materials were expected to produce a powerful effective internal magnetic field), no theorist had predicted that the effect would show up in a material like Ju’s at MIT. The discovery has led to a good deal of head-scratching.
Cano, at Stony Brook, spent her graduate school years delightedly puzzling over the fractional quantum Hall effect, which has since been mostly resolved. “I thought that was in the rearview mirror,” she said. “It was very exciting to me when these kinds of developments in twisted TMDs and graphene came up.” Cano and other theorists are thinking about how to model the materials, but it’s not easy. “They buckle, they ripple, they strain, they distort, and so it’s actually very difficult to make a quantitatively correct model,” she said.
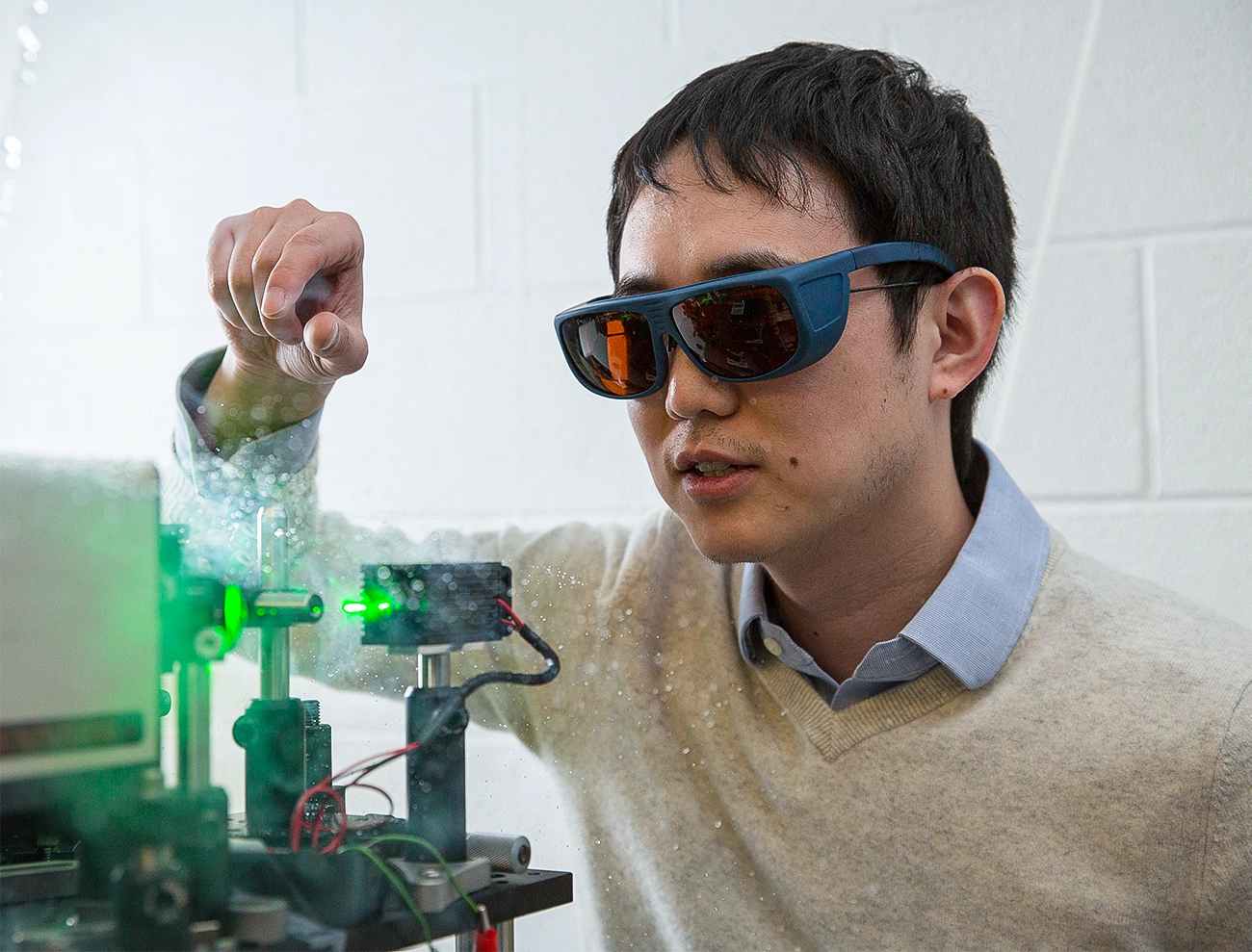
Long Ju in his lab at the Massachusetts Institute of Technology.
MIT
The difficulty, when it comes to understanding the fractional quantum anomalous Hall effect, is twofold. Theorists must first grapple with what is actually happening in the experimental samples. Then they have to consider: What can electrons do, in principle?
The exact role of the moiré pattern remains poorly understood. Some theorists initially wondered whether the moiré is needed at all. Perhaps the magic was all in the graphene. Unpublished results from Ju’s group have since shown that without the mismatched hexagonal boron nitride and graphene lattices, there are no fractional charges. “It’s clear that the moiré is important,” Bernevig said.
One possibility is that the layered graphene could be host to a so-called anomalous Hall crystal. Unlike a physical crystal made of a lattice of atoms, such as a diamond, an anomalous Hall crystal would be ephemeral, with a lattice composed of electrons arranged in a honeycomb. The electrons in such a crystal are stable, yet “still able to talk to each other,” as Cano puts it, and so their collective interactions can give rise to composite fermions with fractional charges.
Ju is currently creating more samples and attempting to measure them at lower temperatures, where there will be less thermal energy in the system, potentially allowing him to spot an anomalous Hall crystal. The work is ongoing, and “it’s still too early to draw any conclusion,” he said.
While most of the quasiparticles with fractional charges that arise in these systems are attributable to composite fermions, certain fractions with odd numerators, like the 5/2 and 7/2 states, may involve stranger quasiparticles called non-abelian anyons. Wind two of these around each other, and their states change, keeping a record of this winding. If these anyons could be isolated and “braided,” a controlled operation to wind their paths, they could form the ideal quantum bits needed for quantum computing, because the information encoded in them would be robust and stable.
The potential of non-abelian anyons for quantum computing has driven decades of research in academia and industry. While there have been hints — and retracted claims — no one has definitively seen the rare quasiparticles, let alone used them for computation. Researchers like Andrea Young, an experimentalist at the University of California, Santa Barbara, are placing their hope in moirés, which may offer the best chance yet to catch sight of their quantum quarry. Even in two dimensions, finding non-abelian anyons won’t be easy. “It’s a zoo,” Young said. “A lot can go on in these systems.”