Does Natural Law Need Elegant Mathematics?

Ever since I was very young, I have been enamored of elegant mathematics. Like many people of a similar bent, I agreed completely with Eugene Wigner’s famous article “The Unreasonable Effectiveness of Mathematics in the Natural Sciences,” in which the Nobel Prize-winning physicist discusses how elegant mathematics has been “unreasonably” successful in explaining physical law. Wigner further states: “The miracle of the appropriateness of the language of mathematics for the formulation of the laws of physics is a wonderful gift which we neither understand nor deserve.” Similar thoughts have been expressed by several famous scientists, including Albert Einstein.

Wigner’s statement is deeply poetic and born of wonder, but is it really true? Does this apparent connection between beautiful mathematics and natural science always hold? And if it does, is it really something that cannot be understood? One possible cause for skepticism is Wigner’s use of the word “miracle.” Used literally, “miracle” implies something magical or mystical, but Wigner was an eminent scientist — is that what he meant? The word “miracle” can also be used in a hyperbolic sense, as in the sentence, “What my smartphone can do is nothing short of a miracle.” What you really mean is that you know, deep down, that the things your smartphone can do have complex technical explanations, but the end result is simple and wondrous. As scientists, we must eschew the magical and try to unpack a more plausible explanation. Let’s try to do this in our new Insights puzzle.

Scenario 1: Simplicity and Uniformity

Part of Newton’s first law states that a body in motion remains in motion in a straight line at constant velocity. In mathematical terms, in the absence of any force, the object’s velocity (v) is constant, so its acceleration (expressed as dv/dt) is zero. The reason this elegant formulation took so long to be discovered is because it is an idealization in which all the elements have been reduced to the simplest form. It would only be absolutely true for the case of a hypothetical point particle that did not experience any forces of any kind. It’s certainly not absolutely true of any physical object on Earth — even an object sliding on a vast flat expanse of ice, though that is a good practical approximation. It would seem that simple assumptions give rise to simple math. So let’s consider two cases that are slightly more complicated:

A. An object slides on a uniform surface with initial velocity 1. For every 1 unit of distance, its velocity decreases by 1/10 of what it was at the start of that particular unit. How far will the object travel before it stops? What’s the general formula?

B. A powered vehicle can move forward or sideways with equal ease. Its normal cruising velocity in either direction is 1 unit on smooth ground. As shown in the figure, it has to navigate through 10 strips of terrain each 10 units long and 1 unit wide. The long axis of each strip is perpendicular to the direction that the vehicle needs to go. The vehicle is sitting near the middle of the first strip, which is smooth (shown gray in the figure). Thereafter uneven (purple) strips and smooth strips alternate.

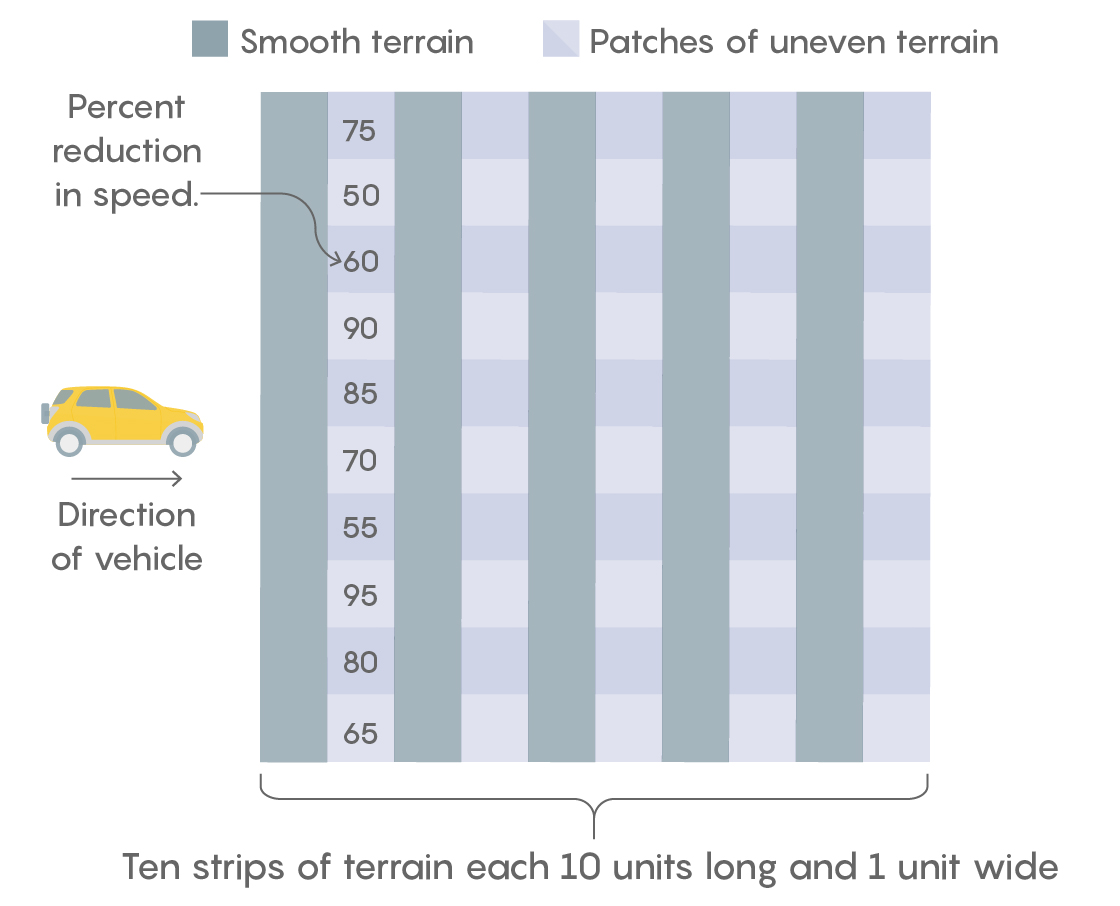
The uneven strips are not uniformly uneven, but consist of 10 square patches that we can think of as “speed bumps.” The speed bumps are stacked one on top of another, and each one is 1 unit by 1 unit in size. The speed bumps vary in how uneven they are. A speed bump can cause the vehicle’s cruising velocity to be reduced by any amount from 50% to 95% in 5% increments. All 10 types of speed bumps are present in every uneven strip, in a random arrangement (one possible arrangement of speed bumps is shown in the first purple strip in the figure). The vehicle can sense the roughness of the speed bump directly ahead of it and can move sideways at its cruising velocity of 1 in order to traverse a different speed bump that will slow it down less, if it so chooses. Of course, this will cost it time, and the time penalty will be greater if it moves sideways multiple blocks. After each strip, the cruising velocity returns to 1 on the intervening smooth strip. What strategy should the vehicle adopt to cross the terrain as fast as possible? How long will this be expected to take?

Note that both these cases are approximations with simplifications. The first case approximates a uniformly rough terrain, and the second case approximates a randomly rough terrain. Though we can use math to reason about them, we lose simplicity, elegance and predictive ability as the situations grow more complex. Such situations occur naturally in messier and more complex sciences like biology, psychology, sociology and human behavior. In such cases, we have to rely on large amounts of accurate data to detect trends and make probabilistic predictions — in the words of Peter Norvig, a research director at Google, and his colleagues, what we have here is the unreasonable effectiveness, not of math, but of data. Physics is generally simpler — and so the math remains tractable, and you can often make very accurate predictions. But even in physics, trying to describe the motion of multiple particles in a quantum or even classical system is hopelessly complex. Thus, the more complicated case B is somewhat reminiscent of, though nowhere near as complex as, the case of multiple particles interacting with each other in a quantum vacuum in which virtual particles are created and destroyed at random. In that situation, the math is likely to be full of nonlinearities and thus completely intractable.

Scenario 2: Summation and Symmetry

Why is it that simple idealized laws give fairly accurate results even in systems containing astronomical numbers of particles? Two reasons are summation and symmetry. We can see this by looking at the law of gravitational attraction: F = Gm₁m₂/r², where F is the gravitational force acting between two objects, m₁ and m₂ are the masses of the objects, r is the distance between the centers of their masses, and G is the gravitational constant. This inverse square law, which arises from geometry, is an idealization that strictly applies to nonphysical point masses. To show that this can be approximately applied to the planets, Newton did some hard, messy work. He showed that if the inverse square law applies to point particles, then it will work, approximately, for large, roughly spherically symmetrical objects as if all the mass is concentrated at the center of each object. The tiny particles that make up a real-world object are not located exactly at the object’s center but are close enough and symmetrical enough that the effects of their small deviations from the center on either side cancel each other out. So they summate to produce an effect that approximately follows the same formula, especially as distances between the objects get larger.

What happens if the objects are close to each other and not symmetrical? Will we still be able to apply the gravitational formula to the whole triangles as individual objects? Let’s find out.

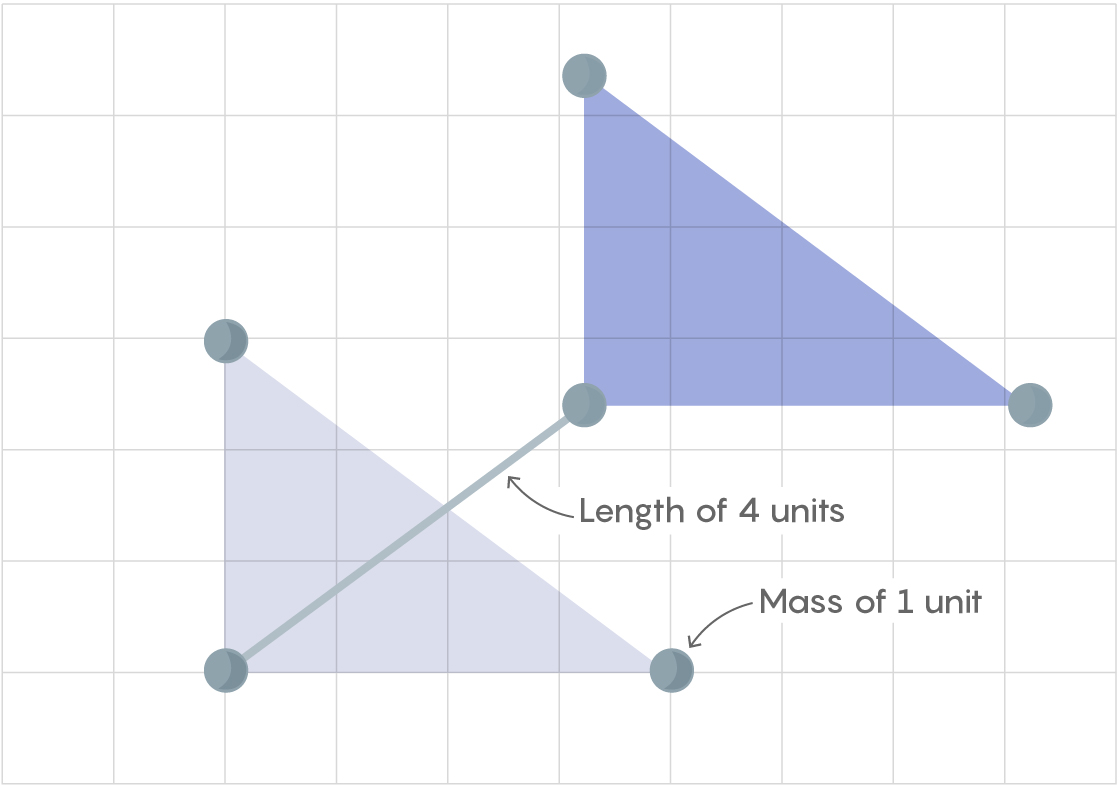
Consider a hypothetical rigid object that is shaped like a right triangle with virtually all of its mass concentrated at its vertices. For simplicity, imagine that the object is entirely two-dimensional — it has no thickness. Each vertex is a point mass of 1 unit, and the total mass is 3 units. The triangle has a base 4 units long, a vertical side 3 units long and a hypotenuse 5 units long going from upper left to lower right. Imagine there is a second such triangle in the same orientation, located as shown, such that the medians (lines drawn from the midpoint of the hypotenuse to the opposite vertex) of the two triangles lie on the same straight line, with the corresponding right-angle vertices 4 units apart. What would the magnitude of the gravitational force be? Does the law of gravitational attraction work if you try to apply it between the two triangles as individual objects? What if the triangles were 8 units apart in the same orientation? Does the formula for gravitational attraction work better if applied to the individual triangles now?

To summarize, mathematically elegant laws of physics may have the form they do because they are idealizations of simple cases, with simple geometry. They do approximately work in the real world, even in large objects, because of symmetry and summation. It’s lucky that most real-world situations can be modeled without discontinuities, though this is not always the case, as we saw in the Insights puzzle involving the elliptical pool table and the ellipsoid paradox. Even more problematic are chaotic, messy situations involving hundreds of particles with multiple interactions, where predictive mathematical beauty might not be possible and we have to revert to supercomputer simulations. Seeing mathematical beauty everywhere in the natural sciences, as Wigner did, could simply be the result of cognitive bias, an emotional reaction to aesthetic beauty. In quantum physics, for instance, the mathematics of renormalization, which is crucial for quantum field theories, has been characterized as “ugly” or “not legitimate” by several giants of physics, including Paul Dirac and Richard Feynman. Yet it works well in practice.

What is your take, Quanta readers — what do you think of Wigner’s thesis? Why do you think we have an intrinsic affinity for beautiful, elegant mathematics? Is it a miracle, or are we drawn to it for some other more mundane reason?

Happy puzzling!

Editor’s note: The reader who submits the most interesting, creative or insightful solution (as judged by the columnist) in the comments section will receive a Quanta Magazine T-shirt or one of the two Quanta books, Alice and Bob Meet the Wall of Fire or The Prime Number Conspiracy (winner’s choice). And if you’d like to suggest a favorite puzzle for a future Insights column, submit it as a comment below, clearly marked “NEW PUZZLE SUGGESTION.” (It will not appear online, so solutions to the puzzle above should be submitted separately.)

Note that we may hold comments for the first day or two to allow for independent contributions by readers.

Update: The solution has been published here.