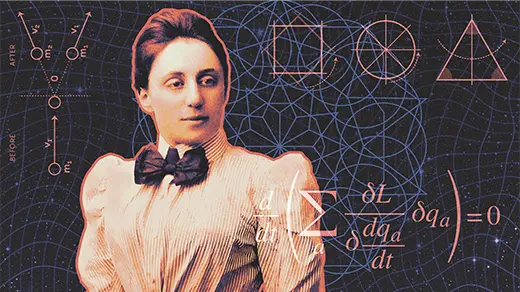Symmetry

From living matter to molecules to elementary particles, the world is made of “chiral” objects that differ from their reflected forms.

Britta Späth has dedicated her career to proving a single, central conjecture. She’s finally succeeded, alongside her partner, Marc Cabanes.

Emmy Noether showed that fundamental physical laws are just a consequence of simple symmetries. A century later, her insights continue to shape physics.

A new proof marks the first progress in decades on important cases of the so-called kissing problem. Getting there meant doing away with traditional approaches.

Mathematicians have long wondered how “shapes of constant width” behave in higher dimensions. A surprisingly simple construction has given them an answer.

What do the integers have in common with the symmetries of a triangle? In the 19th century, mathematicians invented groups as an answer to this question.

If you cover a surface with tiles, repetitive patterns always emerge — or do they? In this week’s episode, mathematician Natalie Priebe Frank and co-host Janna Levin discuss how recent breakthroughs in tiling can unlock structural secrets in the natural world.

Two mathematicians have proved a long-standing conjecture that is a step on the way toward finding the worst shape for packing the plane.

Time is all around us: in the language we use, in the memories we revisit and in our predictions of the future. But what exactly is it? The physicist and Nobel laureate Frank Wilczek joins Steve Strogatz to discuss the fundamental hallmarks of time.