The Largest Sofa You Can Move Around a Corner
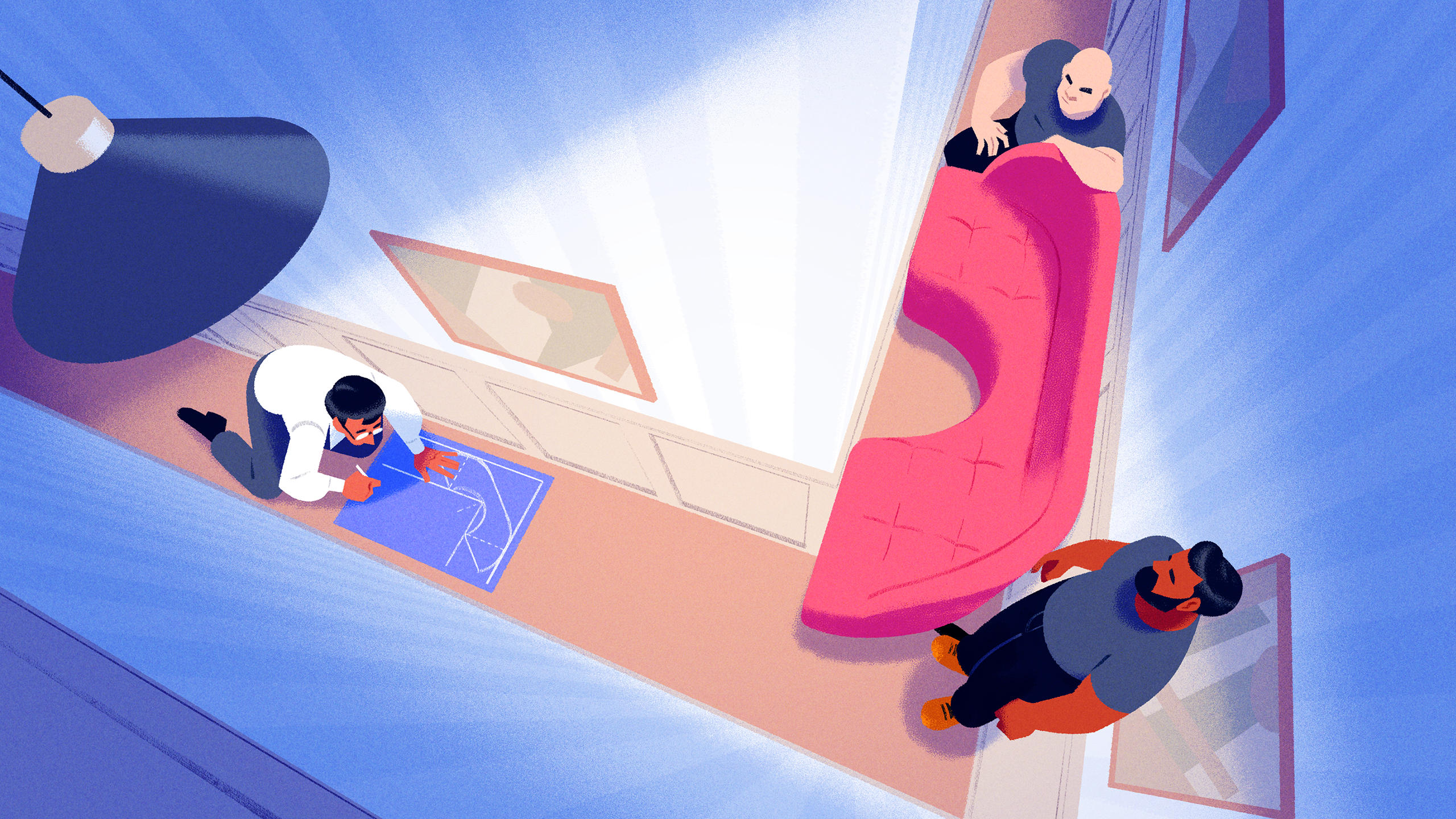
Mathematicians have proved that any sofa bigger than this one will get stuck at the hallway’s corner. Of course, movers might resolve the problem by simply tilting the sofa on its end, but in the mathematical version it must stay flat.
Tommy Parker for Quanta Magazine
If you’ve ever moved into a new home, then you know how difficult it can be to steer bulky furniture through narrow hallways or around awkward corners. Mathematicians have been trying to solve this problem, too, ever since 1966, when Leo Moser framed it in quantitative terms. Say you want to move a two-dimensional shape — your sofa (disregarding its height) — through an L-shaped hallway. For simplicity, assume the hallway is one unit wide. What’s the size of the largest shape that won’t get stuck?
It’s simple enough to find different shapes you can maneuver around the hallway’s 90-degree corner. A square with a side length of 1 does the trick. So does a semicircle with a radius of 1 (and an area of π/2, or approximately 1.57). But such shapes are relatively small. The moving sofa problem, as it soon came to be called, required mathematicians to come up with cleverer solutions.
In 1992, Joseph Gerver of Rutgers University proposed a particularly clever curved shape with an area of approximately 2.2195. Mathematicians suspected that it answered Moser’s question. But they couldn’t prove it.
Now a young postdoctoral researcher has. In a 119-page paper, Jineon Baek of Yonsei University in Seoul showed that Gerver’s sofa is the largest shape that can successfully pass through the hallway.
The paper isn’t just noteworthy for resolving a 60-year-old problem. It has also garnered attention because mathematicians had expected any eventual proof of the conjecture to require a computer. Baek’s proof did not. Mathematicians now hope that the techniques he used might help them make progress on other kinds of optimization problems.
Perhaps even more intriguing, Gerver’s sofa, unlike more familiar shapes, is defined in such a way that its area can’t be expressed in terms of known quantities (such as π or square roots). But for the moving sofa problem — a very simple question — it’s the optimal solution. The result illustrates that even the most straightforward optimization problems can have counterintuitively complicated answers.
Sofas and Telephones
The first major progress on the moving sofa problem came in 1968, just two years after Moser posed it. John Hammersley connected two quarter circles with a rectangle, then cut a semicircle out of it to form a shape that resembled an old telephone. Its area was π/2 + 2/π, or approximately 2.2074.
Hammersley also showed that any solution to the problem could have an area of at most $latex 2\sqrt{2}$, or about 2.8284.
A couple of years later, Gerver, then a graduate student at the University of California, Berkeley, learned about the question. “Another grad student told me this problem and challenged me to find the answer,” he said. “He never said anything about it being unsolved. So I thought about it for a few days. Finally, I came back to him and said ‘OK, I give up. What’s the solution?’ And he refused to tell me! He said, ‘Just keep thinking about it. You’ll get it.’”
Gerver thought about it sporadically over the next 20 years. But it wasn’t until 1990, when he mentioned it to the renowned mathematician John Conway, that he found out it had never been solved. The realization motivated him to spend more time with the problem, and he soon came up with a potential solution.
Gerver’s sofa looked a lot like Hammersley’s telephone, but it was far more complicated to describe, consisting of 18 different pieces. (As it turned out, Ben Logan, an engineer at Bell Labs, independently uncovered the same shape but never published his work.) Some of the pieces were simple line segments and arcs. Others were more exotic, and tougher to describe.
Still, Gerver suspected that this complicated shape was optimal: It possessed many features that mathematicians expected the optimal sofa to have, and he was able to prove that making small perturbations to its contours wouldn’t yield a suitable shape with a bigger area.
In 2016, Dan Romik, a mathematician at the University of California, Davis, provided a more conceptual description of Gerver’s sofa. He wrote down a set of 22 equations that had 22 different variables; the only solution to the whole system of equations, when graphed, was the phonelike shape Gerver had found.
The following year, Romik and Yoav Kallus used computer-assisted techniques to drive Hammersley’s upper bound for the area of the sofa down, bringing it closer to Gerver’s lower bound. But they couldn’t fully close the gap. A new idea was needed.
A Space of Sofas
Shortly after starting graduate school at the University of Michigan in 2016, Baek took a four-year leave of absence to serve in South Korea’s military (a requirement for male citizens). During this time, he encountered the moving sofa problem in a blog post. At first, he said, working on it “was a way to chill out from the day job.” But soon he started thinking about it more seriously. He had an idea for how to prove that Gerver’s sofa was the solution, but there were many details he still needed to pin down. When he returned to graduate school in 2021, he decided to dedicate himself to the problem.
Typically, a doctoral student in mathematics chooses an adviser, who then assigns them a problem to work on. But Baek was determined to work on the moving sofa problem. This made finding an adviser difficult; no one at Michigan felt they had the right kind of expertise. But eventually, Baek approached Michael Zieve, who specializes in the distant field of algebra. He said yes. “I’ve never advised someone this far from what I know before,” Zieve said. “But I’m open to advising students on just about anything.”
During his doctoral studies, Baek built on Kallus and Romik’s work, developing more powerful computational tools to drive the upper bound down even further. “Jin is by far the best student I’ve ever seen with computers,” Zieve said. “He’s able to spot patterns with the computer’s help that you just wouldn’t have ever guessed.”
When Baek finished his graduate studies last year, he intended to continue pursuing computational approaches to fully settle the moving sofa problem. But within just a few months, he realized that he might not need to rely on computers at all.
Mathematicians already knew that any solution to the problem would have to satisfy certain conditions. The optimal sofa would have to be able to rotate in a certain way, and it would have to have a section carved out of its bottom to make room for the hallway corner, among other properties.
There are infinitely many shapes that meet these constraints. Baek narrowed them down, showing that the optimal shape had to at least resemble Gerver’s sofa. He then represented each sofa — there were still infinitely many of them — as a point in an infinite-dimensional space. Ideally, he would have come up with a function that took each point as an input and gave that sofa’s area as an output. Then he’d just have to determine the point at which the function’s output was biggest.
But that was an impossible task: There’s no one formula that can give the area for every kind of shape. (Think about how you use different functions to find the areas of circles versus triangles.)
So Baek decided to study the areas of the shapes indirectly instead. He invented a new function, which he called Q. He defined this function so that it had several important properties.
First, he showed that for any sofa in his space, the output of Q would be at least as big as the sofa’s area. It essentially measured the area of a shape that contained the sofa. That meant that if Baek could find the maximum value of Q, it would give him a good upper bound on the area of the optimal sofa.
This alone wasn’t enough to resolve the moving sofa problem. But Baek also defined Q so that for Gerver’s sofa, the function didn’t just give an upper bound. Its output was exactly equal to the sofa’s area. Baek therefore just had to prove that Q hit its maximum value when its input was Gerver’s sofa. That would mean that Gerver’s sofa had the biggest area of all the potential sofas, making it the solution to the moving sofa problem.
This was where Baek’s definition of Q became even more important. He’d set everything up so that Q behaved nicely — essentially like a simple parabola. It’s relatively easy to find the maximum value of such a function. In this case, Baek was able to show that maximizing Q gave him a shape that satisfied a particular set of conditions — and that the equations that defined Gerver’s sofa also satisfied those conditions.
He’d settled the decades-old conjecture, showing that Gerver’s sofa was the biggest possible shape that could move through the hallway without getting stuck at its corner.
The proof, which is still being peer-reviewed, offers a fresh approach to optimization problems. It combines techniques from disparate areas of mathematics to make a prohibitively difficult problem tractable — all without computer assistance. “The fact that Jin was able to do this without computers was impressive,” Zieve said. “That showed there were real, significant new ideas.”
All of which delights Gerver, more than 30 years after he proposed his solution. “I’m 75 years old now,” he said. “I feel lucky to have lived long enough to see someone finally solve the problem.”



