Why Our Perpetual Energy Puzzle Fails

In our April Fools’ Day Insights puzzle, we offered two paradoxes for Quanta readers to resolve. As you’ll see, neither paradox withstands scrutiny, but the exact way in which they fail is interesting to reason out, as several readers discovered in their lengthy responses.

Paradox 1

The first problem described an object that I claimed could produce energy forever, in defiance of the second law of thermodynamics. The object is sketched and briefly described below:

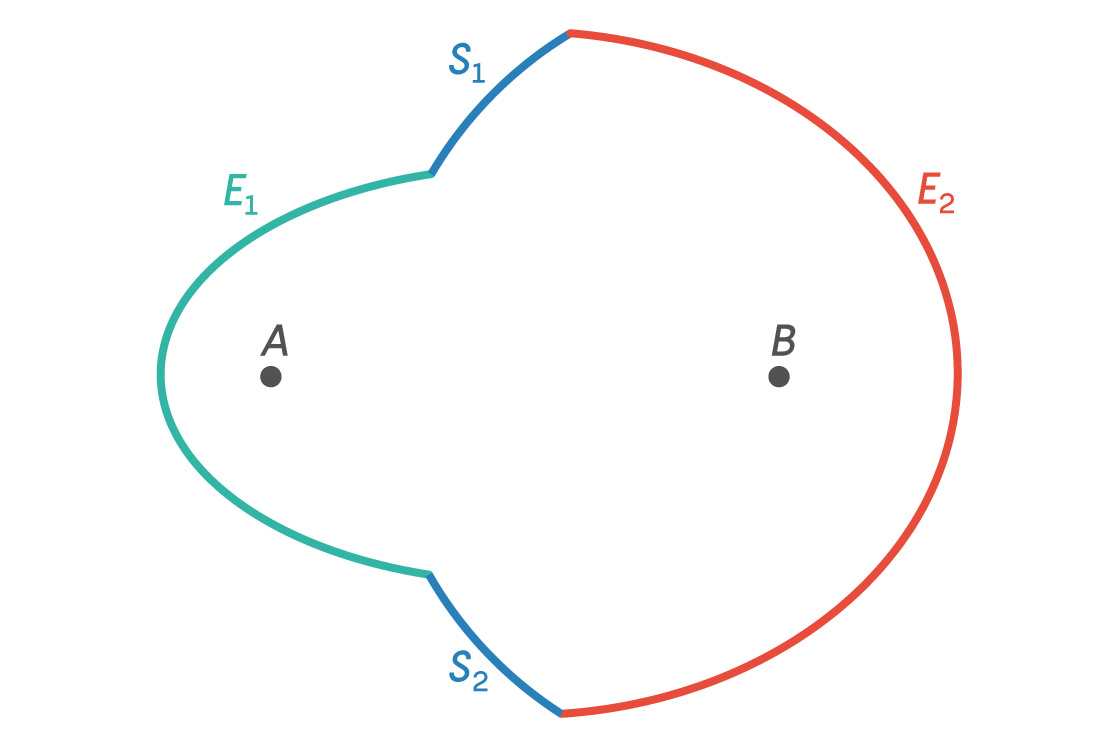
E₁ and E₂ are sections of two concentric ellipses with foci located at points A and B. S₁ and S₂ are arcs of a circle with center B. Since they are arcs of the same circle, every straight line from B to S₁ or S₂ is a radius and therefore normal to the inner surface. This entire figure is a cross section of a hollow object that is created by the solid of revolution of the plane figure. The inside surface of this object is silvered and 100% reflective (or as close to it as practically possible). At A and B are small spherical blackbodies made of thermoelectric materials. They each have thin wires leading to battery terminals outside. The whole structure is completely sealed.

For more details on how this object is supposed to work, check out the original puzzle column. In brief, the geometry dictates that 100% of the radiation originating from the blackbody at A will land on and be absorbed by the blackbody at B because both A and B are the foci of ellipses E₁ and E₂. However, a significant proportion of rays that start from B will land on S₁ or S₂ and be reflected back to B. So if the objects start out at the same temperature, B will receive more rays from A than A receives from B. So B will heat up relative to A, creating a temperature gradient that we can tap for energy by connecting the battery terminals to a circuit. If the temperatures of the two bodies equalize, we just wait awhile and let the above process repeat itself. Voila — inexhaustible energy!

This “ellipsoid paradox,” which can take the form of various ellipsoidal constructions, has been known since 1959. It doesn’t work, of course, but despite several articles debunking it through the years, it has occasionally been taken seriously.

Paradoxes illustrate faulty habits of thought, and that’s a good place to start here. I believe the key mental error in this case is our implicit trust in abstract geometry to solve real-world problems. Though it works most of the time, geometry, like the rest of mathematics, involves idealizations like the concept of a dimensionless point, which makes its way into physics in the form of “point particles” or “point masses.” These abstract constructs have proved very powerful — in the theory of gravitation, for instance.  We know that real-world masses don’t behave exactly like point masses, but they approximately do. Real-world projectiles do not trace out perfect parabolas, but for all practical purposes, they come close enough. We have been spoiled by these “almost exact” successes, and so it seems paradoxical when we encounter rare instances where the approximations don’t work and the idealizations give us results that are qualitatively wrong, as in this case.  The ellipsoid object we described works perfectly well in the universe of point particles, where rays from one focus of an ellipsoid are reflected precisely to the other. But the moment you replace the point particle with a finite real-world object, no matter how small, it no longer works — the approximation fails. There is a yawning discontinuity between zero size and any finite object. To see why, look at the illustration below.

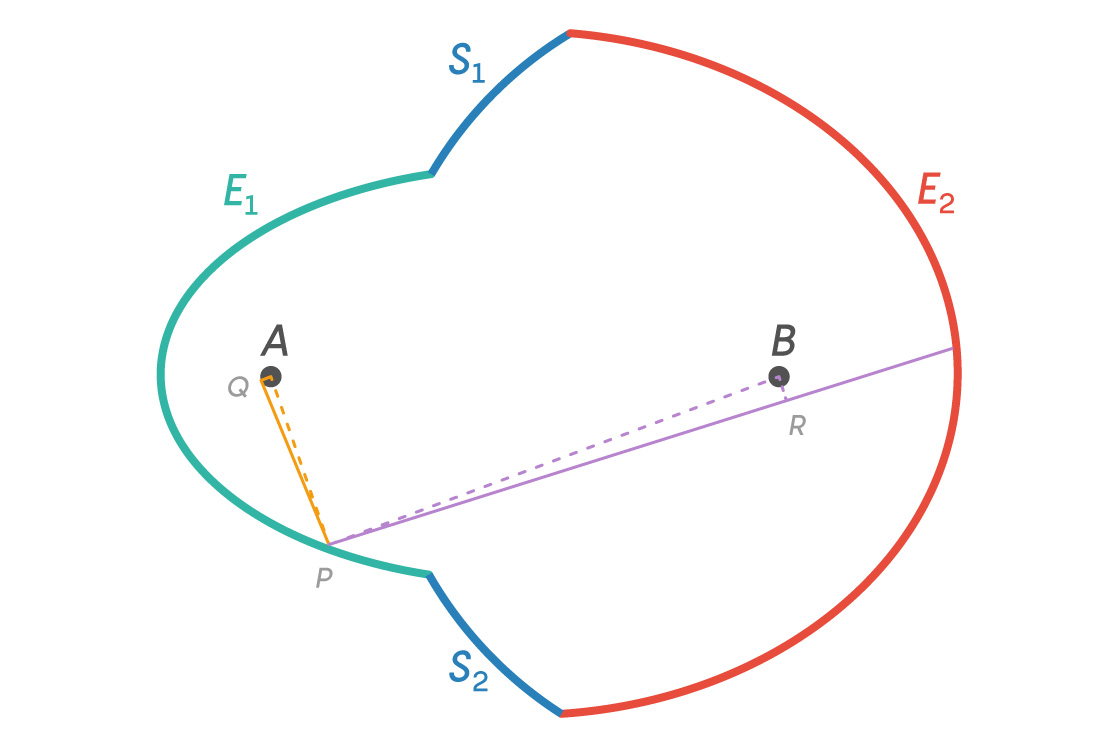
At A and B (the point foci of the two ellipses) are small round objects of the same size. The dashed lines AP and BP join point P on E₁ to the two point foci. Now consider a ray QP that originates tangentially from point Q on the surface of the small object at A. After reflecting off of E₁ at point P, it travels in the direction PR. Now, by the laws of reflection, the angles APQ and BPR are equal. The angle AQP is a right angle (since QP is a tangent), and by construction, so is angle BRP. Therefore, the gold and purple triangles are similar. Since the purple triangle is much larger than the gold one, it is clear that BR is much longer than AQ, the radius of the two objects. As a result, the ray PR misses the object at B by quite a large distance. In fact, it is clear that a significant proportion of rays striking E₁ from an object at A will miss a similar-size object at B.  The same is true of rays starting from the object at B that strike E₂ — a large proportion of them will miss the object at A. Thus, the conclusion that 100% of the rays from an object at A will end up at the object at B, true as it may be for a point object, is completely false for even the tiniest real-world objects. We just used geometry to demolish a false conclusion arising from faulty mental habits about geometry!

OK, many of the rays from the two objects miss each other, but what happens to them? They bounce about in the interior of the object, and most eventually end up on either A or B, though a few might even keep bouncing around forever, something that Spotted Snifflegrub alluded to. If both objects are at the same temperature, there will be exactly as many rays going from A to B as there are going from B to A. How do we know this? We can construct a computer simulation and count a large number of rays, as was done in this paper, and show that the numbers actually even out.

What mandates such a result? One simple principle is reversibility. If a ray starts at A, reflects back and forth, say, 100 times, and lands on B at a certain angle, then a ray emitted from that same spot on B at the same angle will follow the same pattern in reverse and land on A. Therefore, if radiation and absorption are taking place in all directions, A and B will exchange the same number of rays on average. We do not have to worry about rays that bounce off S₁ or S₂ and return to B, or rays that keep bouncing off the walls forever. It is ironic that the integrity of the second law of thermodynamics, which is responsible for irreversibility in the world, is safeguarded at the micro level by this reversibility.

Several readers correctly pointed out that the paradox does not work for finite objects, including FosterBoondoggle, Laurence Cox, James Ough and Manuel Fortin. Manuel Fortin also used a diagram to show how some rays from A would miss B if A and B were spherical objects.

Some readers pointed out that even if the device worked, both objects would cool down over time and the temperature difference would decrease to zero. If the device worked, that would be true. But then you could construct the device using two locking halves that you could take apart, allow to reheat to room temperature, and then reuse perpetually.

Other readers mentioned quantum effects and the impossibility of having perfect mirrors. Both of these issues would perhaps reduce the efficiency of the device, but they are not the reason it doesn’t work.

Paradox 2

Consider the following stipulations:

1. The solution to this Insights column will definitely be published in Quanta in May.
2. If, for the purposes of this problem, we define a week as starting on a Monday and ending on a Sunday, then every day in May will fall in one of five separate weeks (one partial week followed by four full weeks). I declare with certainty that you will not be able to predict in which of these five separate weeks the solution column will be published.

Now, as we know, Quanta readers are a brilliant bunch. Suppose a reader reasons as follows: “If the column is not published by the end of the fourth week, I will be able to predict with certainty that it will be published in the fifth week. Therefore, it cannot be published in the fifth week. But if it cannot be published in the fifth week, then, if it is still unpublished at the end of the third week, I can be certain that it will be published in the fourth week. Therefore, it cannot be published in the fourth week. Now I can apply the same serial logic to prove that it cannot be published in the third, second or first weeks. Therefore, the solution cannot be published at all!”

OMG, this puts me in a terrible bind! My editor will certainly not be pleased. Is this reasoning valid? Why or why not? What if there is a tiny probability (say 0.001) that the column will not be published at all (we all get COVID-19, or the financial system collapses and no business activities can take place)? Assume that “certainty” now means “99% or greater probability of being right.” Does that change the conclusion?

This is a version of  a classic paradox variously called the unexpected hanging or the surprise test, both of which are about a future event whose exact date the protagonist cannot know. The language of these paradoxes has been criticized as being ambiguous, so I thought I would remedy the situation by introducing the idea of certainty.

Unfortunately, I don’t think I was successful. Several readers still found the statement ambiguous, and this ambiguity was explored in excruciating detail by Steve Taylor. Mea culpa. I think a better statement of what I was getting at would have been “I declare that you will not be able to predict correctly with logical certainty which of these five separate weeks the solution column will be published in.” Some readers also seized on my failure to specify that the publication would be in May 2020. Well, while this was not explicitly stated, the next year with the pattern of weeks I described would be 2026. Surely a long time to wait for the solution of a puzzle, as Tommy pointed out!

Neither of these objections invalidates the problem, though. There are always ambiguities that need to be clarified with these kinds of problems, not just because of the language used but also because of assumptions that are not spelled out. If every possible valid and invalid interpretation has to be explicitly specified, a puzzle statement will look like a legal document. That said, here are two explicit clarifications, without which the problem loses meaning.

1. By “certainty,” we mean objective, logical certainty — an inference based on an assumption that all declarations are true, and not just a “feeling of certainty.”
2. You only get one chance to express your certainty — it has to be about one particular week. If you express certainty that I will post the article in a particular week, and your prediction fails, then the game is over. You cannot again express certainty for a different week. Without this stipulation, the problem becomes absurd.

There is another implicit assumption in the problem’s classical versions, the unexpected hanging and the surprise test: that there is a “neutral block of time” at the end of the day during which the hanging or test cannot take place. This is implied by the nature of business or school hours. There is no such limitation in our problem as Quanta publishes online, but let us assume for a moment that there’s a cutoff time, say 8 p.m., after which publication will not occur that day. This gives a reader time to make a prediction about the next week, without fear that the publication will happen at the same time as the prediction.

In this version of the paradox, which is essentially the classical one, the general consensus is that the first inference is correct: If the solution is not published by 8 p.m. at the end of the fourth week, a reader can be certain that it will be published in the fifth week. However, the follow-up inductive inferences for the third and earlier weeks are not sound. You cannot make an inference about the past based on what might be conditionally true in the future. In other words, while it’s true that if the puzzle solution hasn’t been published by the end of the fourth week, it has to be published in the fifth week, that knowledge has absolutely no bearing on what you can know at the start of the month or even after the first, second or third weeks. You can only be certain it hasn’t been published in the first four weeks after the end of the fourth week. So all the author has to do to avert the paradox is to choose a publication week using some random algorithm that the readers do not know about. That’s exactly what I did. Of course I can’t tell you what it was!

Gert-Jan Schouten and Tommy considered a version of the problem in which there are no constraints on the time of the publication, and they came up with a creative solution. I think they have a valid argument, with one caveat. Their point is that the article can be published at the last instant of the fourth week, or the first instant of the fifth week. In the first case, the reader would be wrong to make a prediction of a fifth-week publication no matter how late the prediction is made in the fourth week. Similarly, a prediction for a fifth-week publication made on the first instant of the fifth week would not be valid as a prediction because the publication will have been completed at the same time. Very clever! The caveat is that there must be an agreed-upon last and first instant of time for every week — a kind of discrete time “bin” similar to that of a computer clock — whether it be a minute, a second, a millisecond or, as Gert-Jan Schouten suggested, a nanosecond. We cannot treat time as continuous, because if we do, then we have the prospect of a midnight shootout between the reader and the author, similar to a Wild West gun duel, with both parties attempting to complete their job (the prediction or the publication) closer to midnight than the other party. In fact, the shootout at the end of the fourth week will be less of a gun duel and more of a game of chicken, with each participant waiting to be as agonizingly close to midnight as possible. It’s fun to visualize, but thankfully it didn’t have to materialize!

(Note: Tucker the third raised the objection that if the article is published exactly at midnight between Sunday and Monday, then the week of publication is ambiguous. This is incorrect: by convention, midnight is the start of the subsequent day. Also, time zone differences are immaterial because Quanta gives the day of publication in its own time zone only.)

As for the last part of the problem about certainty being taken as greater than 99% probability, well, I added that because in real life we know that nothing can be 100% certain. For practical purposes, we ignore the possibility of unexpected low-probability events when we say we are certain. In this case, it doesn’t make a difference because the probability I gave of the solution not being published — 0.001 — is much too small. The probability of publication was still clearly above the given certainty threshold.

The prize for this month goes to Manuel Fortin. Congratulations! Thank you to all who contributed. See you next month for new Insights!