A Mathematician’s Unanticipated Journey Through the Physical World
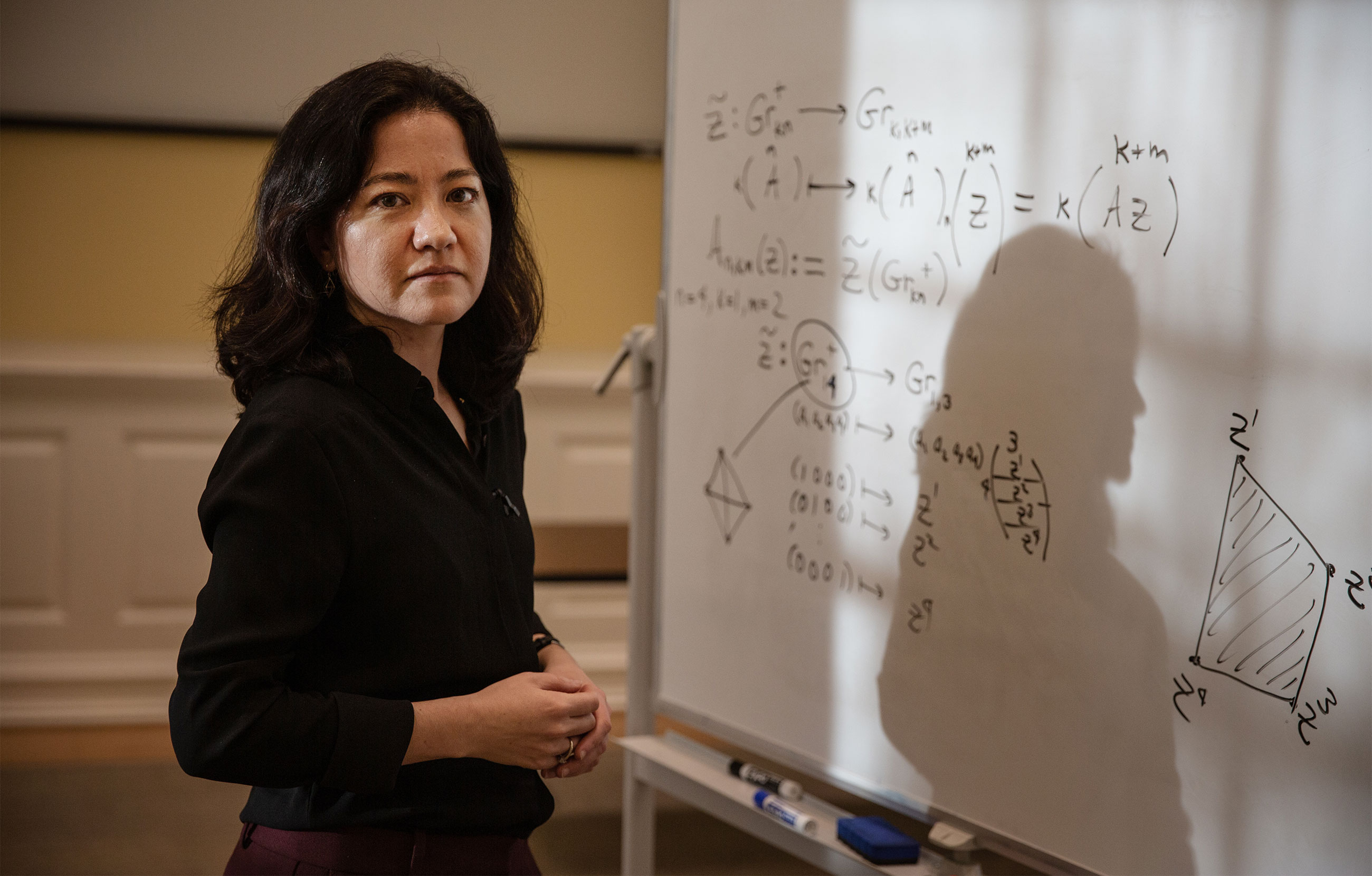
Andrea Patiño Contreras for Quanta Magazine
Introduction
The outline of Lauren Williams’ mathematical career was present very early on in her life.
“Ever since I was a kid, I’ve always loved patterns,” said Williams. “I enjoyed being given a sequence of numbers and having to find the pattern and predict the next number.”
But while many kids are enchanted by patterns, few end up following them as far, or to such unexpected places, as Williams has. As a professor at Harvard University — where she became only the second tenured woman mathematician in the university’s history — she has uncovered correspondences far more bewildering than anything she learned in grade school.
They all involve a single mathematical object that can be described in a number of different ways. But by looking at it from an entirely new perspective, Williams, 42, has proved that it’s the key to decoding the secrets behind a wide range of seemingly unrelated phenomena in math — and in nature.
“She’s always been fearless,” said Federico Ardila of San Francisco State University, who was in graduate school with Williams. “She’s not afraid to build bridges where they didn’t seem to exist.”
The geometric object that weaves through Williams’ work is called the positive Grassmannian. It’s a shape that performs a kind of record-keeping function: Every point on it represents a specific instance of some simpler geometric object. It’s a shape that keeps track of other shapes.
Around the time Williams started graduate school at the Massachusetts Institute of Technology in 2001, mathematicians were developing a new way of thinking about the positive Grassmannian. Instead of thinking of it as a single geometric object, they were trying to understand it in terms of the pieces that make it up.
That perspective captivated Williams, and over the last two decades she’s established many of its most far-reaching implications.

Andrea Patiño Contreras for Quanta Magazine
In often dramatic fashion, Williams has also proved that the pieces of the positive Grassmannian can be reassembled in a form that explains everything from the movement of tsunami waves to particle collisions at the frontier of quantum physics. It’s a potpourri of insights that cohere around the positive Grassmannian, and around the unique mind that generated them.
“Lauren is one of these people who thinks so clearly,” said Nima Arkani-Hamed, a theoretical physicist at the Institute for Advanced Study. “She is open-minded and adventurous.”
Point of Origin
Williams grew up in the Los Angeles suburbs, the oldest of four sisters. Her father was an engineer, and her mother, a third-generation Japanese American, taught English.
As a child Williams enjoyed playing the violin and writing poetry, but she loved reading most of all. At night, she’d stay up past her bedtime with a lamp pulled under her comforter (she eventually burned a hole through her sheets). During summer days, “I spent hours sitting in the branches of an apricot tree in our backyard, reading a book and eating apricots,” she said.


As a child, Williams learned the violin and dreamed of becoming a writer.
Andrea Patiño Contreras for Quanta Magazine; Courtesy of Lauren Williams
Math rose to her attention in fourth grade when she participated in an elementary school math competition. “I unexpectedly won the contest, and the two teachers who organized it sort of took me under their wing,” she said.
When she was 16, she spent a summer at a math research program for high school students hosted by MIT. That was her first serious introduction to combinatorics — an area of math concerned with breaking complicated objects into pieces and then classifying and counting them. She learned about the discipline from an MIT graduate student named Satomi Okazaki — who herself happened to be the student of a mathematician named Richard Stanley. (Stanley would eventually become Williams’ doctoral adviser.)
When she went to college, at Harvard, she was excited to join a wider intellectual world. “There was no end of classes and activities I wanted to try, and my peers were just as excited as me about learning and doing everything.” She majored in mathematics at Harvard, where she graduated in 2000, and went on to graduate school at MIT. There she started studying a mathematical object with many guises: the Grassmannian.
“If you understand the Grassmannian well, you can go in many different directions. It’s central in math,” said Bernd Sturmfels of the University of California, Berkeley, who is also the director of the Max Planck Institute for Mathematics in the Sciences in Leipzig, Germany.
The Grassmannian gets its name from Hermann Grassmann, who first formalized it in the mid-1800s. Only it’s not one exact geometric object, but rather a family of them.
To get a sense for a single Grassmannian, let’s start with just two numbers, 1 and 3. The 3 indicates that we’re in three-dimensional space. The 1 means we’re going to think about one-dimensional lines within that space.
In this three-dimensional space there are three axes — x, y and z — that all intersect at a crossroads, the origin. Now picture a line that runs through the origin. Go a step further and try to imagine all the lines that could go through the origin, each with its own unique trajectory.
Next, imagine positioning a sphere so that it’s centered around the origin. Most of those lines will intersect this sphere twice, in the northern and southern hemispheres (except the ones that pass through the equator). This makes the two hemispheres largely redundant — they carry the same information about the lines — so we can forget the southern one. The leftover northern hemisphere is the Grassmannian formed by one-dimensional lines in three-dimensional space. Or, as mathematicians write it, Gr(1,3).
This means that if you know the coordinates of a point on the northern hemisphere, you know everything about the one-dimensional line through the origin that passes through that point. The Grassmannian is an example of what mathematicians call a moduli space, meaning it’s a single geometric object that serves as a concise way of keeping track of infinitely many others.
“As you move [from line to line] you’re moving from one point to another on the Grassmannian,” said Ardila. “It’s almost like a remote control.”
Samuel Velasco/Quanta Magazine
This is just one example of a Grassmannian. If we’d started with the numbers 4 and 10, we’d instead be thinking about four-dimensional planes passing through the origin in 10-dimensional space — and the Grassmannian, Gr(4,10), would be the shape in which each point represents one of those four-dimensional planes. You can construct infinitely many different Grassmannians by starting with distinct pairs of whole numbers.
Beginning in the early 1990s, many mathematicians started to focus on a particular part of the Grassmannian called the positive Grassmannian. In our example, Gr(1,3), it is one-quarter of the northern hemisphere. It’s referred to as the “positive” part of the Grassmannian because, roughly, all the lines that cut through it have a non-negative slope.
But to really understand its place in mathematics, mathematicians first had to learn how to take the Grassmannian apart.
Out of Many, Few
In the 1970s and 1980s, Gian-Carlo Rota and his student Richard Stanley came up with a new way of thinking about sophisticated mathematical shapes. They’d take those objects — which may have been hard to study on their own — and break them into combinatorial pieces that were more tractable.
“You have some very complicated object that is hard to understand,” said Melissa Sherman-Bennett, a graduate student at Berkeley who works with Williams. “But you can break it into pieces that give you more insight into this big complicated thing.”
When Williams arrived at MIT, she read foundational works from the 1990s by George Lusztig and his student Konstanze Rietsch that had introduced the positive Grassmannian, and more recent papers by Alexander Postnikov that applied a combinatorial perspective to the shape. Postnikov was at MIT, and Williams spent a lot of time talking with him. She was fascinated by the way his work connected this already canonical shape to even more widespread parts of mathematics.
![A diptych. On the left, Lauren Williams drawing the positive Grassmannian on a whiteboard. On the right, a close-up of the positive Grassmannian drawn on a whiteboard. outdoor with mask: [no caption]](https://www.quantamagazine.org/wp-content/uploads/2020/12/Williams-2K-Whiteboard.jpg)
![A diptych. On the left, Lauren Williams drawing the positive Grassmannian on a whiteboard. On the right, a close-up of the positive Grassmannian drawn on a whiteboard. outdoor with mask: [no caption]](https://www.quantamagazine.org/wp-content/uploads/2020/12/Williams-2K-Whiteboard-detail.jpg)
Williams’ work demonstrates how the pieces of the positive Grassmannian can be translated into other areas of mathematics and physics.
Andrea Patiño Contreras for Quanta Magazine
“I found it to be a very beautiful confluence of ideas,” Williams said.
To understand how the Grassmannian breaks into pieces, recall that each point on it encodes the properties of a line or multidimensional plane passing through the origin. Those planes are defined by vectors that can be written down as arrays of numbers called matrices. The size of a matrix depends on the Grassmannian. For Gr(1,3) — one-dimensional lines in three-dimensional space — each line through the origin is specified by a 1 × 3 matrix, such as:
[1 2 3]
The numbers in the matrix serve as the coordinates for the point in the Grassmannian that encodes the line. The Grassmannian itself contains infinitely many points, which can’t be counted in a discrete, finite way. But it’s possible to extract additional data from the matrices that can be counted.
Many matrices have a measurement called a determinant, which is a single value calculated using the numbers in the matrix. They also have “subdeterminants,” which are calculated based on a subset of the values in the matrix; a 1 × 3 matrix has three subdeterminants.
For Williams’ work, the significance of those subdeterminants lies in their signs, which can be positive, negative or neither (if the subdeterminant is zero). With the positive Grassmannian, the options are even more limited: subdeterminants can only take values that are either positive or zero.
This turns something infinite and uncountable into something discrete and possible to sort: While there are infinitely many different 1 × 3 matrices, their three subdeterminants can have only eight different sign patterns: (000), (00+), (0++), and so on. And for technical reasons, mathematicians don’t need to consider one of them, (000), which leaves just seven categories for those infinite points to be divided into.
Samuel Velasco/Quanta Magazine
Points sort into different buckets, or “cells,” based on their sign pattern. You can think of these seven cells as the seven puzzle pieces that make up the positive Grassmannian. The number and shapes of these pieces isn’t obvious when you first look at the overall shape. They become apparent when you sort points by their sign patterns — all the points with a given sign pattern fill out the shape of a single cell, or puzzle piece. This process of sorting points by sign patterns to reveal the shapes of puzzle pieces works especially well for the positive part of the Grassmannian.
“The combinatorics is extremely rich,” said Rietsch.
Starting in graduate school, Williams proved a number of different features of the way points from the positive Grassmannian sort into cells. In 2003 she devised a formula for counting the number of different cells found in positive Grassmannians of any dimension. The result foreshadowed a lot of the innovative work later in her career.
“I think she’s one of the masters of capturing the combinatorial nature of objects that don’t seem combinatorial,” Ardila said.
After she received her doctorate from MIT in 2005, this combinatorial perspective on the positive Grassmannian started leading Williams into unlikely collaborations.
Making Waves
There are lots of ways to chart a career in math. One is to devote yourself to developing a new theory or attacking a prominent open problem. But that’s not what motivates Williams.
“I’d rather not be working on what everybody else is working on,” she said. “I sort of don’t like feeling I’m competing against other people toward the same goal.”
Her collaborators have also noticed this unusual trait. “Lauren is one of the smartest people I’ve ever worked with, but I’ve never felt she had this pretense of competition,” said Ardila. “She has this gentleness about her.”
Williams’ preference for less-trafficked problems found an outlet right after graduate school, when she wrote a series of papers with the mathematician Sylvie Corteel that explored an unexpected link between the combinatorics of the positive Grassmannian and statistical physics. In addition to their mathematical results, Williams gained something else by working with Corteel, who had a baby during their first collaboration.
“When I was much younger, I worried about whether it was possible to be a successful academic and also have a family,” she said. “It was useful for me that fairly early in my career I had these collaborations with slightly older women who were making it work.”
Williams’ research took another surprising turn in 2009, soon after she joined the faculty at Berkeley. While looking for new results on the positive Grassmannian, she noticed that a physicist at Ohio State University had cited her work in his research on shallow water waves.
“If someone writes a paper with the positive Grassmannian in it, she always looks at it,” said the physicist in question, Yuji Kodama. “Of course, she didn’t expect shallow water waves.”
Kodama’s work focused on a particular type of wave called a soliton, or solitary wave. The most famous example of the phenomenon is a tsunami. More often, though, soliton waves occur near the shore. The mathematics behind a single soliton propagating on its own is relatively simple, but it grows more complicated when solitons cross each other. Physicists model them using the Kadomtsev-Petviashvili, or KP, equation: Feed the equations the position of a wave and the equations output its height at any time in the future. Kodama was trying to understand different types of solutions to the KP equations, representing different types of wave interactions.
“If one soliton and another interact … lots of patterns appear, and we like to classify these,” said Kodama.
Williams tried reading Kodama’s work, eager to see how the Grassmannian might fit in, but it was too far from her own research for her to understand it. So she invited him to Berkeley to explain it to her in person. Even then, communication wasn’t easy.
“He’s a physicist and an older Japanese man, and we had a lot of trouble understanding each other that first day. It was like we were speaking different languages,” said Williams.
As they talked, Kodama sketched simple schematics to illustrate patterns of wave interaction: Two lines representing two waves converge at a point, and then a single line representing a new wave emerges. The drawings looked familiar to Williams. She quickly recognized that they mirrored pictorial representations called planar bicolored graphs that mathematicians use to describe points on the positive Grassmannian.
Samuel Velasco/Quanta Magazine
“He’d explain stuff and draw pictures and I had a lot of trouble following his explanations, but I could draw the same picture in a completely different way,” said Williams.
Previous work had established a one-to-one relationship between points on the positive Grassmannian and solutions to the KP equation: Start with a point on the positive Grassmannian, apply some complicated mathematics, and you’ll get a solution to the equations that represents a particular wave interaction.
Emboldened by the matching pictures, Kodama and Williams looked for deeper connections between the positive Grassmannian and shallow water waves. The pair ended up showing that when you associate a point on the positive Grassmannian with a solution to the KP equation, the cell that point belongs to dictates a lot about the wave pattern represented by the solution to the equations.
“The large-scale behavior of a wave formation is entirely determined by which cell your point in the positive Grassmannian lies in,” Williams said.
One of their papers also included a haiku Kodama and Williams wrote, partly in recognition of their shared Japanese heritage:
Arrangements of stones
reveal patterns in the waves
as space-time expands
“Being a writer or a poet was one of my childhood dreams, and I thought, I’ve got tenure now, I can be a little crazy,” Williams said.
It’s as if the Grassmannian, uncovered a century ago to formalize the mathematics of lines and planes through the origin, also happens to index phenomena in the physical world — a bizarre correspondence that Williams still can’t fully explain.
“The Grassmannian seems to be linked to a whole bunch of things that describe ‘real life,’ and I don’t have a great answer why, except to say that the Grassmannian is a very fundamental object in mathematics,” she said.
Waves to Particles
In 2016 the Harvard math department reached out to Williams and asked her if she might be interested in joining them. The overture shocked Williams for two reasons: No one else on the Harvard faculty did her kind of math, and no one else looked quite like her.
“There were no women and no combinatorialists,” she said. “It weighed on me very heavily when I was trying to make up my mind. I wasn’t sure what the atmosphere would be like.” But Williams had loved her four years at Harvard as an undergraduate — and three subsequent years as a postdoctoral researcher — and that made her more inclined to consider the university’s offer.
She traveled to Cambridge and had dinner with her prospective colleagues. The experience was reassuring, but Williams was firmly situated in California by that point. She worried about uprooting her husband and her young kids, and she also recognized that making such a high-profile move within the math world might increase public scrutiny of her and her work. Yet in the end, she felt something of an obligation to take the position, as a way of encouraging other women to pursue careers in mathematics.
“I recognized that coming to Harvard would give me a chance to make a positive impact on a department I cared a great deal about,” Williams said. “I understand that role models matter. It can be hard for people to imagine a career for themselves when they don’t see people like them with that career.”
Williams started at Harvard in the fall of 2018 and became the second woman ever to hold a tenured position in the university’s math department. (The first, Sophie Morel, spent three years at Harvard before leaving in 2012; this fall, Harvard hired two more tenured women mathematicians: Laura DeMarco and Melanie Matchett Wood.)
“There are so many hidden obstacles for female math professors at top research institutions. In some sense you have to be a warrior, but Lauren has handled things with such grace,” said Ardila.

Andrea Patiño Contreras for Quanta Magazine
At the same time Williams was moving across the country, she was deep in a new Grassmannian project. It involved a geometric object called the amplituhedron that had been proposed as the answer to one of the knottiest problems in physics.
The amplituhedron was formally described in a 2013 paper by Nima Arkani-Hamed and Jaroslav Trnka. It was meant to help physicists predict what happens when fundamental particles collide. Due to the nature of quantum interactions, such collisions are not strictly deterministic. Instead, they’re described by an amplitude, which is like a probability that the collision plays out in a given way.
The somewhat clunky prevailing method for calculating amplitudes is something called a Feynman diagram, named for its inventor, Richard Feynman. These diagrams involve vast, tedious computations that are hard to carry out with precision as particle collisions grow more complex.
The amplituhedron is a simpler way of calculating amplitudes. Given a set of particles on a collision course, you can use their properties to construct a geometric object — the amplituhedron. It embodies the particle interaction in a precise way: By calculating its volume, in effect you’re calculating the amplitude for the given collision.
“We build a shape, and the volume of the shape gives me an amplitude,” said Arkani-Hamed.
So the question is how to calculate the volume. One approach is to break the amplituhedron into pieces. This process, called a triangulation, is easy to see with an example. Imagine you have a ball and want to find its volume. One indirect way to do it is to fill it with three-dimensional triangular tiles. The total volume is the sum of the individual volumes of all the tiles used in the triangulation.
“To a first approximation, [physicists] are interested in the volume of the amplituhedron, and one way to compute volume is to break it into smaller pieces. That’s why they want to triangulate the amplituhedron,” said Williams.
Arkani-Hamed and his collaborators had defined the amplituhedron in relation to the positive Grassmannian. They demonstrated that it’s possible to change a positive Grassmannian into the amplituhedron by multiplying it by a type of matrix, effectively providing a mathematical recipe for moving points on the positive Grassmannian over to points on the amplituhedron. As a result, information about the relatively well-studied positive Grassmannian transfers to the relatively unexplored amplituhedron.

Williams and the physicist Nima Arkani-Hamed were co-organizers of a recent program at Harvard that explored connections between the positive Grassmannian and particle physics.
Over the last three years, Williams has expanded this correspondence. She demonstrated that in some cases the combinatorial properties of the positive Grassmannian — the way its points sort into cells — carries over to the amplituhedron via this transformation process. That means the cells of the positive Grassmannian can serve as the tiles needed to triangulate the amplituhedron.
So far, Williams has proved that this relationship holds for simpler versions of the amplituhedron. She’s also laid out a precise conjecture that predicts how many tiles are needed to triangulate any amplituhedron.
“We were groping around in the dark for quite a while, but [her work] on the positive Grassmannian was a shining light throughout this process,” said Arkani-Hamed.
In the fall of 2019 Williams and Arkani-Hamed were two of the co-organizers of a semester-long program at Harvard that brought together mathematicians and physicists to explore the link between the positive Grassmannian and the amplituhedron. During the event, Williams was talking with two physicists who mentioned a sequence of numbers related to triangulations of the amplituhedron.
The numbers were immediately familiar to Williams: She’d encountered them as a graduate student working on a different (and unrelated) problem on a version of the positive Grassmannian 16 years earlier. But she didn’t understand why they’d show up in this new setting.
“Whenever I had a free moment, I’d find myself wandering back to [those numbers] and wondering how to make that connection,” she said.
Eventually she did — following one more surprising pattern, with echoes of the numerical sequences that had fascinated her as a kid.
“After some months we figured out exactly what it was,” Williams said. “It was just this delightful surprise.”
Correction: December 16, 2020
An earlier version of this article incorrectly stated that the Grassmannian Gr(4,10) would be a 10-dimensional shape. It is a 24-dimensional shape.



