Mathematicians Identify Threshold at Which Shapes Give Way
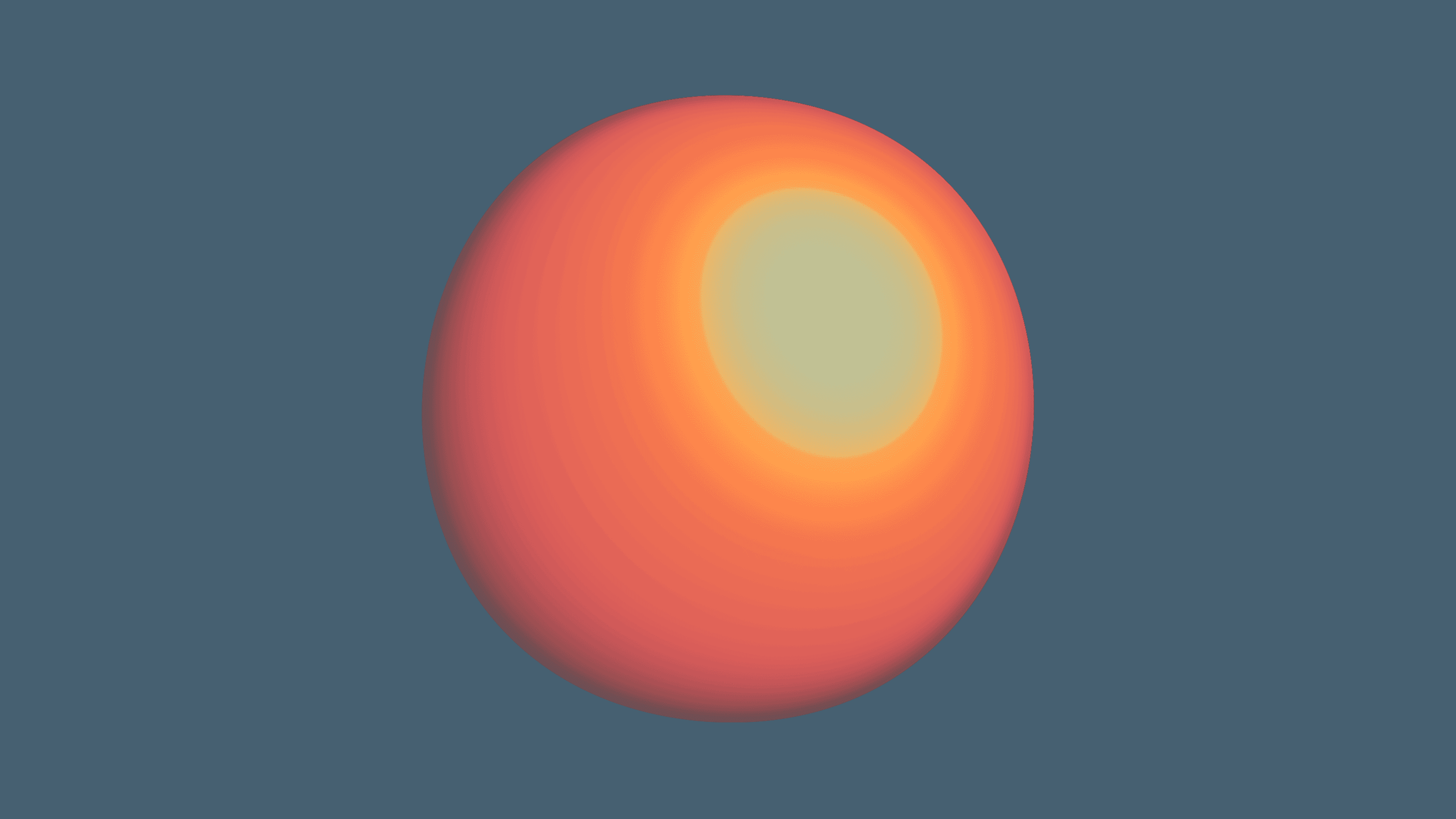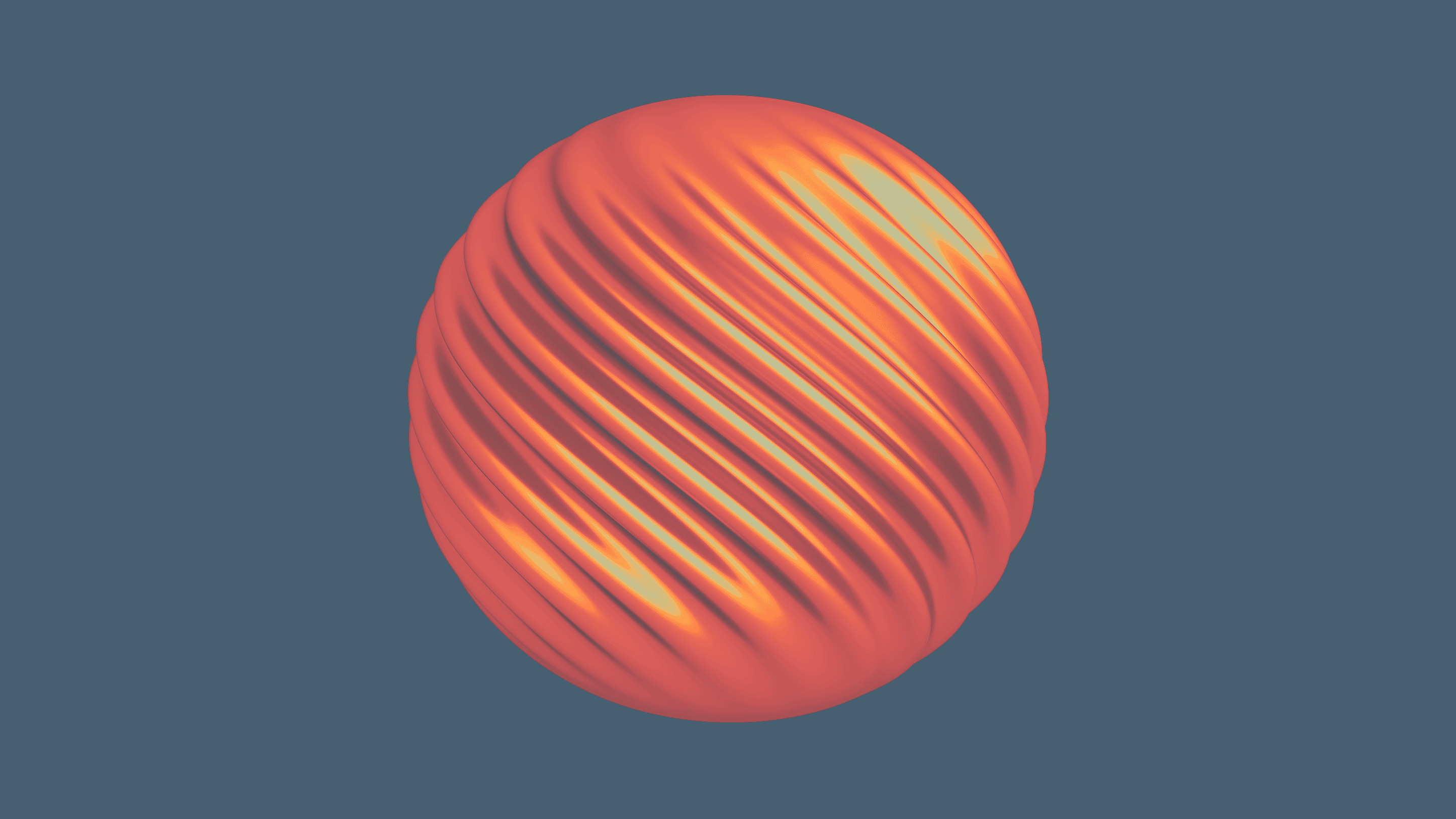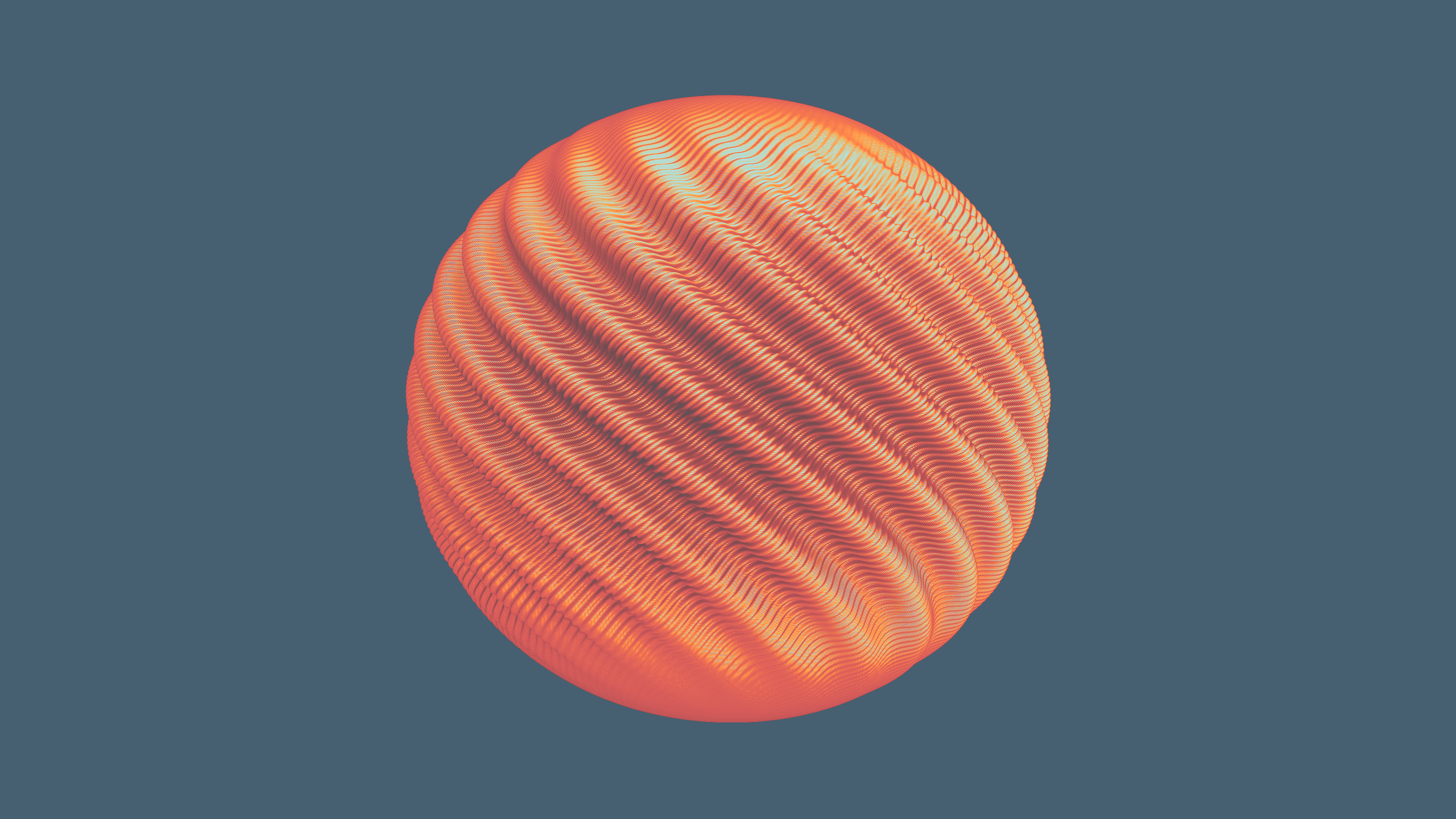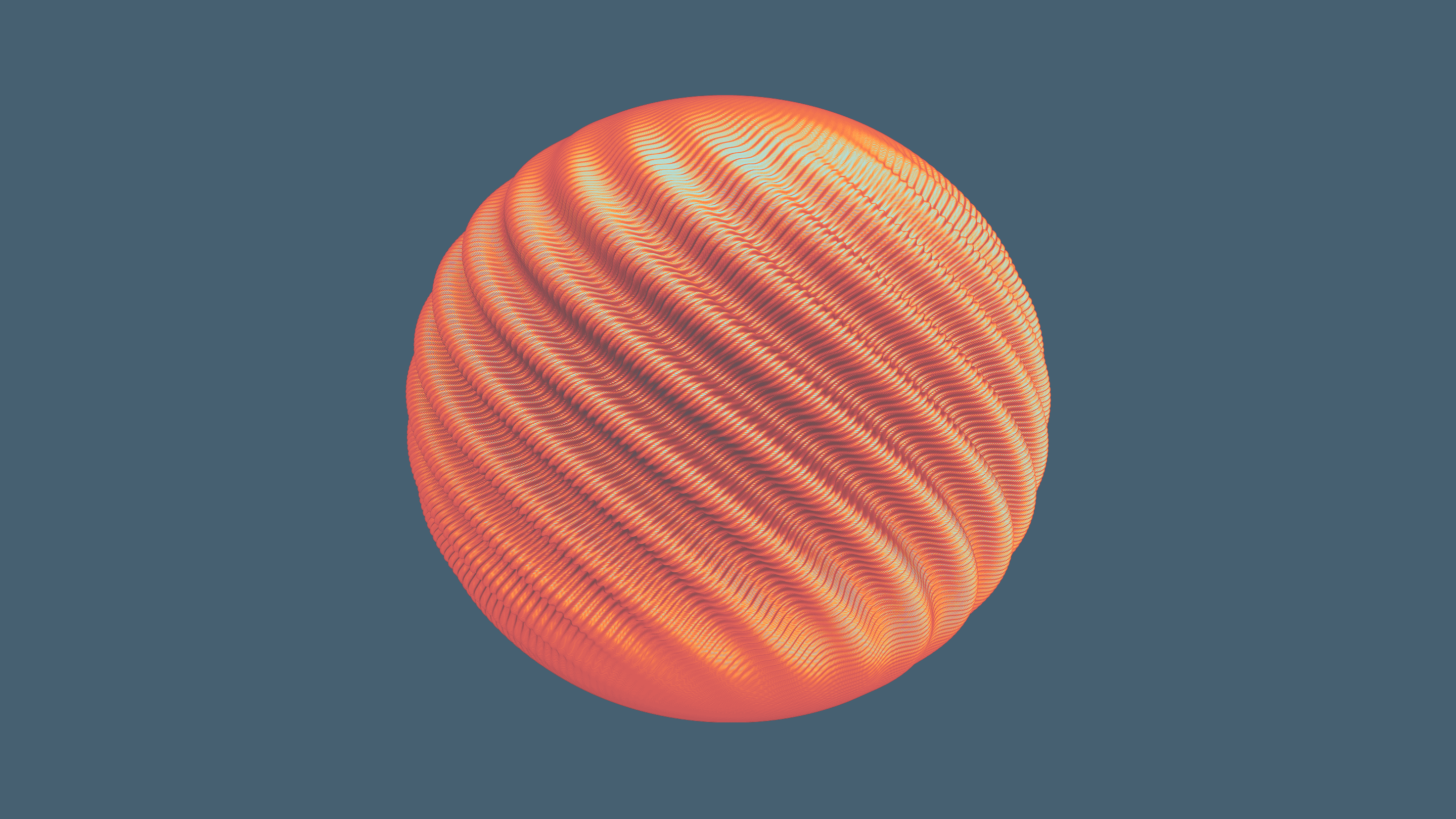
By adding infinitely many twists to the curves on a sphere, it’s possible to crush it down to a tiny ball without distorting its distances.
Hévéa Project; edited by Quanta Magazine
Introduction
In the 1950s, four decades before he won a Nobel Prize for his contributions to game theory and his story inspired the book and film “A Beautiful Mind,” the mathematician John Nash proved one of the most remarkable results in all of geometry. Among other features, it implied that you could crumple a sphere down to a ball of any size without ever creasing it. He made this possible by inventing a new type of geometric object called an “embedding,” which situates a shape inside a larger space — not unlike fitting a two-dimensional poster into a three-dimensional tube.
There are lots of ways of embedding a shape. Some preserve the shape’s natural form — like rolling the poster into a cylinder — while others crease or tear it to make it fit in different ways.
Nash’s technique unexpectedly involved adding twists to all of a shape’s curves, making its structure springy and its surface ruffled. He proved that if you added infinitely many such twists, you could crumple the sphere down to a tiny ball. The result shocked mathematicians who previously had thought that crisp folds were required to crumple the sphere in this way.
Since then, mathematicians have sought to gain a precise understanding of the limits of Nash’s pioneering techniques. He showed that you could crumple the sphere using twists, but he didn’t prove exactly how much twisting you needed, at a minimum, to get that result. Put another way, mathematicians after Nash have wanted to quantify the exact threshold between flatness and twistedness, or more generally, smoothness and roughness, at which a shape like the sphere begins to crumple.
And in a pair of recent papers they have, at least for a sphere sitting in a higher-dimensional space. In a paper posted in September 2018 and published in March 2020, Camillo De Lellis of the Institute for Advanced Study in Princeton, New Jersey, and Dominik Inauen of Leipzig University identified an exact threshold for one particular shape. Later work in October 2020, by Inauen and Wentao Cao, now of Capital Normal University in Beijing, proved that the threshold applied to all shapes of a certain general type.
The two papers significantly improve mathematicians’ understanding of Nash embeddings. They also establish an unlikely connection between embeddings and fluid flows.
“We discovered these kinds of amazing points of contact between the two problems,” De Lellis said.
Tumbling rivers might seem only vaguely related to crumpling shapes, but mathematicians discovered in 2009 that they could in fact be studied using the same techniques. Three years ago mathematicians, including De Lellis, used Nash’s ideas to understand the point at which a flow becomes turbulent. Their work reimagined a fluid as made up of twisty flows — and they proved that if you added just enough twists to those flows, the fluid suddenly took on a key characteristic of turbulence.
The new work on embeddings builds on a crucial lesson from that earlier work on turbulence, suggesting that mathematicians now have a general framework for identifying sharp transition points in a range of mathematical settings.
Maintaining Length
Mathematicians today think of shapes, like the sphere, as having their own intrinsic geometric properties: A sphere is a sphere regardless of where you find it.
But you can take an abstract shape and embed it inside a larger geometric space. As you embed it, you might want to preserve everything about it. Or you might require that only some properties are held constant — for example, that the lengths of curves on its surface remain the same. Such embeddings are called “isometric.”
Isometric embeddings maintain lengths but can still change a shape in significant ways. Start, for example, with a piece of graph paper with its grid of perpendicular lines. Fold it as many times as you like. This process can be thought of as an isometric embedding. The resulting shape will look nothing like the smooth plane you started with, but the lengths of the grid lines won’t have changed.

Nash’s twisty embeddings retain a surprising degree of smoothness, even as they make it possible to radically alter a surface.
Hévéa Project; edited by Quanta Magazine
For a long time, mathematicians thought that sharp folds were the only way to have both characteristics at once: a crumpled shape with lengths preserved.
“If you allow corners to happen, then the problem is much easier,” said Tristan Buckmaster of Princeton University.
But in 1954, John Nash identified a remarkably different type of isometric embedding that managed the same trick. It used helical twists rather than sharp creases and corners.
To get an intuition for Nash’s idea, start again with the smooth surface of a sphere. That surface is composed of many curves. Take each one and twist it into a spring-shaped helix. After all the curves are reformulated like this, it’s possible to compress the sphere. However, such a process seems to violate the rules of an isometric embedding — after all, a curvy path between two points is always longer than a straight one.
But, remarkably, Nash showed there is a rigorous way of maintaining lengths even as you refabricate curves out of twists. First, shrink the sphere evenly, like a deflating balloon. Then add increasingly tight spirals to every curve. By adding infinitely many such twists, you can eventually restore every curve to its original length, even when the original sphere has been crumpled.
Nash’s work called for further exploration. Technically, his results implied that you could crumple a sphere only if it existed in four spatial dimensions. But in 1955, Nicolaas Kuiper extended Nash’s work so that it applied to the standard, three-dimensional sphere. From there, mathematicians wanted to understand the exact point at which, if you twisted the curves of a sphere enough, you could make it collapse.
Smooth Smoothness
Folded and twisted shapes differ from each other in a key way. To understand how, you need to know what mathematicians mean when they say that something is “smooth.”
A classic example of smoothness is the rising and falling shape of a sine wave, one of the most common curves in mathematics. A mathematical way of conveying this smoothness is to say that you can calculate the “derivative” of the wave at every point. The derivative measures the slope of the curve at a point, meaning the degree to which it’s inclining or declining.
In fact, you can do more than calculate the derivative of a sine wave. You can also calculate the derivative of the derivative or, the “second” derivative, which captures the rate of change of the slope. This quantity makes it possible to determine the curvature of the curve — whether the curve is convex or concave near a certain point, and to what degree.
And there’s no reason to stop there. You can also calculate the derivative of the derivative of the derivative (the “third” derivative), and so on. This infinite tower of derivatives is what makes a sine wave perfectly smooth in an exact mathematical sense. But when you fold a sine wave, the derivative tower collapses. Along a crease the slope of the curve is not well defined, meaning it is impossible to calculate even a first derivative.
Before Nash, mathematicians thought losing the first derivative was a necessary consequence of crumpling the sphere while maintaining lengths. Put another way, they thought crumpling and smoothness were incompatible.
But Nash showed otherwise.
Using his method, it’s possible to crumple the sphere without ever folding any curves. All Nash needed was smooth twists. However, the infinitude of tiny twists required by his embedding makes the second-derivative notion of curvature nonsensical, just as folding destroys the first-derivative notion of slope. It’s never clear anywhere on one of Nash’s surfaces whether a curve is concave or convex. Each twist that is added makes the shape more and more corrugated and grooved, and an infinitely grooved surface becomes rough.
“If you were a skier on the surface, then everywhere, you would feel bumps,” said Vincent Borrelli of the University of Lyon, who in 2012 worked with collaborators to create the first accurate visualizations of Nash embeddings.
The new work explains the exact extent to which a surface can hold on to derivatives even as its structure gives way.
Finding the Boundary
Mathematicians have precise notation for describing the number of derivatives that can be calculated on a curve.
An embedding that folds a shape is called C0. The C stands for continuity and the superscript zero means the curves on the embedded surface have no derivatives, not even a first. There are also embeddings with fractional superscripts, like C0,1/2, which still crease curves, but less sharply. Then there are Nash’s C1 embeddings, which crush curves only by applying smooth twists, thereby retaining a first derivative.
Samuel Velasco / Quanta Magazine
Prior to Nash’s work, mathematicians had mainly been concerned with isometric embeddings of a certain standard smoothness, C2 and above. These C2 embeddings might twist or bend curves, but only gently. In 1916, the influential mathematician Hermann Weyl conjectured that you could not change the shape of the sphere using such gentle bends without also destroying distances. In the 1940s, mathematicians solved the Weyl problem, proving that C2 isometric embeddings could not crumple the sphere.
In the 1960s, Yurii Borisov discovered that a C1,1/13 embedding could still crumple the sphere, while a C1,2/3 embedding could not. Thus, somewhere between Nash’s C1 embeddings and gently bending C2 embeddings, crumpling becomes possible. But for decades after Borisov’s work, mathematicians did not get closer to finding an exact boundary — if one even existed.
“Some fundamental new insight [was] needed,” said Inauen.
While mathematicians could not make progress, they did find other applications for Nash’s ideas. In the 1970s Mikhael Gromov reframed them as a general tool called “convex integration,” which allows mathematicians to construct solutions to many problems by using twisty substructures. In one example, which ended up being relevant to the new work, convex integration made it possible to think of a flowing fluid as made of many twisted sub-flows.
Decades later, in 2016, Gromov reviewed the incremental progress on embeddings of the sphere and conjectured that a threshold in fact existed, at C1,1/2. The problem was that at that threshold, existing methods broke down.
“We were stuck,” Inauen said.
To make progress, mathematicians needed a new way to distinguish between embeddings of different smoothness. De Lellis and Inauen found it with inspiration from work on an entirely different phenomenon: turbulence.
Disappearing Energy
All materials that come in contact have friction, and we think of that friction as being responsible for slowing things down. But for years, physicists have observed a remarkable property of turbulent flows: They slow even when internal friction, or viscosity, is absent.
In 1949, Lars Onsager proposed an explanation. He conjectured that frictionless dissipation was related to the extreme roughness (or lack of smoothness) of a turbulent flow: When a flow becomes rough enough, it starts to exhaust itself.
In 2018 Philip Isett proved Onsager’s conjecture, with Buckmaster, De Lellis, László Székelyhidi and Vlad Vicol contributing in a separate work. They used convex integration to construct roiling flows as rough as C0, up to C0,1/3 (so, substantially rougher than C1). These flows violated a formal rule called the conservation of kinetic energy and slowed themselves down, purely by way of their roughness.
“The energy gets sent off to infinitely small scales, zero length scales in finite time, and then disappears,” said Buckmaster.
Earlier work from 1994 had established that frictionless flows smoother than C0,1/3 (with a larger superscript) did conserve energy. Together, the two results pinned down a sharp threshold between turbulent, energy-dissipating flows and nonturbulent, energy-conserving ones.
The Onsager work also gave a kind of proof of principle that sharp thresholds could be revealed with convex integration. The key seemed to be in finding the right rule that held on one side of the threshold and failed on the other. De Lellis and Inauen took notice.
“We thought, well, maybe you have an additional law, like the [kinetic energy law],” said Inauen. “Isometric embeddings above a certain threshold satisfy it, and below that threshold they might violate it.”
After that, they just had to go and find the law.
Maintaining Acceleration
The rule that they ended up investigating has to do with the value of the acceleration of curves on a surface. To understand it, imagine, first, a person skating along a spherical shape before it’s been embedded. They’ll feel a sense of acceleration (or deceleration) as they swing into turns and coast up and down hills. Their trajectory forms a curve.
Now imagine the skater racing along the same shape after it’s been embedded. For smooth-enough isometric embeddings, which don’t crumple the sphere or deform it in any way, the skater should feel the same forces along the embedded curve. After recognizing this, De Lellis and Inauen then needed to prove it: that embeddings smoother than C1,1/2 conserve acceleration.
In 2018 they applied this perspective to a particular shape called the polar cap, which is the cutoff top of the sphere. They studied embeddings of the cap that keep the base of the cap fixed in place. Since the base of the cap is fixed, a curve traveling around it can change acceleration only if the shape of the cap above it is changed, for example, by being buckled inward or outward. They proved that embeddings smoother than C1,1/2 — even Nash embeddings — don’t alter acceleration and therefore don’t buckle the cap.
“It provides a very nice geometric picture,” Inauen said.
On the other hand, they used convex integration to construct embeddings of the cap rougher than C1,1/2. These Nash embeddings twist curves so much that they lose their notion of acceleration, which is a second derivative quantity. But the acceleration of the curve around the base remains sensible, since it is fixed in place. They showed that embeddings below the threshold could alter the acceleration of this curve, implying that they also buckle the cap (because if the cap doesn’t buckle, acceleration remains constant; and if acceleration isn’t constant, it means the cap must have buckled).
Two years later, Inauen and Cao extended the prior paper and proved that Gromov’s predicted value of C1,1/2 was indeed a threshold that applied to any shape, or “manifold,” with a fixed boundary. Above it, shapes don’t buckle, below it they do. “We generalized the result,” said Cao.
A key limitation of Cao and Inauen’s paper is that it requires embedding a shape into eight-dimensional space, rather than the three-dimensional space Gromov had in mind. With extra dimensions, the mathematicians gained more room to add twists, which made the problem easier.
While the results don’t completely answer Gromov’s conjecture, they provide the best insight yet into the relationship between smoothness and crumpling. “They give you a first instance in which we really see this dichotomy,” said De Lellis.
From here, mathematicians have a number of paths to follow. For one, they’d like to solve the conjecture in three dimensions. At the same time, they’d like to better understand the powers of convex integration.
This fall the Institute for Advanced Study will begin hosting a full-year program on the topic. It will bring together researchers from a wide range of fields with the goal of better understanding the ideas Nash invented. As Gromov pointed out in his 2016 paper, Nash’s twisty shapes were not simply part of geometry. As has now become clear, they paved the road to an entirely “new land” of mathematics, where sharp thresholds emerge in many places.



