The Trouble With Turbulence

Qais Sarhan for Quanta Magazine
Introduction
Fluids should be easy. They’re ordinary, classical things — water, air currents, maple syrup — described by physical laws first written down nearly two centuries ago. And yet when a tornado rips open the roof of the table-tennis factory, just try to predict where all the pingpong balls are going to land.
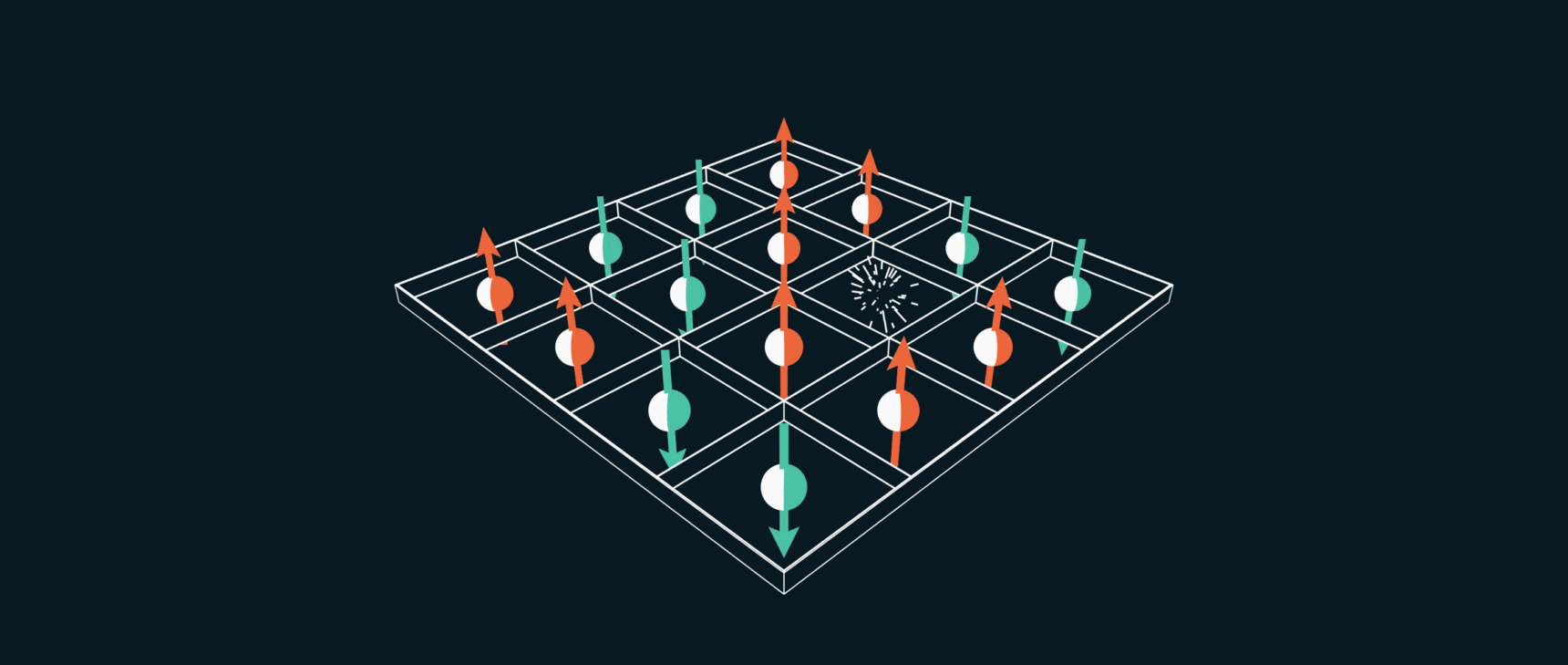
This is hard because of turbulence, a problem that gives physicists and mathematicians more trouble than you might think. On the physical side, turbulence happens when a smooth fluid flow starts to split into smaller eddies and vortices. These swirls then break into smaller swirls, with those swirls begetting ever-smaller whorls, an unpredictable cascade that dissipates the energy from the original smooth stream. These whorls all affect one another, making it impossible to precisely predict what is going to happen to any particular particle in the fluid you’re measuring. On the large scale, energy dissipates gradually and with a semblance of order. On the small scale, chaos abounds.
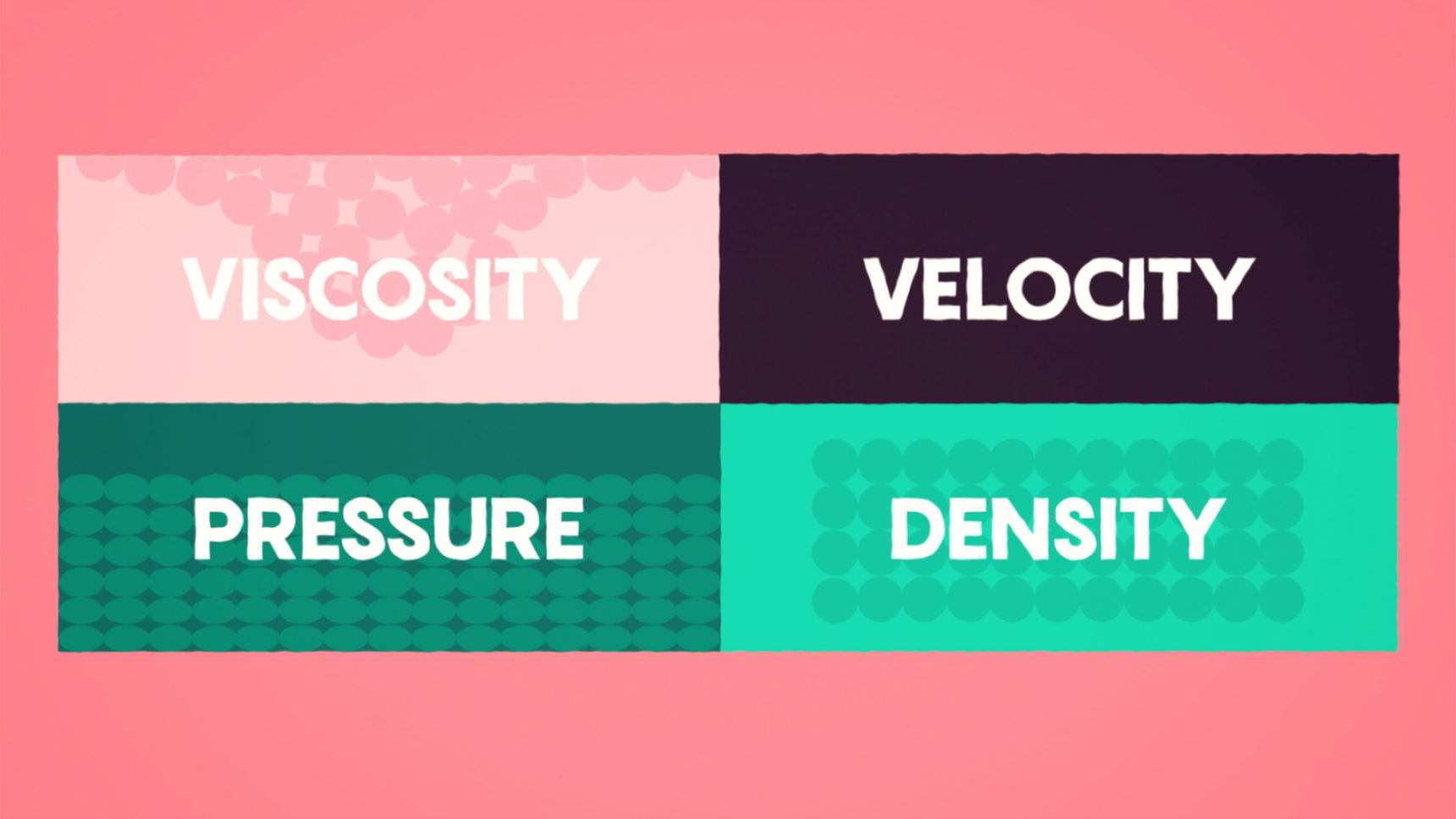
The mathematics of turbulence would at first appear to present a simpler case. The Navier-Stokes equations have been used to describe fluid flows since the early 19th century. They take into account properties of the fluid such as its density and viscosity, along with any forces acting on it. And for all practical purposes — and so long as we don’t ask the equations to predict the exact motions of all the eddies in a turbulent fluid — we know that they work. But “for all practical purposes” is not the same as a proof.
What is there to prove? For one thing, that the Navier-Stokes equations will always behave nicely. That is, given any initial state of the fluid, mathematicians want to be able to prove that the equations will never lead to a nonsensical result. You might imagine a scenario in which all those swirls and whorls conspire to concentrate all their energy at a particular point in the fluid, and in doing so accelerate the flow at that point to infinite velocity. That’s unlikely, but mathematically possible (so far as we know). The proof that such a scenario is impossible — or the opposite, a demonstration of how it might happen — is so coveted that it stands as one of the $1 million Millennium Prize Problems.
This fourth episode from season two of Quanta’s In Theory video series covers the trouble with turbulence. It follows videos on universality, quantum gravity and emergence — like turbulence, never easy, but frequently fascinating.