A Grand Theory of Wrinkles

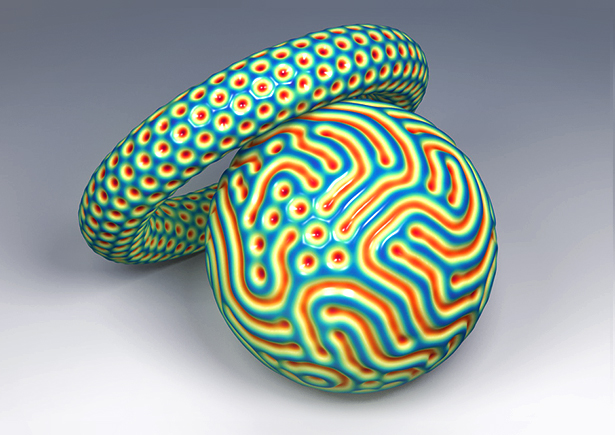
Pedro Reis, an engineer at the Massachusetts Institute of Technology, had long been interested in how things wrinkle. For example, a dimpled surface like that of a golf ball offers less air resistance than a smooth sphere. If a flying object could dimple or wrinkle on command, Reis thought, it could alter its own aerodynamics midflight.

Reis constructed silicone test spheres and sucked air out of them. He noticed that under pressure, some of the spheres formed the dimples he wanted, but some formed squiggly, labyrinthine patterns instead. Some had both dimples and labyrinths. When a member of his group shared the puzzle with a group of mathematicians at MIT led by Jörn Dunkel, they were intrigued: The wrinkling patterns resembled the stripes and swirls that appear when you heat a thin layer of oil, a phenomenon called Rayleigh–Bénard convection. Those phenomena had simplified, calculable equations — so why shouldn’t wrinkles have a simplified equation too?

Earlier researchers had worked backwards from specific wrinkling effects to create simulations that worked in single cases, but nobody had simplified the full elastic equations from the ground up to describe all wrinkling behavior — there was not yet a universal theory of wrinkles. It had been unclear which of the many variables were important.

Reis and the mathematicians started to go over the detailed body of experiments that Reis’s group had assembled. When they examined the data from the rubbery spheres, the researchers found that just two factors controlled the formation of patterns: the curvature of a lower layer as compared to the thickness of the wrinkling layer on top, and the stress applied to that wrinkling layer. Films over less-curved surfaces would quickly transition to hybrid or labyrinth forms when put under stress. Setups that were more curved with a thicker layer on top would form a hexagonal layout of dimples and then, if stressed enough (as when Reis pulled air from inside the spheres), would eventually go labyrinthine as well. Releasing the stress would transition the surface back. “What’s interesting is not just that these two parameters are important, but that all the other parameters are not important,” said Norbert Stoop, one of the MIT mathematicians. The researchers found that the stiffness of the wrinkling layer, for instance, has no effect on the outcome. “Our theory you could basically apply to the surface of the moon or Mars, or the surface of a grape.”

“It’s one of those things, I promise you, you kick yourself that you didn’t do it first,” said Christian Santangelo, a physicist and materials scientist at University of Massachusetts, Amherst. “It didn’t occur to anybody, I think, that you can write something that simple and have it work.”

Reis’s experiments were macroscopic, the size of ping-pong balls, but the group found that microscopic spheres transitioned in line with the theory too: Another lab had recorded identical patterns on tiny hemispheres of a silicone polymer by chemically increasing the stress on a thin, oxide coating layer.

As the group pursued their final, simplified equation, they found that their initial hunch was right. The equation closely resembled one in fluid dynamics that describes the convection currents that form in hot oil. In its generalized form, the description was part of a larger class of systems where a regular arrangement suddenly becomes unstable and “breaks” its symmetry as a variable gets tweaked — for example, when ice, which has a regular crystalline structure, warms and melts into water. General symmetry-breaking theories were developed in the 1970s, but they rarely find straightforward counterparts in nonfluid systems, Stoop said.

And the work may help others discover simple descriptions of other complicated elastic systems, said Santangelo. With the aid of computers, researchers can construct complicated models that faithfully describe the phenomenon at hand, but they don’t provide much insight into the underlying physics. “There are these huge programs where they basically just put everything and the kitchen sink into it, and then yeah, sure, it works,” he said. “But the idea that somehow certain classes of phenomenon are simpler than that, that they just don’t need as much description, is pretty useful.”

The new model could help researchers understand a number of important wrinkled systems found in nature, including the undulating surfaces of planets and the dimples of the small intestine. Anything curved and wrinkled could have these basic forms at its core, even if they’re obscured by more complicated interactions.

For the collaborators themselves, the journey is far from over. The theoretical equations aren’t constrained to spheres, and they have plenty to say about how wrinkles will form on more complicated shapes where the curvature changes — experiments that Reis’s group has yet to try.

“What’s beautiful about this work is the collaboration between experimentalists and theorists,” said Reis. “We challenged them with results we didn’t understand, and they went somewhere new. Now the theory is challenging us with new questions that we’re testing out in simulations and new experiments.”

This article was reprinted on Wired.com and in Spanish at Investigacionyciencia.es.